Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-24T00:02:22.280Z Has data issue: false hasContentIssue false
  • English
  • Français

On symmetric Siegel domains

Published online by Cambridge University Press:  22 January 2016

Masaru Takeuchi
Masaru Takeuchi*
Affiliation:
Department of Mathematics, Osaka University
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let V be a convex cone in a real vector space X, F: Y × Y → Xc a V-positive hermitian map on a complex vector space Y, and

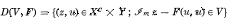
the Siegel domain associated to V and F. D(V,F) is said to be symmetric, if for each point p ∈ D(V, F) there exists an involutive holomorphic automorphism σp of D(V,F) such that the fixed point set of σp consists of only the point p. Satake [6] showed that the symmetric Siegel domain D(V, F) is characterized by the following three conditions (i), (ii) and (iii).

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 59 , December 1975 , pp. 9 - 44
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1975

References

[1] Kaneyuki, S., On the automorphism groups of homogeneous bounded domains, J. Fac. Sci. Univ. Tokyo 14 (1967), 89–130.Google Scholar
[2] Kaup, W., Matsushima, Y. and Ochiai, T., On automorphisms and equivalences of generalized Siegel domains, Amer. J. Math. 92 (1970), 475–497.CrossRefGoogle Scholar
[3] Nakajima, K., Some studies on Siegel domains, to appear.Google Scholar
[4] Ozeki, H. and Takeuchi, M., On some types of isoparametric hypersurfaces in spheres I, to appear.Google Scholar
[5] Pjateckii-Sapiro, I. I., Géométrie des domaines classiques et théorie des fonctions automorphes, Dunod, Paris, 1966.Google Scholar
[6] Satake, I., Infinitesimal automorphisms of symmetric Siegel domains, to appear.Google Scholar
[7] Takeuchi, M., Homogeneous Siegel domains, Pub. Study Group Geometry, No. 7, Tokyo, 1973.Google Scholar
[8] Tsuji, T., Siegel domains over self-dual cones and their automorphisms, to appear.Google Scholar
[9] Vinberg, È. B., Homogeneous cones, Soviet Math. Dokl. 1 (1960), 787–790.Google Scholar
[10] Vinberg, È. B., The theory of convex homogeneous cones, Trans. Moscow Math. Soc. (1963), 340–403.Google Scholar
[11] Vinberg, È. B., The structure of the group of automorphisms of a homogeneous convex cone, Trans. Moscow Math. Soc. (1965), 63–93.Google Scholar
[12] Vinberg, È. B., Gindikin, S. G. and Pjateckii-Šapiro, I. I., Classification and canonical realization of complex bounded homogeneous domains, Trans. Moscow Math. Soc. (1963), 404–437.Google Scholar