Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-23T16:10:37.915Z Has data issue: false hasContentIssue false
  • English
  • Français

On Maximally Central Algebras

Published online by Cambridge University Press:  22 January 2016

Gorô Azumaya
Gorô Azumaya*
Affiliation:
Mathematical Institute, Nagoya University
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let A be a primary algebra with unit element over a field K and Z its center. Let Ā be the simple residue class algebra of A modulo its radical. Then it is known, and can readily be seen, that there holds the inequality where t is the rank of A over its center. We call A maximally central if in particular 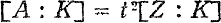i.e. if the rank [Z: K] takes its maximum value. Further, an algebra which is a direct sum of those primary algebras will be called maximally central too. The notion was introduced in Azumaya-Nakayama [5], as a by-product of the study of absolutely uni-serial algebras.

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 2 , February 1951 , pp. 119 - 150
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1951

References

[1] Albert, A. A., Structure of Algebras, New York (1939).CrossRefGoogle Scholar
[2] Artin, E., Nesbitt, C. J. and Thrall, R. M., Rings with minimum condition, Michigan Press (1944).Google Scholar
[3] Azumaya, G., On generalized semi-primary rings and Krull-Remak-Schmidt’s theorem, Jap. Journ. Math. 19 (1948).Google Scholar
[4] Azumaya, G., Galois theory for uni-serial rings, Journ. Math. Soc. Jap. 1 (1949).Google Scholar
[5] Azumaya, G. and Nakayama, T., On absolutely uni-serial algebras, Jap. Journ. Math. 19 (1948).Google Scholar
[6] Chevalley, C., On the theory of local rings, Ann. Math; 44 (1943).Google Scholar
[7] Cohen, I. S., On the structure and ideal theory of complete local rings, Trans. Amer. Math. Soc. 59, (1946).Google Scholar
[8] Deuring, M., Algebren, Ergeb. Math. 4 (1935).CrossRefGoogle Scholar
[9] Jacobson, N., The radical and semi-simplicity for arbitrary rings, Amer. Journ. Math. 67 (1945).Google Scholar
[10] Icev, A. Ma, On the representation of an algebra as a direct sum of the radical and a semi-simple algebra, C. R. URSS. 36 (1942).Google Scholar
[11] Nagata, M., On the structure of complete local rings, Nagoya Math. Journ. 1 (1950).Google Scholar
[12] Nakayama, T., Divisionsalgebren über diskret bewerteten perf’ekten Korpern, Journ. rein, angew. Math. 178 (1937).Google Scholar
[13] Witt, E., Schiefkörper über diskret bewerteten Korpern, Journ. rein, angew. Math. 176 (1936).Google Scholar