Published online by Cambridge University Press: 22 January 2016
Let M be an n-dimensional hypersurface immersed in the (n + 1)-dimensional unit sphere Sn+1 with the standard metric by an immersion f. We denote by A the second fundamental form of the immersion / which is considered as a symmetric linear transformation of each tangent space TXM, i.e. for an arbitrary point x of M and the unit normal vector field ξ defined in a neighborhood of x, A is given by 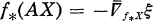 where
where 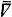 is the covariant differentiation in Sn+i and
is the covariant differentiation in Sn+i and  Thus, A depends on the orientation of the unit normal vector field ξ and, in general, it is locally defined on M.
Thus, A depends on the orientation of the unit normal vector field ξ and, in general, it is locally defined on M.