Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-20T04:30:38.493Z Has data issue: false hasContentIssue false
  • English
  • Français

On boundaries of Schottky spaces

Published online by Cambridge University Press:  22 January 2016

Hiroki Sato
Hiroki Sato*
Affiliation:
Department of Mathematics, Shizuoka University
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let S be a compact Riemann surface and let Sn be the surface obtained from S in the course of a pinching deformation. We denote by Γn the quasi-Fuchsian group representing Sn in the Teichmüller space T(Γ), where Γ is a Fuchsian group with U/Γ = S (U: the upper half plane). Then in the previous paper [7] we showed that the limit of the sequence of Γn is a cusp on the boundary ∂T(Γ). In this paper we will consider the case of Schottky space 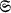. Let Gn be a Schottky group with Ω(Gn)/Gn = Sn. Then the purpose of this paper is to show what the limit of Gn is.

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 62 , September 1976 , pp. 97 - 124
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1976

References

[1] Abikoff, W., Two theorems on totally degenerate Kleinian groups, (to appear).Google Scholar
[2] Bers, L., On boundaries of Teichmüller spaces and on Kleinian groups: I, Ann. of Math. 91 (1970), 570–600.CrossRefGoogle Scholar
[3] Chuchrow, V., On Schottky groups with applications to Kleinian groups, Ann. of Math. 88 (1968), 47–61.CrossRefGoogle Scholar
[4] Kra, I,. On spaces of Kleinian groups, Comment. Math. Helv. 47 (1972), 53–69.CrossRefGoogle Scholar
[5] Marden, A., Schottky groups and circles, Contributions to Analysis: A collected papers dedicated to Lipmann Bers, (1974), 273–278.Google Scholar
[6] Maskit, B., On boundaries of Teichmüller spaces and on Kleinian groups: II, Ann. of Math. 91 (1970), 607–639.CrossRefGoogle Scholar
[7] Sato, H., Cusps on boundaries of Teichmüller spaces, Nagoya Math. J. 60, (to appear).Google Scholar