Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ono, Takashi
1983.
On certain integrals over spheres.
Proceedings of the Japan Academy, Series A, Mathematical Sciences,
Vol. 59,
Issue. 10,
Ono, Takashi
1984.
On deformations of Hopf maps and hypergeometric series.
Nagoya Mathematical Journal,
Vol. 95,
Issue. ,
p.
63.


 and the norm
and the norm 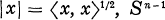 be the unit sphere {
be the unit sphere {