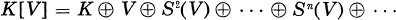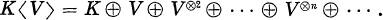Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Teranishi, Yasuo
1991.
Young diagrammatic methods in non-commutative invariant theory.
Nagoya Mathematical Journal,
Vol. 121,
Issue. ,
p.
15.
Kharchenko, V.K.
2000.
Vol. 2,
Issue. ,
p.
359.
Göbel, Manfred
and
Kredel, Heinz
2002.
Reduction of Permutation-Invariant Polynomials.
Information and Computation,
Vol. 175,
Issue. 2,
p.
158.
Schröer, André
2004.
Besteuerung verbundener Unternehmen in föderalen Strukturen.
p.
9.
Benaych-Georges, Florent
and
Nechita, Ion
2009.
A permutation model for free random variables and its classical analogue.
Pacific Journal of Mathematics,
Vol. 242,
Issue. 1,
p.
33.
Lehner, Franz
2011.
A noncrossing basis for noncommutative invariants of SL(2,C).
Journal of Combinatorial Theory, Series A,
Vol. 118,
Issue. 1,
p.
257.
Muminov, Kabiljon Kadyrovich
and
Juraboev, Saidakhbor Solijonovich
2023.
Equivalence of paths with respect to the action of the group Sp (n).
Vol. 2997,
Issue. ,
p.
020040.