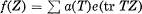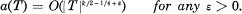Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Fomenko, O. M.
1987.
Fourier coefficients of Siegel cusp forms of genus n.
Journal of Soviet Mathematics,
Vol. 38,
Issue. 4,
p.
2148.
Kohnen, Winfried
1992.
Estimates for fourier coefficients of siegel cusp forms of degree two, II.
Nagoya Mathematical Journal,
Vol. 128,
Issue. ,
p.
171.
Duke, W.
Howe, R.
and
Li, J.-S.
1992.
Estimating Hecke eigenvalues of Siegel modular forms.
Duke Mathematical Journal,
Vol. 67,
Issue. 1,
Böcherer, Siegfried
and
Kohnen, Winfried
1993.
Estimates for Fourier coefficients of Siegel cusp forms.
Mathematische Annalen,
Vol. 297,
Issue. 1,
p.
499.
Wang, Juping
1997.
Some new results about Jacobi forms.
Chinese Science Bulletin,
Vol. 42,
Issue. 3,
p.
181.
Kowalski, Emmanuel
Saha, Abhishek
and
Tsimerman, Jacob
2012.
Local spectral equidistribution for Siegel modular forms and applications.
Compositio Mathematica,
Vol. 148,
Issue. 2,
p.
335.
Das, Soumya
and
Sengupta, Jyoti
2012.
Nonvanishing of Siegel Poincaré series.
Mathematische Zeitschrift,
Vol. 272,
Issue. 3-4,
p.
869.
Tóth, Árpád
2013.
Symplectic Kloosterman sums.
Studia Scientiarum Mathematicarum Hungarica,
Vol. 50,
Issue. 2,
p.
143.
Chida, Masataka
Katsurada, Hidenori
and
Matsumoto, Kohji
2014.
On Fourier coefficients of Siegel modular forms of degree two with respect to congruence subgroups.
Abhandlungen aus dem Mathematischen Seminar der Universität Hamburg,
Vol. 84,
Issue. 1,
p.
31.
Dickson, Martin
2015.
Local spectral equidistribution for degree two Siegel modular forms in level and weight aspects.
International Journal of Number Theory,
Vol. 11,
Issue. 02,
p.
341.
Waibel, Fabian
2019.
MOMENTS OF SPINOR L-FUNCTIONS AND SYMPLECTIC KLOOSTERMAN SUMS.
The Quarterly Journal of Mathematics,
Blomer, Valentin
2021.
Relative Trace Formulas.
p.
51.
Blomer, Valentin
and
Corbett, Andrew
2022.
A symplectic restriction problem.
Mathematische Annalen,
Vol. 382,
Issue. 3-4,
p.
1323.
Man, Siu Hang
2022.
Symplectic Kloosterman sums and Poincaré series.
The Ramanujan Journal,
Vol. 57,
Issue. 2,
p.
707.
Wei, Zhining
2023.
Linear Relations of Siegel Poincaré Series and Non-vanishing of the Central Values of Spinor L-functions.
Journal de théorie des nombres de Bordeaux,
Vol. 34,
Issue. 3,
p.
755.
Ikeda, Tamotsu
and
Katsurada, Hidenori
2023.
Estimates for the Fourier coefficients of the Duke–Imamoglu–Ikeda lift.
Forum Mathematicum,
Vol. 35,
Issue. 4,
p.
975.
Das, Soumya
and
Krishna, Hariram
2024.
Bounds for the Bergman Kernel and the Sup-Norm of Holomorphic Siegel Cusp Forms.
International Mathematics Research Notices,
Vol. 2024,
Issue. 7,
p.
6140.