Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-03T05:23:09.115Z Has data issue: false hasContentIssue false
  • English
  • Français

FINITENESS OF LOG MINIMAL MODELS AND NEF CURVES ON $3$-FOLDS IN CHARACTERISTIC 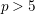$p>5$

Part of: Birational geometry

Published online by Cambridge University Press:  10 September 2018

OMPROKASH DAS Open the ORCID record for OMPROKASH DAS [Opens in a new window]
OMPROKASH DAS*
Affiliation:
Department of Mathematics, University of California, Los Angeles, 520 Portola Plaza, Math Sciences Building 6363, USA email [email protected], [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this article, we prove a finiteness result on the number of log minimal models for 3-folds in 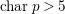$\operatorname{char}p>5$. We then use this result to prove a version of Batyrev’s conjecture on the structure of nef cone of curves on 3-folds in characteristic 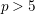$p>5$. We also give a proof of the same conjecture in full generality in characteristic 0. We further verify that the duality of movable curves and pseudo-effective divisors hold in arbitrary characteristic. We then give a criterion for the pseudo-effectiveness of the canonical divisor 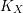$K_{X}$ of a smooth projective variety in arbitrary characteristic in terms of the existence of a family of rational curves on 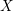$X$.

MSC classification

Primary: 14E30: Minimal model program (Mori theory, extremal rays) 14E05: Rational and birational maps 14E99: None of the above, but in this section
Type
Article
Information
Nagoya Mathematical Journal , Volume 239 , September 2020 , pp. 76 - 109
Check for updates
Copyright
© 2018 Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Araujo, C., The cone of pseudo-effective divisors of log varieties after Batyrev, Math. Z. 264(1) (2010), 179–193.10.1007/s00209-008-0457-8Google Scholar
Batyrev, V. V., “The cone of effective divisors of threefolds”, in Proceedings of the International Conference on Algebra, Part 3 (Novosibirsk, 1989), Contemporary Mathematics 131, American Mathematical Society, Providence, RI, 1992, 337–352.Google Scholar
Birkar, C., Existence of flips and minimal models for 3-folds in char p, Ann. Sci. Éc. Norm. Supér. (4) 49(1) (2016), 169–212.10.24033/asens.2279Google Scholar
Birkar, C., Singularities of linear systems and boundedness of Fano varieties, preprint, 2016, arXiv:1609.05543.Google Scholar
Birkar, C., Cascini, P., Hacon, C. D. and McKernan, J., Existence of minimal models for varieties of log general type, J. Amer. Math. Soc. 23(2) (2010), 405–468.10.1090/S0894-0347-09-00649-3Google Scholar
Birkar, C. and Waldron, J., Existence of Mori fibre spaces for 3-folds in char p, Adv. Math. 313 (2017), 62–101.10.1016/j.aim.2017.03.032Google Scholar
Boucksom, S., Demailly, J.-P., Păun, M. and Peternell, T., The pseudo-effective cone of a compact Kähler manifold and varieties of negative Kodaira dimension, J. Algebraic Geom. 22(2) (2013), 201–248.10.1090/S1056-3911-2012-00574-8Google Scholar
Campana, F., Slope rational connectedness for orbifolds, preprint, 2016, arXiv:1607.07829.Google Scholar
Debarre, O., Higher-dimensional Algebraic Geometry, Universitext. Springer, New York, 2001.10.1007/978-1-4757-5406-3Google Scholar
Fujino, O., Fundamental theorems for the log minimal model program, Publ. Res. Inst. Math. Sci. 47(3) (2011), 727–789.10.2977/PRIMS/50Google Scholar
Fulger, M. and Lehmann, B., Zariski decompositions of numerical cycle classes, J. Algebraic Geom. 26(1) (2017), 43–106.10.1090/jag/677Google Scholar
Hacon, C. D. and Xu, C., On the three dimensional minimal model program in positive characteristic, J. Amer. Math. Soc. 28(3) (2015), 711–744.10.1090/S0894-0347-2014-00809-2Google Scholar
Kollár, J., Effective base point freeness, Math. Ann. 296(4) (1993), 595–605.10.1007/BF01445123Google Scholar
Kollár, J. and Mori, S., Birational Geometry of Algebraic Varieties, Cambridge Tracts in Mathematics 134, Cambridge University Press, Cambridge, 1998, with the collaboration of C. H. Clemens and A. Corti, Translated from the 1998 Japanese original.10.1017/CBO9780511662560Google Scholar
Lazarsfeld, R., “Positivity in algebraic geometry. I”, in Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics] 48, Springer, Berlin, 2004, Classical setting: line bundles and linear series.Google Scholar
Lazarsfeld, R., “Positivity in algebraic geometry. II”, in Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics] 49, Springer, Berlin, 2004, Positivity for vector bundles, and multiplier ideals.Google Scholar
Lehmann, B., A cone theorem for nef curves, J. Algebraic Geom. 21(3) (2012), 473–493.10.1090/S1056-3911-2011-00580-8Google Scholar
Miyaoka, Y., “Rational curves on algebraic varieties”, in Proceedings of the International Congress of Mathematicians, Vols 1, 2 (Zürich, 1994), Birkhäuser, Basel, 1995, 680–689.10.1007/978-3-0348-9078-6_61Google Scholar
Takagi, S., Fujita’s approximation theorem in positive characteristics, J. Math. Kyoto Univ. 47(1) (2007), 179–202.10.1215/kjm/1250281075Google Scholar
Tanaka, H., Semiample perturbations for log canonical varieties over an F-finite field containing an infinite perfect field, Internat. J. Math. 28(5) (2017), 1750030, 13.10.1142/S0129167X17500306Google Scholar
Waldron, J., The lmmp for log canonical 3-folds in characteristic p > 5, Nagoya Math. J. (2017), 1–24.+5,+Nagoya+Math.+J.+(2017),+1–24.>Google Scholar