Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Morey, Susan
Noh, Sunsook
and
Vasconcelos, Wolmer V.
1995.
Symbolic powers, Serre conditions and Cohen-Macaulay Rees algebras.
Manuscripta Mathematica,
Vol. 86,
Issue. 1,
p.
113.
Cortadellas, Teresa
1996.
Depth formulas for the rees algebras of filtrations.
Communications in Algebra,
Vol. 24,
Issue. 2,
p.
705.
Herrmann, Manfred
Hyry, Eero
and
Korb, Thomas
1996.
Filter-regularity and cohen-macaulay multigraded rees algebras.
Communications in Algebra,
Vol. 24,
Issue. 6,
p.
2177.
Huckaba, Sam
and
Marley, Thomas
1999.
On Associated Graded Rings of Normal Ideals.
Journal of Algebra,
Vol. 222,
Issue. 1,
p.
146.
Goto, Shiro
1999.
Buchsbaumness in Rees Algebras Associated to Ideals of Minimal Multiplicity.
Journal of Algebra,
Vol. 213,
Issue. 2,
p.
604.
Cortadellas, Teresa
2000.
On the depth of the canonical modules of graded rings associated to an ideal.
Communications in Algebra,
Vol. 28,
Issue. 2,
p.
601.
Goto, Shiro
2000.
Cohen–Macaulayness and negativity of A-invariants in Rees algebras associated to m-primary ideals of minimal multiplicity.
Journal of Pure and Applied Algebra,
Vol. 152,
Issue. 1-3,
p.
93.
Cortadellas, Teresa
and
Zarzuela, Santiago
2001.
Burch's inequality and the depth of the blow up rings of an ideal.
Journal of Pure and Applied Algebra,
Vol. 157,
Issue. 2-3,
p.
183.
Zarzuela, S
and
Cortadellas, T
2001.
Geometric And Combinatorial Aspects Of Commutative Algebra.
Morey, S
and
Vasconcelos, W
2001.
Ring Theory And Algebraic Geometry.
Elias, Juan
2002.
On the Grauert–Riemenschneider Vanishing Theorem.
Journal of Algebra,
Vol. 248,
Issue. 1,
p.
265.
Ghezzi, Laura
2002.
On the Depth of the Associated Graded Ring of an Ideal.
Journal of Algebra,
Vol. 248,
Issue. 2,
p.
688.
Aberbach, Ian
Ghezzi, Laura
and
Tài Hà, Huy
2004.
The depth of the associated graded ring of ideals with any reduction number.
Journal of Algebra,
Vol. 276,
Issue. 1,
p.
168.
Nishida, Koji
2005.
On the depth of the associated graded ring of a filtration.
Journal of Algebra,
Vol. 285,
Issue. 1,
p.
182.
Puthenpurakal, Tony J.
2007.
Ratliff–Rush filtration, regularity and depth of higher associated graded modules: Part I.
Journal of Pure and Applied Algebra,
Vol. 208,
Issue. 1,
p.
159.
Colomé-Nin, Gemma
and
Elias, Juan
2009.
Cohomological properties of non-standard multigraded modules.
Journal of Algebra,
Vol. 322,
Issue. 5,
p.
1415.
Branco Correia, Ana L.
and
Zarzuela, Santiago
2010.
On the depth of the Rees algebra of an ideal module.
Journal of Algebra,
Vol. 323,
Issue. 5,
p.
1503.
Miyazaki, Mitsuhiro
2010.
On the discrete counterparts of algebras with straightening laws.
Journal of Commutative Algebra,
Vol. 2,
Issue. 1,
Kustin, Andrew R.
Polini, Claudia
and
Ulrich, Bernd
2011.
Rational normal scrolls and the defining equations of Rees algebras.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2011,
Issue. 650,
Ozeki, Kazuho
2012.
The equality of Elias–Valla and the associated graded ring of maximal ideals.
Journal of Pure and Applied Algebra,
Vol. 216,
Issue. 6,
p.
1306.


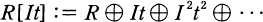
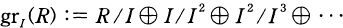 .
.