Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-23T23:59:29.826Z Has data issue: false hasContentIssue false
  • English
  • Français

Commuting Families in Hecke and Temperley-Lieb Algebras

Published online by Cambridge University Press:  11 January 2016

Tom Halverson ,
Manuela Mazzocco  and
Arun Ram
Tom Halverson
Affiliation:
Department of MathematicsMacalester College, Saint Paul, MN 55105, [email protected]
Manuela Mazzocco
Affiliation:
Department of MathematicsUniversity of Wisconsin, Madison, WI 53706, U.S.A.
Arun Ram
Affiliation:
Department of Mathematics and StatisticsUniversity of Melbourne, Parkville VIC 3010, [email protected], Department of MathematicsUniversity of Wisconsin Madison, WI 53706, U.S.A.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We define analogs of the Jucys-Murphy elements for the affine Temperley-Lieb algebra and give their explicit expansion in terms of the basis of planar Brauer diagrams. These Jucys-Murphy elements are a family of commuting elements in the affine Temperley-Lieb algebra, and we compute their eigenvalues on the generic irreducible representations. We show that they come from Jucys-Murphy elements in the affine Hecke algebra of type A, which in turn come from the Casimir element of the quantum group . 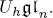We also give the explicit specializations of these results to the finite Temperley-Lieb algebra.

Keywords

20G0516G9981R5082B20
Type
Research Article
Information
Nagoya Mathematical Journal , Volume 195 , 2009 , pp. 125 - 152
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 2009

References

[Ar] Ariki, S., Representations of quantum algebras and combinatorics of Young tableaux, University Lecture Series 26, Amer. Math. Soc, Providence, RI, 2002. ISBN: 0-8218-3232-8, MR1911030 (2004b:17022)Google Scholar
[Ch] Cherednik, I., A new interpretation of Gel’fand-Tzetlin bases, Duke Math. J., 54 (1987), no. 2, 563–577.CrossRefGoogle Scholar
[Dr] Drinfel’d, V. G., Almost cocommutative Hopf algebras, Leningrad Math. J., 1 (1990), 321–342.Google Scholar
[Dx] Dixmier, J., Enveloping algebras, Graduate Studies in Mathematics 11, Amer. Math. Soc, Providence, RI, 1996. ISBN: 0-8218-0560-6, MR1393197 (97c:17010)Google Scholar
[FH] Fulton, W. and Harris, J., Representation theory. A first course, Graduate Texts in Mathematics 129, Springer-Verlag, New York 1991. ISBN: 0-387-97527-6; 0-387-97495-4, MR1153249 (93a:20069)Google Scholar
[GHJ] Goodman, F., la Harpe, P. de, and Jones, V., Coxeter graphs and towers of algebras, Mathematical Sciences Research Institute Publications 14, Springer-Verlag, New York, 1989.Google Scholar
[GL1] Graham, J. and Lehrer, G., Diagram algebras, Hecke algebras and decomposition numbers at roots of unity, Ann. Scient. Ec. Norm. Sup. 4 e série 36 (2003), no. 4, 479–524. MR2013924 (2004k:20007)Google Scholar
[GL2] Graham, J. and Lehrer, G., Cellular and diagram algebras in representation theory, Representation theory of algebraic groups and quantum groups, Adv. Stud. Pure Math. 40, Math. Soc. Japan, Tokyo, 2004, pp. 141–173. MR2074593 (2005i:20005)Google Scholar
[Gr] Grojnowski, I., Affine s lp controls the representation theory of the symmetric group and related Hecke algebras, arxiv:math.RT/9907129.Google Scholar
[Ji] Jimbo, M., A q-analogue of U(gl(N + 1)), Hecke algebra, and the Yang-Baxter equation, Lett. Math. Phys., 11 (1986), no. 3, 247–252. MR0841713 (87k:17011)Google Scholar
[K] Kleshchev, A., Linear and projective representations of symmetric groups, Cambridge Tracts in Mathematics 163, Cambridge University Press, Cambridge, 2005. ISBN: 0-521-83703-0, MR2165457 (2007b:20022)Google Scholar
[KL] Kazhdan, D. and Lusztig, G., Proof of the Deligne-Langlands conjecture for Hecke algebras, Invent. Math., 87 (1987), no. 1, 153–215. MR0862716 (88d:11121)CrossRefGoogle Scholar
[LR] Leduc, R. and Ram, A., A ribbon Hopf algebra approach to the irreducible representations of centralizer algebras: the Brauer, Birman-Wenzl, and type A Iwahori-Hecke algebras, Adv. Math., 125 (1997), 1–94. MR1427801 (98c:20015)Google Scholar
[Mac] Macdonald, I. G., Symmetric functions and Hall polynomials, Second Edition, Oxford University Press, 1995.Google Scholar
[OR] Orellana, R. and Ram, A., Affine braids, Markov traces and the category O, Proceedings of the International Colloquium on Algebraic Groups and Homogeneous Spaces, Mumbai 2004, Tata Institute of Fundamental Research (TIFR), Mumbai, Narosa Publishing House, 2007, pp. 423–473.Google Scholar
[OV] Okounkov, A. and Vershik, A., A new approach to representation theory of symmetric groups, Selecta Math. (N.S.), 2 (1996), no. 4, 581–605. MR1443185 (99g:20024)Google Scholar
[R] Ram, A., Skew shape representations are irreducible, Combinatorial and Geometric representation theory (Kang, S.-J. and Lee, K.-H., eds.), Contemp. Math. 325, Amer. Math. Soc., 2003, pp. 161–189. MR1988991 (2004f:20014)Google Scholar
[Re] Reshetikhin, N. Yu., Quantized universal enveloping algebras, the Yang-Baxter equation, and invariants of links I, LOMI Preprint E-4-87 (1987).Google Scholar