 $\ell$ LOCAL LANGLANDS CORRESPONDENCE BY NILPOTENT GAMMA FACTORS
$\ell$ LOCAL LANGLANDS CORRESPONDENCE BY NILPOTENT GAMMA FACTORSPublished online by Cambridge University Press: 12 May 2020
Let 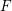 $F$ be a
$F$ be a 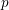 $p$-adic field and choose
$p$-adic field and choose 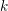 $k$ an algebraic closure of
$k$ an algebraic closure of  $\mathbb{F}_{\ell }$, with
$\mathbb{F}_{\ell }$, with  $\ell$ different from
$\ell$ different from  $p$. We define “nilpotent lifts” of irreducible generic
$p$. We define “nilpotent lifts” of irreducible generic 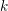 $k$-representations of
$k$-representations of 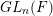 $GL_{n}(F)$, which take coefficients in Artin local
$GL_{n}(F)$, which take coefficients in Artin local  $k$-algebras. We show that an irreducible generic
$k$-algebras. We show that an irreducible generic  $\ell$-modular representation
$\ell$-modular representation  $\unicode[STIX]{x1D70B}$ of
$\unicode[STIX]{x1D70B}$ of 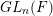 $GL_{n}(F)$ is uniquely determined by its collection of Rankin–Selberg gamma factors
$GL_{n}(F)$ is uniquely determined by its collection of Rankin–Selberg gamma factors 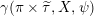 $\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D70B}\times \widetilde{\unicode[STIX]{x1D70F}},X,\unicode[STIX]{x1D713})$ as
$\unicode[STIX]{x1D6FE}(\unicode[STIX]{x1D70B}\times \widetilde{\unicode[STIX]{x1D70F}},X,\unicode[STIX]{x1D713})$ as 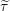 $\widetilde{\unicode[STIX]{x1D70F}}$ varies over nilpotent lifts of irreducible generic
$\widetilde{\unicode[STIX]{x1D70F}}$ varies over nilpotent lifts of irreducible generic  $k$-representations
$k$-representations  $\unicode[STIX]{x1D70F}$ of
$\unicode[STIX]{x1D70F}$ of 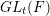 $GL_{t}(F)$ for
$GL_{t}(F)$ for  $t=1,\ldots ,\lfloor \frac{n}{2}\rfloor$. This gives a characterization of the mod-
$t=1,\ldots ,\lfloor \frac{n}{2}\rfloor$. This gives a characterization of the mod- $\ell$ local Langlands correspondence in terms of gamma factors, assuming it can be extended to a surjective local Langlands correspondence on nilpotent lifts.
$\ell$ local Langlands correspondence in terms of gamma factors, assuming it can be extended to a surjective local Langlands correspondence on nilpotent lifts.