Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
DONG, Chen
and
MATSUZAWA, Tadato
1993.
<i>S</i>-spaces of Gel'fand-Shilov and differential equations.
Japanese journal of mathematics. New series,
Vol. 19,
Issue. 2,
p.
227.
Chung, Soon-Yeong
and
NA, Ji-Young
2000.
Rotation Inveriant Generalized Functioins∗.
Integral Transforms and Special Functions,
Vol. 10,
Issue. 1,
p.
25.
Chung, Jaeyoung
2003.
Stability of functional equations in the spaces of distributions and hyperfunctions.
Journal of Mathematical Analysis and Applications,
Vol. 286,
Issue. 1,
p.
177.
Chung, Jaeyoung
Chung, Soon-Yeong
and
Kim, Dohan
2004.
The stability of Cauchy equations in the space of Schwartz distributions.
Journal of Mathematical Analysis and Applications,
Vol. 295,
Issue. 1,
p.
107.
Chung, Jaeyoung
2004.
Hyers–Ulam–Rassias stability of Cauchy equation in the space of Schwartz distributions.
Journal of Mathematical Analysis and Applications,
Vol. 300,
Issue. 2,
p.
343.
Chung *, Jaeyoung
Kim †, Dohan
and
Shin ‡, Chang Eon
2004.
Fourier transforms via heat kernel.
Integral Transforms and Special Functions,
Vol. 15,
Issue. 4,
p.
295.
Li, Linsong
Chung, Jaeyoung
and
Kim, Dohan
2004.
Stability of Jensen equations in the space of generalized functions.
Journal of Mathematical Analysis and Applications,
Vol. 299,
Issue. 2,
p.
578.
DHUNGANA, Bishnu P.
2006.
Mehler Kernel Approach to Tempered Distributions.
Tokyo Journal of Mathematics,
Vol. 29,
Issue. 2,
Yoshino, Kunio
and
Suwa, Masanori
2006.
The Bochner–Schwartz theorem for Fourier ultra-hyperfunctions.
Integral Transforms and Special Functions,
Vol. 17,
Issue. 2-3,
p.
109.
Chung, Jaeyoung
2006.
Approximately additive Schwartz distributions.
Journal of Mathematical Analysis and Applications,
Vol. 324,
Issue. 2,
p.
1449.
Lee, Young-Su
and
Chung, Soon-Yeong
2006.
Stability of a quadratic Jensen type functional equation in the spaces of generalized functions.
Journal of Mathematical Analysis and Applications,
Vol. 324,
Issue. 2,
p.
1395.
Yoshino, Kunio
and
Suwa¶, Masanori
2006.
The structure of positive definite Fourier ultra-hyperfunctions.
Complex Variables and Elliptic Equations,
Vol. 51,
Issue. 5-6,
p.
611.
Kim, Jong-Ho
Chung, Yun-Sung
and
Chung, Soon-Yeong
2006.
Stability of a Jensen type equation in the space of generalized functions.
Journal of Mathematical Analysis and Applications,
Vol. 321,
Issue. 1,
p.
114.
Lee, Young-Su
and
Chung, Soon-Yeong
2007.
Stability for quadratic functional equation in the spaces of generalized functions.
Journal of Mathematical Analysis and Applications,
Vol. 336,
Issue. 1,
p.
101.
Chung, Jaeyoung
2007.
Stability of approximately quadratic Schwartz distributions.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 67,
Issue. 1,
p.
175.
Chung, Jaeyoung
2008.
Stability of exponential equations in Schwartz distributions.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 69,
Issue. 10,
p.
3503.
Chung, Jae-Young
Kim, Dohan
and
Rassias, John Michael
2009.
Hyers–Ulam stability on a generalized quadratic functional equation in distributions and hyperfunctions.
Journal of Mathematical Physics,
Vol. 50,
Issue. 11,
Chung, Jae-Young
2010.
A heat kernel approach to the stability of exponential equation in distributions and hyperfunctions.
Journal of Mathematical Physics,
Vol. 51,
Issue. 5,
Chung, Jae-Young
2011.
Functional Equations in Mathematical Analysis.
Vol. 52,
Issue. ,
p.
59.
OKA, Yasuyuki
2011.
Asymptotic Expansions of Solutions to the Heat Equations with Initial Value in the Dual of Gel'fand-Shilov Spaces.
Tokyo Journal of Mathematics,
Vol. 34,
Issue. 2,


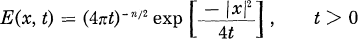 .
.