Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Rodin, Burton
and
Sario, Leo
1967.
Convergence of normal operators.
Kodai Mathematical Journal,
Vol. 19,
Issue. 2,
Nakai, Mitsuru
and
Sario, Leo
1967.
Harmonic fields with given boundary behavior in Riemannian spaces.
Journal d'Analyse Mathématique,
Vol. 18,
Issue. 1,
p.
245.


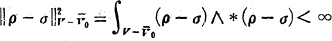 was given a complete solution in [2]. The corresponding problem for harmonic forms
was given a complete solution in [2]. The corresponding problem for harmonic forms  by the point norm [3].
by the point norm [3].