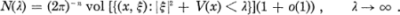Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Iwatsuka, Akira
1990.
Functional-Analytic Methods for Partial Differential Equations.
Vol. 1450,
Issue. ,
p.
157.
Ikeda, N.
1990.
École d'Été de Probabilités de Saint-Flour XVIII - 1988.
Vol. 1427,
Issue. ,
p.
195.
Raikov, George D.
1990.
Eigenvalue asymptotics for the södinger operator.
Communications in Partial Differential Equations,
Vol. 15,
Issue. 3,
p.
407.
Matsumoto, Hiroyuki
1991.
Classical and non-classical eigenvalue asymptotics for magnetic Schrödinger operators.
Journal of Functional Analysis,
Vol. 95,
Issue. 2,
p.
460.
Matsumoto, Hiroyuki
1994.
Semiclassical asymptotics of eigenvalue distributions for schrödinger operators with magnetic fields.
Communications in Partial Differential Equations,
Vol. 19,
Issue. 5-6,
p.
719.
Levendorskii, S.Z.
1997.
Spectral Properties of Schrödinger Operators with Irregular Magnetic Potentials, for a Spin 12 Particle.
Journal of Mathematical Analysis and Applications,
Vol. 216,
Issue. 1,
p.
48.
Kondratiev, Vladimir
Maz'ya, Vladimir
and
Shubin, Mikhail
2005.
Discreteness of Spectrum and Strict Positivity Criteria for Magnetic Schrödinger Operators.
Communications in Partial Differential Equations,
Vol. 29,
Issue. 3-4,
p.
489.
KACHMAR, AYMAN
2008.
WEYL ASYMPTOTICS FOR MAGNETIC SCHRÖDINGER OPERATORS AND DE GENNES' BOUNDARY CONDITION.
Reviews in Mathematical Physics,
Vol. 20,
Issue. 08,
p.
901.
Truc, Françoise
2011.
Spectral Theory and Analysis.
p.
135.
Fournais, Søren
Frank, Rupert L.
Goffeng, Magnus
Kachmar, Ayman
and
Sundqvist, Mikael
2025.
Counting negative eigenvalues for the magnetic Pauli operator.
Duke Mathematical Journal,
Vol. 174,
Issue. 2,