Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
1993.
Global Affine Differential Geometry of Hypersurfaces.
Dillen, Franki
Vrancken, Luc
and
Leuven, K. U.
1993.
The classification of 3-dimensional locally strongly convex homogeneous affine hypersurfaces.
Manuscripta Mathematica,
Vol. 80,
Issue. 1,
p.
165.
Dillen, Franki
and
Vrancken, Luc
1994.
Calabi-type composition of affine spheres.
Differential Geometry and its Applications,
Vol. 4,
Issue. 4,
p.
303.
Dillen, Franki
Vrancken, Luc
and
Yaprak, Sahnur
1994.
Affine hypersurfaces with parallel cubic form.
Nagoya Mathematical Journal,
Vol. 135,
Issue. ,
p.
153.
DILLEN, Franki
and
VRANCKEN, Luc
1998.
Hypersurfaces with parallel difference tensor.
Japanese journal of mathematics. New series,
Vol. 24,
Issue. 1,
p.
43.
Dillen, Franki
and
Vrancken, Luc
1998.
When Do Some Geodesics of the Induced and the Metrical Connection Coincide?.
Mathematische Nachrichten,
Vol. 192,
Issue. 1,
p.
173.
Chen, Bang-Yen
2005.
An Optimal Inequality and Extremal Classes of Affine Spheres in Centroaffine Geometry.
Geometriae Dedicata,
Vol. 111,
Issue. 1,
p.
187.
Hu, Zejun
Li, Haizhong
Simon, Udo
and
Vrancken, Luc
2009.
On locally strongly convex affine hypersurfaces with parallel cubic form. Part I.
Differential Geometry and its Applications,
Vol. 27,
Issue. 2,
p.
188.
Hu, Zejun
and
Li, Cece
2011.
The classification of 3-dimensional Lorentzian affine hypersurfaces with parallel cubic form.
Differential Geometry and its Applications,
Vol. 29,
Issue. 3,
p.
361.
Hu, Zejun
Li, Cece
Li, Haizhong
and
Vrancken, Luc
2011.
Lorentzian Affine Hypersurfaces with Parallel Cubic Form.
Results in Mathematics,
Vol. 59,
Issue. 3-4,
p.
577.
Hu, Zejun
Li, Cece
Li, Haizhong
and
Vrancken, Luc
2011.
The classification of 4-dimensional non-degenerate affine hypersurfaces with parallel cubic form.
Journal of Geometry and Physics,
Vol. 61,
Issue. 11,
p.
2035.
Li, Cece
2014.
Affine hypersurfaces with parallel difference tensor relative to affineα-connection.
Journal of Geometry and Physics,
Vol. 86,
Issue. ,
p.
81.
Hu, Zejun
Li, Cece
and
Zhang, Chuanjing
2014.
On quasi-umbilical locally strongly convex homogeneous affine hypersurfaces.
Differential Geometry and its Applications,
Vol. 33,
Issue. ,
p.
46.
Hildebrand, Roland
2015.
Centro-affine hypersurface immersions with parallel cubic form.
Beiträge zur Algebra und Geometrie / Contributions to Algebra and Geometry,
Vol. 56,
Issue. 2,
p.
593.
Li, Cece
and
Zhang, Dong
2015.
The Generalized Cayley Hypersurfaces and Their Geometrical Characterization.
Results in Mathematics,
Vol. 68,
Issue. 1-2,
p.
25.
Cheng, Xiuxiu
Hu, Zejun
and
Moruz, Marilena
2017.
Classification of the Locally Strongly Convex Centroaffine Hypersurfaces with Parallel Cubic Form.
Results in Mathematics,
Vol. 72,
Issue. 1-2,
p.
419.
Cheng, Xiuxiu
Hu, Zejun
Moruz, Marilena
and
Vrancken, Luc
2020.
On product affine hyperspheres in ℝn+1.
Science China Mathematics,
Vol. 63,
Issue. 10,
p.
2055.
Szancer, Zuzanna
2020.
On $$\widetilde{J}$$-tangent Affine Hyperspheres.
Results in Mathematics,
Vol. 75,
Issue. 3,
Szancer, Michal
and
Szancer, Zuzanna
2020.
On 4‐dimensional Lorentzian affine hypersurfaces with an almost symplectic form.
Mathematische Nachrichten,
Vol. 293,
Issue. 8,
p.
1613.
Xu, Ruiwei
and
Lei, Miaoxin
2021.
Classification of Calabi hypersurfaces with parallel Fubini-Pick form.
Differential Geometry and its Applications,
Vol. 74,
Issue. ,
p.
101707.
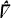 of the affine metric h.
of the affine metric h.