Introduction
Metals and their alloys are in ubiquitous use, from bicycles to airplane wings to space frames. Generally, we prefer them in their solid state, and in most cases, producing them necessitates cooling down a high-temperature liquid. Indeed, solidification from a liquid is inherently advantageous for the commercial production of bulk materials, due to the greater mass processing rates compared to growth of crystalline layers from a vapor phase (e.g., chemical vapor deposition).Reference Rudolph1 As noted by Derby,Reference Rudolph1 this is simply due to the higher density of a condensed-liquid phase, in contrast to the low density of a gaseous phase, especially under near-vacuum conditions employed in molecular beam epitaxial processes.
On a practical note, more than 1.8 billion tons of metal was solidified globally in 2019 alone.2 Since many metals are used in their as-cast state (i.e., without further thermal or mechanical processing), it follows that the microscopic structure formed by solidification has a direct consequence on the mechanical properties of the metal product. Castings are produced with dimensions of a few millimeters up to tens of meters, and thus one may infer that the important dimensions to describe solidification cross orders of magnitude. This is true not only in length scale, but also time scale. However, it is the solidification microstructure (on the order of tens to hundreds of micrometers) that ultimately determines the physical properties and performance of metals and alloys.Reference Stefanescu3 Because solidification is the process by which atoms are transferred from liquid to solid, the distances over which individual atoms diffuse (nanometers) are also important. An accurate description of solidification must therefore reconcile the various processes that occur over several order-of-magnitudes in length scale and also time scale,Reference Stefanescu3 which is an important challenge in its own right.
Mastering solidification dynamics is a primary aim in process metallurgy and synthesis science. The end goal is to understand the formation of solid phases and their shapes and patterns that develop from a “disordered” liquid environment (although we note that local ordering in the liquid is an area ripe for further understanding), in addition to growth regime (or morphological) transitions. These microscale patterns span dendrites to cells to labyrinths to spirals.Reference Glicksman, Schaefer and Ayers4–Reference Moniri, Bale, Volkenandt, Wang, Gao, Lu, Sun, Ritchie and Shahani9 Questions that arise include: Is each of these diverse patterns the result of unique causes and effects, or are there unifying physical principles that govern their growth and form?Reference Ben-Jacob and Garik10 How do the solidification processing conditions influence phase formation and pattern selection? The past few years have seen the development of such principles, in some cases validating or expanding upon past theoretical treatments. These breakthroughs have been achieved with the aid of in situ and complementary ex situ characterization techniques, new analysis methods, and multiscale modeling approaches. These themes are reflected in the articles in this issue.
A hierarchy of equilibrium
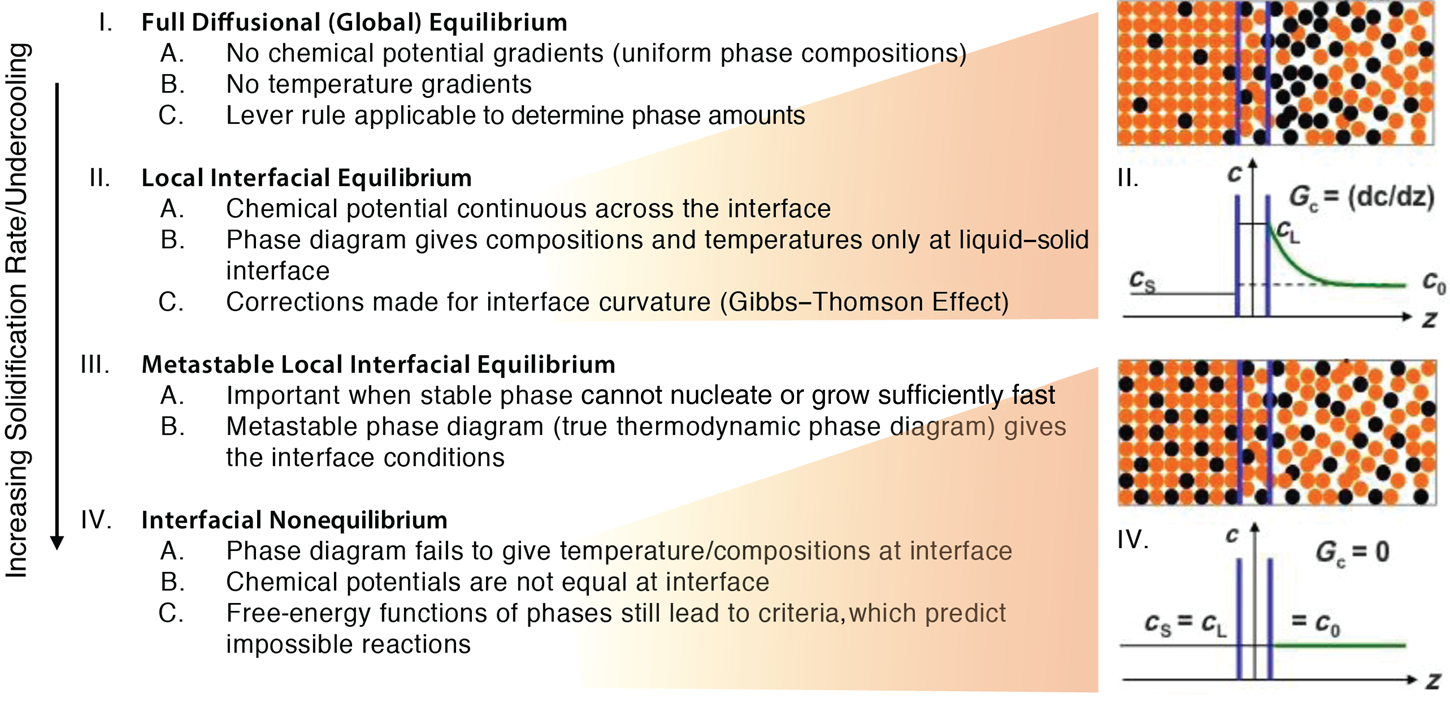
Solidification is generally considered a nonequilibrium process. In other words, any motion of the solid–liquid interface requires a thermodynamic driving force (such as undercooling or supersaturation).Reference Trivedi and Kurz11 That said, there exist different degrees of nonequilibrium that constitute a hierarchy, as first popularized by Boettinger and Perepezko in the 1980s.Reference Stefanescu3,Reference Boettinger, Perepezko and Liebermann12
As shown in Figure 1, depending on the amount of undercooling or the solidification rate, the liquid-to-solid transformation changes from fully diffusional to nondiffusional. The conditions required for global equilibrium (I) for metals and alloys are usually obtained on geological times. More relevant to the solidification of most castings are the conditions defined in (II), where the equilibrium phase diagram is invoked to establish the temperature and composition of the phase boundaries (e.g., the solid–liquid interfaces). This local equilibrium assumption holds, so long as the ratio of the velocity of solid growth V to the velocity of mass transport D/ℓ is small, where D is the liquid diffusion coefficient and ℓ is a characteristic length scale of the microstructure. In other words, the growth Péclet number Pe = V ℓ/D ≪ 1. This dimensionless parameter indicates the relative scale of the solid-liquid interfacial velocity to the relaxation speed of the solute atoms in the liquid phase.

Figure 1. Hierarchy of equilibrium that follows with an increasing solidification rate or undercooling (left), with illustrations of corresponding solute concentration profiles (right).Reference Boettinger, Perepezko and Liebermann12,Reference Herlach15
In some cases, a thermodynamically metastable phase grows in lieu of the stable phase, due to difficulties in nucleation of the latter. Thus, metastable equilibrium (III) should be used locally at the interface. A salient example is the gray-to-white (stable-to-metastable) transition in cast iron as the solidification rate increases.Reference Magnin and Kurz13 Another example is the growth of metastable quasicrystals.Reference Shechtman, Blech, Gratias and Cahn14 For both (II) and (III) in Figure 1, the chemical potentials of the components must be equal across the interface for the liquid and the solid. However, at high solidification velocities, V, encountered in emerging technologies such as additive manufacturing (AM) of metals and alloys, there is much less time for interfacial equilibration, and hence significant departures from equilibrium (IV) are to be expected. Under such conditions, the crystal growth rate exceeds the diffusive speed of the solute atoms in the liquid phase and the solute is trapped at the growth front.Reference Herlach15 That is, solidification proceeds without partitioning of solute at the moving solid–liquid interface. The impossibility of partitionless solidification is sometimes associated with glass formation.Reference Boettinger, Kear, Giessen and Cohen16,Reference Greer17
Effect of fluid flow
Most theoretical models of the development of solidification microstructures are based on diffusive growth conditions, whereas convection is also generally present in most terrestrial solidification experiments.Reference Trivedi, Miyahara, Mazumder, Simsek and Tewari18 This is particularly true for cellular and dendritic growth at low velocities,Reference Clarke, Tourret, Song, Imhoff, Gibbs, Gibbs, Fezzaa and Karma8,Reference Dupouy, Camel and Favier19 and for layer formation in peritectic systems.Reference Park and Trivedi20 In these examples, the fluid flow velocity may be higher than the interfacial velocity, especially for bulk metallic samples (typically greater than 1 mm in diameterReference Trivedi, Miyahara, Mazumder, Simsek and Tewari18), and thus the influence of convection cannot be neglected. In turn, fluid motion leads to a redistribution of solute and a resulting modification to the solid morphology. For a given fluid flow intensity, the magnitude of the Prandtl number Pr = υ/α indicates the importance of the convective heat transfer, where υ is the kinematic viscosity and α is the thermal diffusivity. Typically, molten metals and semiconductors have small characteristic Prandtl numbers of Pr ≈ 0.01, whereas nonmetallic melts, such as nearly all organic materials, often have Pr ≈ 1–100 or higher.Reference Rudolph1 Thus, solidification processes in metals may exhibit a greater degree of complexity than in organic systems, since buoyancy-driven flow is more prominent in the former. These considerations of heat transfer also suggest that organic materials may not necessarily mimic the growth behavior of metallic alloys, as had been assumed previously.Reference Jackson21 Such organic materials are optically transparent and thus traditionally have been used to capture the interfacial morphology during solidification, aiding in the development and testing of solidification theory.
In practice, fluid flow may be generated by various types of body forces or surface stresses such as thermo-capillary effects, as summarized in Reference 22. The most common culprits are gravity, magnetic fields, and magneto-thermo-electric effects.Reference Hachani, Wang, Kaldre, Salloum-Abou-Jaoude, Budenkova, Reinhart, Zaidat, Mangelinck, Li, Nguyen Thi, Bojarevics, Ren, Buligins and Fautrelle22 Except in microgravity conditions, natural convection is unavoidable in solidification experiments. Rotating magnetic fields are often used to stir the bulk liquid or to create fragments that may initiate grain morphology changes (e.g., the columnar-to-equiaxed transition) of potential importance to metal casting and AM processes. In contrast, static magnetic fields may be applied to decrease the extent of fluid flow. Such is the case for electromagnetic braking in continuous casting of steel, wherein a magnetic field of intensity ≲1 T leads to a considerable slowing down of liquid flow in the mold.Reference Mazumdar and Evans23 Nevertheless, it has been demonstrated recently that static magnetic fields may also interact with Thomson–Seebeck currents to produce flows, the so-called magneto-thermo-electric currents.Reference Moreau, Laskar, Tanaka and Camel24,Reference Kao, Djambazov, Pericleous and Voller25 The influence of such fluid flows on microstructure formation depends on the length scale under consideration (see Figure 2).Reference Hachani, Wang, Kaldre, Salloum-Abou-Jaoude, Budenkova, Reinhart, Zaidat, Mangelinck, Li, Nguyen Thi, Bojarevics, Ren, Buligins and Fautrelle22 Large-scale flows disrupt heat and mass transport and mixing within the bulk liquid, leading to macrosegregation and remelting of primary dendrites. Meanwhile, small-scale flows impact the microstructure directly via solute transport within the mushy zone.Reference Hachani, Wang, Kaldre, Salloum-Abou-Jaoude, Budenkova, Reinhart, Zaidat, Mangelinck, Li, Nguyen Thi, Bojarevics, Ren, Buligins and Fautrelle22 Understanding segregation to predict solidification in metal alloys requires a synergy between multiphysical simulations and real-time experiments.

Figure 2. Correspondence between flow scales (bolded) and the imprints they leave in solidification (unbolded). Reprinted with permission from Reference 22. © 2014 Trans Tech Publications Ltd.
In this issue
The articles in this issue of MRS Bulletin highlight selected important aspects of current research in solidification science, providing fresh insights into the state of the art. They outline a field that draws much of its appeal to the processing-structure paradigm of materials science. For example, in their article in this issue, Pinomaa et al.Reference Pinomaa, Laukkanen and Provatas26 focus on solute trapping in rapid solidification (Figure 1[IV]) and its impact on solidification morphology and the emergent length scales. They introduce the phenomenological theories of solute trapping, which generally prescribe a velocity-dependent partition coefficient k(V) (i.e., the ratio of the solid composition to the liquid composition at the interface), as well as a velocity-dependent interface temperature T(V). One can then input k(V) into phase-field simulations to investigate microstructure evolution (e.g., side-branching instabilities in dendritic solidification).
Indispensable from theoretical advances are new modes of real-time characterization, which are needed to capture the full microstructural details. McKeown et al.Reference McKeown, Clarke and Wiezorek27 review in situ imaging techniques using novel probes—protons, x-rays, and electrons—which altogether provide complementary information on solid–liquid interfacial evolution and solidification dynamics across a range of length- and time scales in metallic alloys. Choice of technique depends upon the solidification phenomena and processing (e.g., casting, directional solidification, AM) of interest and the spatial and temporal resolutions available to capture the dynamics under different conditions. Such time-resolved experiments can be used to validate and inform multiscale modeling and simulations, which may otherwise involve a number of unknown parameters (e.g., the influence of convection, and solute trapping on the development of microstructure).
Sun et al.Reference Sun, Tan, Chen and Rollett28 expand upon the potential of synchrotron-based in situ x-ray imaging to shine light on laser–material interactions in AM. The x-ray measurements provide detailed information on the depression zone and its dynamical fluctuation (i.e., ground truths for process models). The “depression zone” refers to a vapor cavity that forms underneath the laser spot due to the recoil pressure induced by the localized vaporization.Reference King, Barth, Castillo, Gallegos, Gibbs, Hahn, Kamath and Rubenchik29 Such experiments can also be used to visualize the generation of defects, such as pores and cracks, which may limit the properties, performance, and lifetime of a material.
In their article in this issue, Feng et al.Reference Feng, Liotti, Wilson, Jowitt and Grant30 point out another promising application of synchrotron x-rays: chemical mapping in real time. Their approach combines x-ray radiography and fluorescence spectroscopy to determine, for example, equilibrium partition coefficients,Reference Kobayashi, Dobara, Todoroki, Nam, Morishita and Yasuda31 phase compositions, and segregation patterns. These data are otherwise unobtainable by theory or experiment, and thus may be used to parametrize solidification models accordingly. It is envisioned that synchrotron upgrade activities will afford a higher x-ray flux (by 10–1000×), and hence higher temporal resolution (toward kHz), amenable for visualizing the dynamics of chemical segregation under AM conditions.
At even higher cooling rates, nucleation of ground-state compounds may be suppressed entirely. What is the relationship between the developing short- and medium-range order in the undercooled liquid and the kinetic and thermodynamic stability of the nuclei that are forming? Kramer et al.Reference Kramer and Li32 address this question in their article. Molecular dynamics simulations suggest that the deeply undercooled liquid is not as “disordered” as previously imagined, but rather quite heterogeneous with nanoscale regions of varying topology and varying diffusivity. Interestingly, the formation of clusters or polytypes into extended structures in the liquid is thought to control both vitrification and phase selection. Levitation methods combined with synchrotron x-rays have provided some insight into the nature of changes in bonding upon undercooling, which can be qualitatively matched to simulations.Reference Hirata, Guan, Fujita, Hirotsu, Inoue, Yavari, Sakurai and Chen33
Outlook
Our understanding of metals solidification has evolved significantly over the past decade, thanks in part to the close collaborations between experimental and modeling research groups. These efforts have revealed the interplay between small-scale patterns and the larger-scale microstructural characteristics that impact the properties and performance of materials. This integrated approach has been termed “knowledge-based materials engineering,”Reference Hecht, Gránásy, Pusztai, Böttger, Apel, Witusiewicz, Ratke, De Wilde, Froyen, Camel, Drevet, Faivre, Fries, Legendre and Rex34 and is expected to accelerate the design and deployment of advanced metallic alloys and processes.
Even so, further advances in the field are needed to describe (1) the solidification of multicomponent, multiphase systems of industrial significance (e.g., high entropy alloys), in which convective fluid flow may play a dominant role; (2) the growth of complex intermetallics, which make up 94% of known compoundsReference Steurer and Dshemuchadse35 and may exhibit a relatively strong anisotropy in interfacial energy and interfacial mobility;Reference Gille and Belin-Ferré36,Reference Han, Xiao and Shahani37 (3) nonclassical or multistep nucleation pathways, which are typically associated with the presence of several free-energy minima;Reference Moniri, Bale, Volkenandt, Wang, Gao, Lu, Sun, Ritchie and Shahani9,Reference Kurtuldu, Shamlaye and Löffler38 (4) the relationship between eutectic growth and glass formation, and the competition between the two seen at high solidification rates; and (5) the relationship between high Pe growth in solidification and in solid-state transformation.Reference Asta, Beckermann, Karma, Kurz, Napolitano, Plapp, Purdy, Rappaz and Trivedi39 Answers to these questions depend not only on the integration of experiments and modeling/simulations, but also the availability of databases for thermophysical properties (e.g., composition-dependent interdiffusivities). This information may be obtained in a high-throughput manner through combinatorial experiments, as described elsewhere.Reference Chen and Zhang40
We hope this issue will inspire researchers to enter the field, harness the experimental and computational techniques introduced in the articles, and contribute their ideas to solving the open challenges remaining in solidification science.
 Ashwin J. Shahani is an assistant professor in the Department of Materials Science and Engineering at the University of Michigan. He earned his BS degree from Cornell University, and his PhD degree from Northwestern University, in materials science and engineering. His research group specializes in the development and application of in situ characterization methods for the study of phase and structural transformations in metals. His awards include the Air Force Young Investigator Research Program in 2017, the Army Young Investigator Research Program in 2018, and the National Science Foundation CAREER Award in 2019. Shahani can be reached by email at [email protected].
Ashwin J. Shahani is an assistant professor in the Department of Materials Science and Engineering at the University of Michigan. He earned his BS degree from Cornell University, and his PhD degree from Northwestern University, in materials science and engineering. His research group specializes in the development and application of in situ characterization methods for the study of phase and structural transformations in metals. His awards include the Air Force Young Investigator Research Program in 2017, the Army Young Investigator Research Program in 2018, and the National Science Foundation CAREER Award in 2019. Shahani can be reached by email at [email protected].
 Amy J. Clarke is an associate professor in the Department of Metallurgical and Materials Engineering, co-director for the Center for Advanced Non-Ferrous Structural Alloys, and a faculty member with the Advanced Steel Processing and Products Research Center, all at the Colorado School of Mines. She earned her BS degree from Michigan Technological University, and her MS and PhD degrees in metallurgical and materials engineering from the Colorado School of Mines. Her research focuses on physical metallurgy, and making, measuring, and modeling metallic alloys during processing to realize advanced manufacturing. Clarke can be reached by email at [email protected].
Amy J. Clarke is an associate professor in the Department of Metallurgical and Materials Engineering, co-director for the Center for Advanced Non-Ferrous Structural Alloys, and a faculty member with the Advanced Steel Processing and Products Research Center, all at the Colorado School of Mines. She earned her BS degree from Michigan Technological University, and her MS and PhD degrees in metallurgical and materials engineering from the Colorado School of Mines. Her research focuses on physical metallurgy, and making, measuring, and modeling metallic alloys during processing to realize advanced manufacturing. Clarke can be reached by email at [email protected].