No CrossRef data available.
Published online by Cambridge University Press: 26 February 2010
Let Γ be a finite group of order n and let K be a field whose characteristic does does not divide n. The group ring K(Γ) is then an involution algebra if we define  for y є Γ and extend by linearity, so that ¯ is trivial on K. A subalgebra T of K(Γ) is said to be an S-ring on Γ (see [4]) if there exists a decomposition
for y є Γ and extend by linearity, so that ¯ is trivial on K. A subalgebra T of K(Γ) is said to be an S-ring on Γ (see [4]) if there exists a decomposition
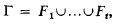
of Γ into non-empty, pairwise disjoint subsets Fi with the properties that the elements 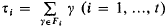 of K(Γ) form a K-basis of T and that for each τi there exists a τj such that
of K(Γ) form a K-basis of T and that for each τi there exists a τj such that 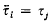 .
.