Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-26T17:43:51.585Z Has data issue: false hasContentIssue false
  • English
  • Français

On the positivity of solutions to the Smoluchowski equations

Part of: General theory in ordinary differential equations

Published online by Cambridge University Press:  26 February 2010

F. P. Da Costa
F. P. Da Costa
Affiliation:
Departamento de Matemática, Instituto Superior Tecnico, Av. Rovisco Pais, P-1096 Lisboa, Portugal.
Get access

Extract

The dynamics of cluster growth can be modelled by the following infinite system of ordinary differential equations, first proposed by Smoluchowski, [8],

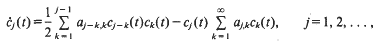
where cj=cj(t) represents the physical concentration of j-clusters (aggregates of j identical particles), aj,k=aj,k≥0 are the time-independent coagulation coefficients, measuring the effectiveness of the coagulation process between a j-cluster and a k-cluster, and the first sum in the right-hand side of (1) is defined to be zero if j = 1.

MSC classification

Secondary: 34A34: Nonlinear equations and systems, general
Type
Research Article
Information
Mathematika , Volume 42 , Issue 2 , December 1995 , pp. 406 - 412
Copyright
Copyright © University College London 1995

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Ball, J. and Carr, J.. The discrete coagulation-fragmentation equations: existence, uniqueness, and density conservation. J. Slat. Phys., 61 (1990), 203–234.Google Scholar
2.Buffet, E. and Pulé, J.. Gelation: the diagonal case revisited. Nonlinearity, 2 (1989), 373–381.CrossRefGoogle Scholar
3.Carr, J. and Costa, F. P. da. Instantaneous gelation in coagulation dynamics. Z. Angew. Math. Phys, 43 (1992), 974–983.CrossRefGoogle Scholar
4.Carr, J. and Costa, F. P. da. Asymptotic behavior of solutions to the coagulation-fragmentation equations. II. Weak fragmentation. J. Stat. Phys., 11 (1994), 89–123.CrossRefGoogle Scholar
5.Leyvraz, F.Existence and properties of post-gel solutions for the kinetic equations of coagulation. J. Phys. A: Math. Gen., 16 (1983), 2861–2873.CrossRefGoogle Scholar
6.Leyvraz, F. and Tschudi, H. R.. Singularities in the kinetics of coagulation processes. J. Phys. A: Math. Gen., 14 (1981), 3389–3405.CrossRefGoogle Scholar
7.Slemrod, M.. A note on the kinetic equations of coagulation. J. Integral Eq. and Appl., 3 (1991), 167–173.Google Scholar
8.Smoluchowski, M.. Drei Vortràge über Diffusion, Brownsche Bewegung und Koagulation von Kolloidteilchen. Physik Z., 17 (1916), 557–585.Google Scholar