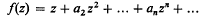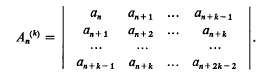Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Noonan, James W.
1974.
Coefficient differences and Hankel determinants of areally mean 𝑝-valent functions.
Proceedings of the American Mathematical Society,
Vol. 46,
Issue. 1,
p.
29.
Noonan, J. W.
and
Thomas, D. K.
1976.
On the second Hankel determinant of areally mean 𝑝-valent functions.
Transactions of the American Mathematical Society,
Vol. 223,
Issue. 0,
p.
337.
Hamilton, D. H.
1984.
A sharp form of the Ahlfors’ distortion theorem, with applications.
Transactions of the American Mathematical Society,
Vol. 282,
Issue. 2,
p.
799.
2008.
Nine Introductions in Complex Analysis.
Vol. 208,
Issue. ,
p.
473.
Ul-Haq, Wasim
and
Noor, Khalida Inayat
2012.
A certain class of analytic functions and the growth rate of Hankel determinant.
Journal of Inequalities and Applications,
Vol. 2012,
Issue. 1,
Lee, See Keong
Ravichandran, V
and
Supramaniam, Shamani
2013.
Bounds for the second Hankel determinant of certain univalent functions.
Journal of Inequalities and Applications,
Vol. 2013,
Issue. 1,
Krishna, D. Vamshee
2014.
Coefficient inequality for certain $p$-valent analytic functions.
Rocky Mountain Journal of Mathematics,
Vol. 44,
Issue. 6,
Liu, Ming-Sheng
Xu, Jun-Feng
and
Yang, Ming
2014.
Upper Bound of Second Hankel Determinant for Certain Subclasses of Analytic Functions.
Abstract and Applied Analysis,
Vol. 2014,
Issue. ,
p.
1.
Oshah, Anessa
and
Darus, Maslina
2015.
Upper bound of second Hankel determinant for a class of analytic functions associated with generalised derivative operator.
p.
35.
Vamshee Krishna, D.
and
Ram Reddy, T.
2015.
Coefficient inequality for certain subclasses of analytic functions associated with Hankel determinant.
Indian Journal of Pure and Applied Mathematics,
Vol. 46,
Issue. 1,
p.
91.
Prajapat, J. K.
Bansal, Deepak
Singh, Alok
and
Mishra, Ambuj K.
2015.
Bounds on third Hankel determinant for close-to-convex functions.
Acta Universitatis Sapientiae, Mathematica,
Vol. 7,
Issue. 2,
p.
210.
Al-Areefi, Najla M.
and
Mohd, Maisarah Haji
2015.
Best bounds for the second Hankel determinant of the kth-root transform of analytic functions.
Vol. 1682,
Issue. ,
p.
040014.
Mishra, Ambuj K.
Prajapat, Jugal K.
Maharana, Sudhananda
and
Srivastava, Hari M.
2016.
Bounds on Hankel determinant for starlike and convex functions with respect to symmetric points.
Cogent Mathematics,
Vol. 3,
Issue. 1,
p.
1160557.
Bharavi Sharma, Rayaprolu
and
Rajya Laxmi, Kalikota
2016.
Coefficient Inequalities of Second Hankel Determinants for Some Classes of Bi-Univalent Functions.
Mathematics,
Vol. 4,
Issue. 1,
p.
9.
Zaprawa, Paweł
2016.
Second Hankel Determinants for the Class of Typically Real Functions.
Abstract and Applied Analysis,
Vol. 2016,
Issue. ,
p.
1.
Inayat Noor, Khalida
Khan, Nazar
and
Zahoor Ahmad, Qazi
2017.
Coeffcient bounds for a subclass of multivalent functions of reciprocal order.
AIMS Mathematics,
Vol. 2,
Issue. 2,
p.
322.
Sharma, R. B.
and
Haripriya, M.
2017.
On a class of α-convex functions subordinate to a shell shaped region.
The Journal of Analysis,
Vol. 25,
Issue. 1,
p.
93.
Zaprawa, Paweł
2017.
Third Hankel Determinants for Subclasses of Univalent Functions.
Mediterranean Journal of Mathematics,
Vol. 14,
Issue. 1,
Zaprawa, Paweł
Figiel, Magdalena
and
Futa, Anna
2017.
On Coefficients Problems for Typically Real Functions Related to Gegenbauer Polynomials.
Mediterranean Journal of Mathematics,
Vol. 14,
Issue. 2,
Thomas, D. K.
and
Abdul Halim, S.
2017.
RETRACTED ARTICLE: Toeplitz Matrices Whose Elements are the Coefficients of Starlike and Close-to-Convex Functions.
Bulletin of the Malaysian Mathematical Sciences Society,
Vol. 40,
Issue. 4,
p.
1781.