Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Paouris, Grigoris
and
Pivovarov, Peter
2012.
A probabilistic take on isoperimetric-type inequalities.
Advances in Mathematics,
Vol. 230,
Issue. 3,
p.
1402.
Paouris, Grigoris
and
Pivovarov, Peter
2013.
Small-Ball Probabilities for the Volume of Random Convex Sets.
Discrete & Computational Geometry,
Vol. 49,
Issue. 3,
p.
601.
Stavrakakis, P.
and
Valettas, P.
2013.
Asymptotic Geometric Analysis.
Vol. 68,
Issue. ,
p.
359.
Paouris, Grigoris
and
Valettas, Petros
2014.
Neighborhoods on the Grassmannian of marginals with bounded isotropic constant.
Journal of Functional Analysis,
Vol. 267,
Issue. 9,
p.
3427.
Huang, QingZhong
and
He, BinWu
2014.
An asymmetric Orlicz centroid inequality for probability measures.
Science China Mathematics,
Vol. 57,
Issue. 6,
p.
1193.
Cordero-Erausquin, Dario
Fradelizi, Matthieu
Paouris, Grigoris
and
Pivovarov, Peter
2015.
Volume of the polar of random sets and shadow systems.
Mathematische Annalen,
Vol. 362,
Issue. 3-4,
p.
1305.
Klartag, Bo’az
2017.
Geometric Aspects of Functional Analysis.
Vol. 2169,
Issue. ,
p.
187.
Huang, Han
and
Slomka, Boaz A.
2019.
Approximations of convex bodies by measure-generated sets.
Geometriae Dedicata,
Vol. 200,
Issue. 1,
p.
173.
Haddad, J.
2021.
A convex body associated to the Busemann random simplex inequality and the Petty conjecture.
Journal of Functional Analysis,
Vol. 281,
Issue. 7,
p.
109118.
Lv, Songjun
2024.
Sharp norm estimates for functional dual affine quermassintegrals.
Banach Journal of Mathematical Analysis,
Vol. 18,
Issue. 2,


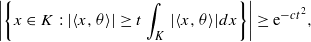
 , where
, where 