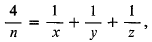Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Vaughan, R.C.
1973.
Some applications of Montgomery's sieve.
Journal of Number Theory,
Vol. 5,
Issue. 1,
p.
64.
Guy, Richard K.
1981.
Unsolved Problems in Number Theory.
p.
79.
Delang, Li
1982.
Letter to the editor.
Journal of Number Theory,
Vol. 15,
Issue. 2,
p.
282.
Martin, Greg
1999.
Dense Egyptian fractions.
Transactions of the American Mathematical Society,
Vol. 351,
Issue. 9,
p.
3641.
Croot, Ernest S
Dobbs, David E
Friedlander, John B
Hetzel, Andrew J
and
Pappalardi, Francesco
2000.
Binary Egyptian Fractions.
Journal of Number Theory,
Vol. 84,
Issue. 1,
p.
63.
Guy, Richard K.
2004.
Unsolved Problems in Number Theory.
Vol. 1,
Issue. ,
p.
209.
Huang, Jingjing
and
Vaughan, Robert C.
2011.
Mean value theorems for binary Egyptian fractions.
Journal of Number Theory,
Vol. 131,
Issue. 9,
p.
1641.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
307.
Jia, ChaoHua
2012.
The estimate for mean values on prime numbers relative to $\frac{4} {p} = \frac{1} {{n_1 }} + \frac{1} {{n_2 }} + \frac{1} {{n_3 }} $.
Science China Mathematics,
Vol. 55,
Issue. 3,
p.
465.
ELSHOLTZ, CHRISTIAN
and
TAO, TERENCE
2013.
COUNTING THE NUMBER OF SOLUTIONS TO THE ERDŐS–STRAUS EQUATION ON UNIT FRACTIONS.
Journal of the Australian Mathematical Society,
Vol. 94,
Issue. 1,
p.
50.
Bergner, Julia E.
and
Walker, Christopher D.
2015.
Groupoid Cardinality and Egyptian Fractions.
The College Mathematics Journal,
Vol. 46,
Issue. 2,
p.
122.
Subburam, S.
and
Togbé, Alain
2016.
A note on the Erdős–Straus conjecture.
Periodica Mathematica Hungarica,
Vol. 72,
Issue. 1,
p.
43.
Garcia, Stephan Ramon
O’Neill, Christopher
and
Yih, Samuel
2019.
Factorization length distribution for affine semigroups I: Numerical semigroups with three generators.
European Journal of Combinatorics,
Vol. 78,
Issue. ,
p.
190.
Xiao, Xuanxuan
and
Zhai, Wenguang
2020.
Error term of the mean value theorem for binary Egyptian fractions.
Open Mathematics,
Vol. 18,
Issue. 1,
p.
1250.
Gionfriddo, Mario
and
Guardo, Elena
2021.
A short proof of Erdös–Straus conjecture for everyn≡ 13 mod 24.
Journal of Interdisciplinary Mathematics,
Vol. 24,
Issue. 8,
p.
2307.