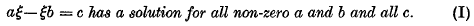Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Cohn, P. M.
1959.
On the free product of associative rings.
Mathematische Zeitschrift,
Vol. 71,
Issue. 1,
p.
380.
Cohn, P. M.
1959.
Simple rings without zero‐divisors, and Lie division rings.
Mathematika,
Vol. 6,
Issue. 1,
p.
14.
Jans, J. P.
1960.
Some Remarks on Symmetric and Frobenius Algebras.
Nagoya Mathematical Journal,
Vol. 16,
Issue. ,
p.
65.
Meisters, G. H.
1961.
On the equation 𝑎𝑥-𝑥𝑏=𝑐 in division rings.
Proceedings of the American Mathematical Society,
Vol. 12,
Issue. 3,
p.
428.
Kowol, G.
1984.
Embeddings of universal algebras into simple ones.
Algebra Universalis,
Vol. 19,
Issue. 1,
p.
83.
Lawrence, J.
and
Simons, G. E.
1989.
Equations in Division Rings—A Survey.
The American Mathematical Monthly,
Vol. 96,
Issue. 3,
p.
220.
Magnus, John
1994.
Lie ideals closed under non-lie polynomials.
Communications in Algebra,
Vol. 22,
Issue. 13,
p.
5117.
Kharchenko, V.K.
1996.
Vol. 1,
Issue. ,
p.
761.
Kepka, Tomáš
Korbelář, Miroslav
and
Landsmann, Günter
2023.
Congruence-simple semirings without nilpotent elements.
Journal of Algebra and Its Applications,
Vol. 22,
Issue. 09,
Kala, Vítězslav
Kepka, Tomáš
and
Korbelář, Miroslav
2024.
Congruence-simple matrix semirings.
International Journal of Algebra and Computation,
Vol. 34,
Issue. 03,
p.
407.