Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
HEIL, E.
and
MARTINI, H.
1993.
Handbook of Convex Geometry.
p.
347.
Makai, E.
and
Martini, H.
1996.
The cross-section body, plane sections of convex bodies and approximation of convex bodies, I.
Geometriae Dedicata,
Vol. 63,
Issue. 3,
p.
267.
Böröczky, K.
Makai, E.
Meyer, M.
and
Reisner, S.
2013.
On the volume product of planar polar convex bodies — Lower estimates with stability.
Studia Scientiarum Mathematicarum Hungarica,
Vol. 50,
Issue. 2,
p.
159.


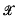 be a plane bounded convex set whose width in the direction φ is
be a plane bounded convex set whose width in the direction φ is 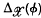 . It has been shown by L. A. Santaló that
. It has been shown by L. A. Santaló that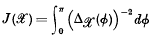
 and that equality characterizes triangles. In this note Santaló's conjecture is shown to be true.
and that equality characterizes triangles. In this note Santaló's conjecture is shown to be true.