Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Conrey, Brian
1998.
Selected Papers of Norman Levinson.
p.
306.
Conrey, J. Brian
2001.
Mathematics Unlimited — 2001 and Beyond.
p.
331.
Fyodorov, Yan V.
and
Strahov, Eugene
2002.
Characteristic polynomials of random Hermitian matrices and Duistermaat–Heckman localisation on non-compact Kähler manifolds.
Nuclear Physics B,
Vol. 630,
Issue. 3,
p.
453.
Conrey, J. Brian
2006.
Frontiers in Number Theory, Physics, and Geometry I.
p.
107.
Conrey, J. B.
and
Snaith, N. C.
2007.
Applications of theL-functions ratios conjectures.
Proceedings of the London Mathematical Society,
Vol. 94,
Issue. 3,
p.
594.
Feng, Shaoji
2012.
Zeros of the Riemann zeta function on the critical line.
Journal of Number Theory,
Vol. 132,
Issue. 4,
p.
511.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
307.
Rodgers, Brad
2015.
The Covariance of Almost-Primes in 𝔽q[T].
International Mathematics Research Notices,
Vol. 2015,
Issue. 14,
p.
5976.
Baluyot, Siegfred Alan C.
2016.
On the zeros of Riemann's zeta-function on the critical line.
Journal of Number Theory,
Vol. 165,
Issue. ,
p.
203.
Bettin, Sandro
and
Gonek, Steven M.
2017.
THE CONJECTURE IMPLIES THE RIEMANN HYPOTHESIS.
Mathematika,
Vol. 63,
Issue. 1,
p.
29.
Conrey, Brian
and
Keating, Jonathan P
2017.
Averages of ratios of the Riemann zeta-function and correlations of divisor sums.
Nonlinearity,
Vol. 30,
Issue. 10,
p.
R67.
Pratt, Kyle
Robles, Nicolas
Zaharescu, Alexandru
and
Zeindler, Dirk
2020.
More than five-twelfths of the zeros of $$\zeta $$ are on the critical line.
Research in the Mathematical Sciences,
Vol. 7,
Issue. 1,
Chirre, Andrés
2022.
A note on the mean values of the derivatives of 𝜁’/𝜁.
Proceedings of the American Mathematical Society,
Vol. 150,
Issue. 8,
p.
3253.
Bui, Hung
Florea, Alexandra
and
Keating, Jonathan
2023.
The Ratios Conjecture and upper bounds for negative moments of 𝐿-functions over function fields.
Transactions of the American Mathematical Society,
Vol. 376,
Issue. 6,
p.
4453.
Das, Mithun Kumar
and
Pujahari, Sudhir
2024.
Mean of the product of derivatives of Hardy's Z-function with Dirichlet polynomial.
Journal of Number Theory,
Vol. 258,
Issue. ,
p.
334.
Rodgers, Brad
2024.
Arithmetic Consequences of the GUE Conjecture for Zeta Zeros.
Michigan Mathematical Journal,
Vol. 74,
Issue. 5,
Gao, Peng
and
Zhao, Liangyi
2024.
Ratios conjecture for quadratic twists of modular L-functions.
Research in Number Theory,
Vol. 10,
Issue. 3,
Bui, Hung M.
and
Florea, Alexandra
2024.
Negative moments of the Riemann zeta-function.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 0,
Issue. 0,
Čech, Martin
2024.
The ratios conjecture for real Dirichlet characters and multiple Dirichlet series.
Transactions of the American Mathematical Society,
Rodgers, Brad
and
Vallabhaneni, Harshith Sai
2024.
Autocorrelations of characteristic polynomials for the Alternative Circular Unitary Ensemble.
Glasgow Mathematical Journal,
Vol. 66,
Issue. 1,
p.
51.
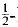 -line, where
-line, where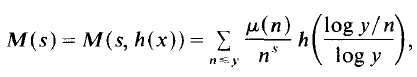
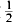 yields κ > 0·3562, having 0 < θ <
yields κ > 0·3562, having 0 < θ <  gives κ > 0·40219, and it is necessary to have θ > 0·165 in order to obtain a positive lower bound for κ. At present, it is known that the asymptotic formula remains valid for 0 < θ <
gives κ > 0·40219, and it is necessary to have θ > 0·165 in order to obtain a positive lower bound for κ. At present, it is known that the asymptotic formula remains valid for 0 < θ <  , this is due to Conrey. Without assuming the Riemann Hypothesis, Levinson's method provides the only known way of obtaining a positive lower bound for κ.
, this is due to Conrey. Without assuming the Riemann Hypothesis, Levinson's method provides the only known way of obtaining a positive lower bound for κ.