Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-23T18:09:27.211Z Has data issue: false hasContentIssue false
  • English
  • Français

A LOCAL UNIQUENESS THEOREM FOR MINIMIZERS OF PETTY’S CONJECTURED PROJECTION INEQUALITY

Part of: Classical differential geometry General convexity

Published online by Cambridge University Press:  24 January 2018

Mohammad N. Ivaki
Mohammad N. Ivaki*
Affiliation:
Department of Mathematics, University of Toronto, Ontario, M5S 2E4, Canada email [email protected]
Get access

Abstract

Employing the inverse function theorem on Banach spaces, we prove that in a 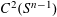$C^{2}(S^{n-1})$-neighborhood of the unit ball, the only solutions of $\unicode[STIX]{x1D6F1}^{2}K=cK$ are origin-centered ellipsoids. Here 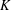$K$ is an $n$-dimensional convex body, $\unicode[STIX]{x1D6F1}K$ is the projection body of $K$ and 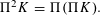$\unicode[STIX]{x1D6F1}^{2}K=\unicode[STIX]{x1D6F1}(\unicode[STIX]{x1D6F1}K).$

MSC classification

Primary: 52A20: Convex sets in $n$ dimensions (including convex hypersurfaces) 53A15: Affine differential geometry
Type
Research Article
Information
Mathematika , Volume 64 , Issue 1 , 2018 , pp. 1 - 19
Copyright
Copyright © University College London 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andrews, B., Entropy inequalities for evolving hypersurfaces. Comm. Anal. Geom. 2 1994, 53–64.CrossRefGoogle Scholar
Bonnesen, T. and Fenchel, W., Theorie der konvexen Körper, Springer (Berlin, 1934).Google Scholar
Fish, A., Nazarov, F., Ryabogin, D. and Zvavitch, A., The unit ball is an attractor of the intersection body operator. Adv. Math. 226 2011, 2629–2642.CrossRefGoogle Scholar
Gardner, R., Geometric Tomography, Vol. 6, Cambridge University Press (Cambridge, 2006).CrossRefGoogle Scholar
Goodey, P. R. and Groemer, H., Stability results for first order projection bodies. Proc. Amer. Math. Soc. 109 1990, 1103–1114.CrossRefGoogle Scholar
Goodey, P. R. and Weil, W., Centrally symmetric convex bodies and the spherical Radon transform. J. Differential Geom. 5 1992, 675–688.Google Scholar
Groemer, H., Geometric Applications of Fourier Series and Spherical Harmonics, Vol. 61, Cambridge University Press (New York, 1996).CrossRefGoogle Scholar
Hamilton, R. S., The inverse function theorem of Nash and Moser. Bull. Amer. Math. Soc. (N.S.) 7 1982, 65–222.CrossRefGoogle Scholar
Helgason, S., Integral Geometry and Radon Transforms, Springer (New York, 2011).Google Scholar
Ludwig, M., Projection bodies and valuations. Adv. Math. 172 2002, 158–168.CrossRefGoogle Scholar
Ludwig, M., Minkowski valuations. Trans. Amer. Math. Soc. 357 2005, 4191–4213.CrossRefGoogle Scholar
Lutwak, E., On a conjectured projection inequality of Petty. Contemp. Math. 113 1990, 171–182.CrossRefGoogle Scholar
Lutwak, E., On quermassintegrals of mixed projection bodies. Geom. Dedicata 33 1990, 51–58.CrossRefGoogle Scholar
Lutwak, E., Selected affine isoperimetric inequalities. In Handbook of Convex Geometry (eds Gruber, P. M. and Wills, J. M.), North-Holland (Amsterdam, 1993), 151–176.CrossRefGoogle Scholar
Martinez-Maure, Y., Hedgehogs and zonoids. Adv. Math. 158 2001, 1–17.CrossRefGoogle Scholar
Petty, C. M., Projection bodies. In Proc. Colloq. Convexity (Copenhagen, 1965) (ed. Fenchel, W.), Københavns Universitet Matematiske Institut (Copenhagen, 1967), 234–241.Google Scholar
Petty, C. M., Isoperimetric problems. In Proc. Conf. Convexity and Combinatorial Geometry (University of Oklahoma, 1971), University of Oklahoma (Norman, OK, 1972), 26–41.Google Scholar
Saroglou, C. and Zvavitch, A., Iterations of the projection body operator and a remark on Petty’s conjectured projection inequality. J. Funct. Anal. 272 2017, 613–630.CrossRefGoogle Scholar
Schneider, R., Convex Bodies: the Brunn–Minkowski Theory, Vol. 151, 2nd edn., Cambridge University Press (New York, 2013).CrossRefGoogle Scholar
Simon, L., Non-linear evolution equations, with applications to geometric problems. Ann. of Math. (2) 118 1983, 525–571.CrossRefGoogle Scholar
Strichartz, R. S., L p estimates for Radon transforms in Euclidean and non-Euclidean spaces. Duke Math. J. 48 1981, 699–727.CrossRefGoogle Scholar
Weil, W., Über die Projektionenkörper konvexer Polytope. Arch. Math. 22 1971, 664–672.CrossRefGoogle Scholar