Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-23T19:02:57.905Z Has data issue: false hasContentIssue false
  • English
  • Français

LARGE MOMENTS AND EXTREME VALUES OF CLASS NUMBERS OF INDEFINITE BINARY QUADRATIC FORMS

Part of: Forms and linear algebraic groups

Published online by Cambridge University Press:  20 April 2017

Youness Lamzouri
Youness Lamzouri*
Affiliation:
Department of Mathematics and Statistics, York University, 4700 Keele Street, Toronto, ON, M3J1P3, Canada email [email protected]
Get access

Abstract

Let $h(d)$ be the class number of indefinite binary quadratic forms of discriminant 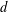$d$ and let 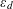$\unicode[STIX]{x1D700}_{d}$ be the corresponding fundamental unit. In this paper, we obtain an asymptotic formula for the 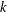$k$th moment of 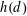$h(d)$ over positive discriminants 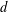$d$ with 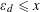$\unicode[STIX]{x1D700}_{d}\leqslant x$, uniformly for real numbers 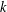$k$ in the range 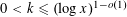$0<k\leqslant (\log x)^{1-o(1)}$. This improves upon the work of Raulf, who obtained such an asymptotic for a fixed positive integer 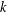$k$. We also investigate the distribution of large values of 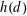$h(d)$ when the discriminants 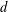$d$ are ordered according to the size of their fundamental units $\unicode[STIX]{x1D700}_{d}$. In particular, we show that the tail of this distribution has the same shape as that of class numbers of imaginary quadratic fields ordered by the size of their discriminants. As an application of these results, we prove that there are many positive discriminants 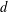$d$ with class number 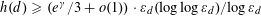$h(d)\geqslant (e^{\unicode[STIX]{x1D6FE}}/3+o(1))\cdot \unicode[STIX]{x1D700}_{d}(\log \log \unicode[STIX]{x1D700}_{d})/\log \,\unicode[STIX]{x1D700}_{d}$, a bound that we believe is best possible. We also obtain an upper bound for 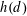$h(d)$ that is twice as large, assuming the generalized Riemann hypothesis.

MSC classification

Primary: 11E41: Class numbers of quadratic and Hermitian forms
Type
Research Article
Information
Mathematika , Volume 63 , Issue 2 , 2017 , pp. 564 - 586
Copyright
Copyright © University College London 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Dahl, A. and Lamzouri, Y., The distribution of class numbers in a special family of real quadratic fields. Trans. Amer. Math. Soc. (to appear).Google Scholar
Fouvry, E. and Klüners, J., On the negative Pell equation. Ann. of Math. (2) 172(3) 2010, 2035–2104.CrossRefGoogle Scholar
Gauss, C. F., Disquisitiones Arithmeticae, Yale University Press (New Haven, CT, 1966), 304.Google Scholar
Granville, A. and Soundararajan, K., The distribution of values of L (1, 𝜒 d ). Geom. Funct. Anal. 13(5) 2003, 992–1028.Google Scholar
Heath-Brown, D. R., A mean value estimate for real character sums. Acta Arith. 72(3) 1995, 235–275.Google Scholar
Hooley, C., On the Pellian equation and the class number of indefinite binary quadratic forms. J. reine angew. Math. 353 1984, 98–131.Google Scholar
Lamzouri, Y., Distribution of values of L-functions at the edge of the critical strip. Proc. Lond. Math. Soc. (3) 100(3) 2010, 835–863.CrossRefGoogle Scholar
Lamzouri, Y., Extreme values of argL (1, 𝜒). Acta Arith. 146(4) 2011, 335–354.CrossRefGoogle Scholar
Littlewood, J. E., On the class number of the corpus P (√-k). Proc. Lond. Math. Soc. (3) 27 1928, 358–372.Google Scholar
Raulf, N., Asymptotics of class numbers for progressions and for fundamental discriminants. Forum Math. 21(2) 2009, 221–257.Google Scholar
Raulf, N., Limit distribution of class numbers for discriminants in progressions and fundamental discriminants. Int. J. Number Theory 12(5) 2016, 1237–1258.CrossRefGoogle Scholar
Sarnak, P., Class numbers of indefinite binary quadratic forms. J. Number Theory 15 1982, 229–247.Google Scholar
Sarnak, P., Class numbers of indefinite binary quadratic forms II. J. Number Theory 21 1985, 333–346.Google Scholar
Siegel, C. L., The average measure of quadratic forms with given determinant and signature. Ann. of Math. (2) 45 1944, 667–685.Google Scholar