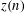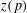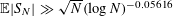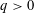Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Brevig, Ole Fredrik
and
Perfekt, Karl-Mikael
2016.
Weak Product Spaces of Dirichlet Series.
Integral Equations and Operator Theory,
Vol. 86,
Issue. 4,
p.
453.
Heap, Winston P.
and
Lindqvist, Sofia
2016.
Moments of Random Multiplicative Functions and Truncated Characteristic Polynomials.
The Quarterly Journal of Mathematics,
Bondarenko, Andriy
Brevig, Ole Fredrik
Saksman, Eero
Seip, Kristian
and
Zhao, Jing
2018.
Pseudomoments of the Riemann zeta function.
Bulletin of the London Mathematical Society,
Vol. 50,
Issue. 4,
p.
709.
HARPER, ADAM J.
2020.
MOMENTS OF RANDOM MULTIPLICATIVE FUNCTIONS, I: LOW MOMENTS, BETTER THAN SQUAREROOT CANCELLATION, AND CRITICAL MULTIPLICATIVE CHAOS.
Forum of Mathematics, Pi,
Vol. 8,
Issue. ,
Mahatab, Kamalakshya
2020.
Sixth norm of a Steinhaus chaos.
International Journal of Number Theory,
Vol. 16,
Issue. 06,
p.
1295.
Queffélec, Hervé
and
Queffélec, Martine
2020.
Diophantine Approximation and Dirichlet Series.
Vol. 80,
Issue. ,
p.
145.
de la Bretèche, Régis
Munsch, Marc
and
Tenenbaum, Gérald
2021.
Small Gál sums and applications.
Journal of the London Mathematical Society,
Vol. 103,
Issue. 1,
p.
336.
Brevig, Ole Fredrik
Ortega-Cerdà, Joaquim
and
Seip, Kristian
2021.
Idempotent Fourier multipliers acting contractively on $$H^p$$ spaces.
Geometric and Functional Analysis,
Vol. 31,
Issue. 6,
p.
1377.
Benatar, Jacques
Nishry, Alon
and
Rodgers, Brad
2022.
Moments of polynomials with random multiplicative coefficients.
Mathematika,
Vol. 68,
Issue. 1,
p.
191.
Defant, A.
Galicer, D.
Mansilla, M.
Mastyło, M.
and
Muro, S.
2024.
Projection constants for spaces of Dirichlet polynomials.
Mathematische Annalen,
Vol. 390,
Issue. 2,
p.
1885.
Xu, Max Wenqiang
2024.
Better than square-root cancellation for random multiplicative functions.
Transactions of the American Mathematical Society, Series B,
Vol. 11,
Issue. 14,
p.
482.
Aymone, Marco
2024.
Sign changes of the partial sums of a random multiplicative function II.
Comptes Rendus. Mathématique,
Vol. 362,
Issue. G8,
p.
895.