Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Reiner, Irving
1975.
Representations of Algebras.
Vol. 488,
Issue. ,
p.
253.
Fröhlich, A
1976.
A normal integral basis theorem.
Journal of Algebra,
Vol. 39,
Issue. 1,
p.
131.
Wilson, S.M.J
1977.
Reduced norms in the K-theory of orders.
Journal of Algebra,
Vol. 46,
Issue. 1,
p.
1.
Taylor, M.J
1978.
Locally free classgroups of groups of prime power order.
Journal of Algebra,
Vol. 50,
Issue. 2,
p.
463.
Stein, Michael R.
1978.
Whitehead groups of finite groups.
Bulletin of the American Mathematical Society,
Vol. 84,
Issue. 2,
p.
201.
Oliver, Robert
1978.
Subgroups generating D(ZG).
Journal of Algebra,
Vol. 55,
Issue. 1,
p.
43.
Magurn, Bruce
1978.
SK1 of dihedral groups.
Journal of Algebra,
Vol. 51,
Issue. 2,
p.
399.
Mislin, G.
and
Varadarajan, K.
1979.
The finiteness obstructions for nilpotent spaces lie inD(? ?).
Inventiones Mathematicae,
Vol. 53,
Issue. 2,
p.
185.
Hambleton, Ian
and
Milgram, R. James
1979.
Algebraic Topology Waterloo 1978.
Vol. 741,
Issue. ,
p.
73.
Carlsson, G.
and
Milgram, R. James
1979.
Algebraic Topology Waterloo 1978.
Vol. 741,
Issue. ,
p.
1.
Endo, Shizuo
and
Miyata, Takehiko
1980.
On the class groups of dihedral groups.
Journal of Algebra,
Vol. 63,
Issue. 2,
p.
548.
Hambleton, Ian
and
Milgram, R. James
1980.
The surgery obstruction groups for finite 2-groups.
Inventiones Mathematicae,
Vol. 61,
Issue. 1,
p.
33.
Mislin, Guido
1980.
The geometric realization of Wall obstructions by nilpotent and simple spaces.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 87,
Issue. 2,
p.
199.
Anderson, Douglas R.
and
Hambleton, Ian
1980.
Balanced splittings of Semi-free actions of finite groups on homotopy spheres.
Commentarii Mathematici Helvetici,
Vol. 55,
Issue. 1,
p.
130.
Fröhlich, A.
1980.
Galois module structure and rootnumbers for quaternion extensions of degree 2n.
Journal of Number Theory,
Vol. 12,
Issue. 4,
p.
499.
Miyata, Takehiko
1980.
A normal integral basis theorem for dihedral groups.
Tohoku Mathematical Journal,
Vol. 32,
Issue. 1,
Wilson, S. M. J.
1981.
Integral Representations and Applications.
Vol. 882,
Issue. ,
p.
247.
Keating, M. E.
1982.
Algebraic K-Theory.
Vol. 966,
Issue. ,
p.
122.
Theohari-Apostolidi, Th
1985.
On integral representations of twisted group rings.
Journal of Algebra,
Vol. 93,
Issue. 2,
p.
413.
Milgram, R. James
1985.
Algebraic and Geometric Topology.
Vol. 1126,
Issue. ,
p.
127.


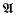 be a = ℤ-order in
be a = ℤ-order in  locally isomorphic to
locally isomorphic to  as all ℤΓ projectives are locally free [12].)
as all ℤΓ projectives are locally free [12].)