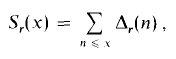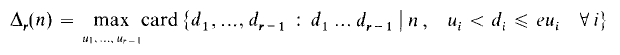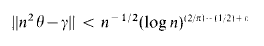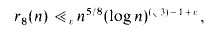Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Maier, H.
and
Tenenbaum, G.
1984.
On the set of divisors of an integer.
Inventiones Mathematicae,
Vol. 76,
Issue. 1,
p.
121.
Tenebaum, Gérald
1985.
Sur la concentration moyenne des diviseurs.
Commentarii Mathematici Helvetici,
Vol. 60,
Issue. 1,
p.
411.
Hall, R.R
and
Tenenbaum, G
1986.
Les ensembles de multiples et la densité divisorielle.
Journal of Number Theory,
Vol. 22,
Issue. 3,
p.
308.
Hall, R. R.
1990.
Uniform upper bounds for the complex divisor function.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 108,
Issue. 3,
p.
421.
Tuckfeld, Manon
1997.
Orte des Politischen.
p.
1.
Ford, Kevin
Green, Ben
and
Koukoulopoulos, Dimitris
2023.
Equal sums in random sets and the concentration of divisors.
Inventiones mathematicae,
Vol. 232,
Issue. 3,
p.
1027.
Koukoulopoulos, Dimitris
and
Tao, Terence
2023.
An upper bound on the mean value of the Erdős–Hooley Delta function.
Proceedings of the London Mathematical Society,
Vol. 127,
Issue. 6,
p.
1865.
Ford, Kevin
Koukoulopoulos, Dimitris
and
Tao, Terence
2024.
A lower bound on the mean value of the Erdős–Hooley Delta function.
Proceedings of the London Mathematical Society,
Vol. 129,
Issue. 1,