Integral geometry is the study of measures of sets of geometric figures. Commonly a measure of this sort is an integral of a density or differential form; the density is determined by the type of figure, but is independent of the particular set of such figures to which the measure is assigned. As one of the simplest examples, the area of a plane convex point set K is the integral over K of the density dx dy for points with Cartesian co-ordinates x, y. But when we assign a Hausdorff linear measure to the set of boundary points of K, we obtain a measure of quite another sort. This is representable as a Stieltjes integral of arc length density; here the density depends on the choice of K. The examples suggest examining measures for other sets of figures, where each such set 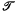is made up of all those figures from a certain class which support, in some sense, a convex body. Further, the examples lead us to expect that measures of this kind will appear as integrals of densities which may depend on the choices of 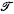. Here we treat a question of the type just described: to determine a measure for sets of q–flats which support a convex body.