Hostname: page-component-5cf477f64f-mgq6s Total loading time: 0 Render date: 2025-04-07T16:14:30.142Z Has data issue: false hasContentIssue false
  • English
  • Français

A LIMITING CASE OF ULTRASYMMETRIC SPACES

Part of: Linear function spaces and their duals Special classes of linear operators Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  01 June 2016

Pedro Fernández-Martínez  and
Teresa Signes
Pedro Fernández-Martínez
Affiliation:
Departamento de Matemáticas, Facultad de Matemáticas, Universidad de Murcia, Campus de Espinardo, 30071 Espinardo (Murcia), Spain email [email protected]
Teresa Signes
Affiliation:
Departamento de Matemáticas, Facultad de Matemáticas, Universidad de Murcia, Campus de Espinardo, 30071 Espinardo (Murcia), Spain email [email protected]
Get access

Abstract

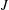We study ultrasymmetric spaces in the case in which the fundamental function belongs to a limiting class of quasiconcave functions. In the process, we study limiting cases of $J$ interpolation spaces and establish new $J$ – 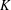$K$ identities as well as a reiteration theorem for these limiting interpolation methods.

MSC classification

Primary: 46B70: Interpolation between normed linear spaces 46E30: Spaces of measurable functions ($L^p$-spaces, Orlicz spaces, Köthe function spaces, Lorentz spaces, rearrangement invariant spaces, ideal spaces, etc.) 47B38: Operators on function spaces (general)
Type
Research Article
Information
Mathematika , Volume 62 , Issue 3 , 2016 , pp. 929 - 948
Copyright
Copyright © University College London 2016 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Astashkin, S. and Maligranda, L., Ultrasymmetric Orlicz spaces. J. Math. Anal. Appl. 1 2008, 273–285.Google Scholar
Bennett, C. and Rudnick, K., On Lorentz–Zygmund spaces. Dissertationes Math. (Rozprawy Mat.) 175 1980, 1–67.Google Scholar
Bennett, C. and Sharpley, R., Interpolation of operators. In Pure and Applied Mathematics, Vol. 129, Academic Press, Inc. (Boston, MA, 1988).Google Scholar
Bergh, J. and Löfström, J., Interpolation Spaces. An Introduction, Springer (Berlin–Heidelberg–New York, 1976).Google Scholar
Brézis, H. and Wainger, S., A note on limiting cases of Sobolev embeddings and convolution inequalities. Comm. Partial Differential Equations 5(7) 1980, 773–789.Google Scholar
Brudnyĭ, Yu. A. and Krugljak, N. Ya., Interpolation Functors and Interpolation Spaces, Vol. I (North-Holland Mathematical Library 47 ), North-Holland Publishing (Amsterdam, 1991).Google Scholar
Cobos, F., Fernández-Cabrera, L. M., Kühn, T. and Ullrich, T., On an extreme class of real interpolation spaces. J. Funct. Anal. 256 2009, 2321–2366.Google Scholar
Cobos, F., Fernández-Cabrera, L. M. and Mastylo, M., Abstract limit J -spaces. J. Lond. Math. Soc. (2) 82 2010, 501–525.CrossRefGoogle Scholar
Cobos, F., Fernández-Cabrera, L. M. and Silvestre, P., Limiting J-spaces for general couples. Z. Anal. Anwend. 32(1) 2013, 83–101.Google Scholar
Cobos, F. and Kühn, T., Equivalence of K- and J-methods for limiting real interpolation spaces. J. Funct. Anal. 216 2011, 3696–3722.Google Scholar
Cobos, F. and Segurado, A., Some reiteration formulae for limiting real methods. J. Math. Anal. Appl. 411 2014, 405–421.Google Scholar
Cobos, F. and Segurado, A., Description of logarithmic interpolation spaces by means of theJ-functional and applications. J. Funct. Anal. 268 2015, 2906–2945.Google Scholar
Cwikel, M. and Pustylnik, E., Sobolev type embeddings in the limiting case. J. Fourier Anal. Appl. 4(4–5) 1998, 433–446.Google Scholar
Cwikel, M. and Pustylnik, E., Weak type interpolation near ‘endpoint’ spaces. J. Funct. Anal. 171 2000, 235–277.CrossRefGoogle Scholar
Edmunds, D. E., Gurka, P. and Opic, B., Double exponential integrability of convolution operators in generalized Lorentz–Zygmund spaces. Indiana Univ. Math. J. 44 1995, 19–43.Google Scholar
Edmunds, D. E., Gurka, P. and Opic, B., Sharpness of embeddings in logarithmic Bessel-potential spaces. Proc. Roy. Soc. Edinburgh 126A 1996, 995–1009.Google Scholar
Edmunds, D. E., Gurka, P. and Opic, B., On embeddings in logarithmic Bessel potential spaces. J. Funct. Anal. 1 1997, 116–150.Google Scholar
Edmunds, D. E., Gurka, P. and Opic, B., Norms of embeddings of logarithmic Bessel potential spaces. Proc. Amer. Math. Soc. 126(8) 1998, 2417–2425.Google Scholar
Edmunds, D. E., Gurka, P. and Opic, B., Optimality of embeddings of logarithmic Bessel potential spaces. Q. J. Math. 51(2) 2000, 185–209.Google Scholar
Edmunds, D. E., Gurka, P. and Opic, B., Compact and continuous embeddings of logarithmic Bessel potential spaces. Stud. Math. 168(3) 2005, 229–250.Google Scholar
Edmunds, D. E., Gurka, P. and Opic, B., Non-compact and sharp embeddings of logarithmic Bessel potential spaces into Hölder-type spaces. Z. Anal. Anwend. 25(1) 2006, 73–80.Google Scholar
Edmunds, D. E., Kerman, R. and Pick, L., Optimal Sobolev imbeddings involving rearrangement-invariant quasinorms. J. Funct. Anal. 170(2) 2000, 307–355.Google Scholar
Fernández-Martínez, P. and Signes, T., Real interpolation with slowly varying functions and symmetric spaces. Q. J. Math. 63(1) 2012, 133–164.Google Scholar
Fernández-Martínez, P. and Signes, T., Reiteration theorems with extreme values of parameters. Ark. Mat. 52(2) 2014, 227–256.Google Scholar
Fernández-Martínez, P. and Signes, T., Limit cases of reiteration theorems. Math. Nachr. 288(1) 2015, 25–47.Google Scholar
Hansson, K., Imbedding theorems of Sobolev type in potential theory. Math. Scand. 45(1) 1979, 77–102.Google Scholar
Kerman, R. and Pick, L., Optimal Sobolev imbeddings. Forum Math. 18(4) 2006, 535–570.Google Scholar
Kerman, R. and Pick, L., Compactness of Sobolev imbeddings involving rearrangement invariant spaces. Stud. Math. 2(186) 2008, 127–160.CrossRefGoogle Scholar
Kerman, R. and Pick, L., Optimal Sobolev imbedding spaces. Studia Math. 192(3) 2009, 195–217.Google Scholar
Kerman, R. and Pick, L., Explicit formulas for optimal rearrangement-invariant norms in Sobolev imbedding inequalities. Studia Math. 206(2) 2011, 97–119.Google Scholar
Kreĭn, S. G., Petunin, Ju. I. and Semenov, E. M., Interpolation of linear operators. In Translations of Mathematical Monographs, Vol. 54, American Mathematical Society (Providence, RI, 1982).Google Scholar
Lindenstrauss, J. and Tzafriri, L., Classical Banach Spaces II, Springer (New York, 1979).Google Scholar
Maz’ya, V., Sobolev Spaces with Applications to Elliptic Partial Differential Equations. Second, Revised and Augmented Edition (Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences] 342 ), Springer (Heidelberg, 2011).Google Scholar
Neves, J. S., Lorentz-Karamata spaces, Bessel and Riesz potentials and embeddings. Dissertationes Math. (Rozprawy Mat.) 405 2002, 1–46.Google Scholar
Opic, B. and Pick, L., On generalized Lorentz–Zygmund spaces. Math. Inequal. Appl. 2 1999, 391–467.Google Scholar
Pietsch, A., Approximation spaces. J. Approx. Theory 32(2) 1981, 115–134.Google Scholar
Pustylnik, E., Ultrasymmetric Spaces. J. Lond. Math. Soc. (2) 68(2) 2003, 165–182.Google Scholar
Pustylnik, E., Sobolev type inequalities in ultrasymmetric spaces with applications to Orlicz-Sobolev embeddings. J. Funct. Spaces Appl. 3(2) 2005, 18–208.CrossRefGoogle Scholar
Pustylnik, E., Ultrasymmetric sequence spaces in approximation theory. Collect. Math. 57(3) 2006, 257–532.Google Scholar
Pustylnik, E. and Signes, T., Orbits and co-orbits of ultrasymmetric spaces in weak interpolation. J. Math. Anal. Appl. 339 2008, 938–953.Google Scholar