Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Kechris, A. S.
Louveau, A.
and
Woodin, W. H.
1987.
The structure of 𝜎-ideals of compact sets.
Transactions of the American Mathematical Society,
Vol. 301,
Issue. 1,
p.
263.
Kechris, Alexander S.
1991.
Hereditary properties of the class of closed sets of uniqueness for trigonometric series.
Israel Journal of Mathematics,
Vol. 73,
Issue. 2,
p.
189.
Kechris, A. S.
and
Louveau, A.
1992.
Descriptive set theory and harmonic analysis.
Journal of Symbolic Logic,
Vol. 57,
Issue. 2,
p.
413.
Becker, Howard
1992.
Set Theory of the Continuum.
Vol. 26,
Issue. ,
p.
1.
Cooke, Roger
1993.
Uniqueness of trigonometric series and descriptive set theory, 1870–1985.
Archive for History of Exact Sciences,
Vol. 45,
Issue. 4,
p.
281.
Matheron, Étienne
and
Zelený, Miroslav
2007.
Descriptive set Theory of Families of Small Sets.
Bulletin of Symbolic Logic,
Vol. 13,
Issue. 4,
p.
482.
2011.
A Probability Metrics Approach to Financial Risk Measures.
p.
7.
Rachev, Svetlozar T.
Klebanov, Lev B.
Stoyanov, Stoyan V.
and
Fabozzi, Frank J.
2013.
The Methods of Distances in the Theory of Probability and Statistics.
p.
11.


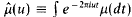 its Fourier-Stieltjes transform. We define ℛ to be the set of μ with
its Fourier-Stieltjes transform. We define ℛ to be the set of μ with 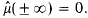 When μ ∈ ℛ and φ is of class
When μ ∈ ℛ and φ is of class 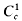 (continuously differentiable of compact support), the identity
(continuously differentiable of compact support), the identity 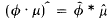 shows that θ · μ ∈ ℛ.
shows that θ · μ ∈ ℛ.