Published online by Cambridge University Press: 26 February 2010
A compact space K is said to have the Namioka Property, or to belong to the class 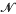 *, if, for every Baire space B and every separately continuous function Ψ:B × K → ℝ, there is dense
*, if, for every Baire space B and every separately continuous function Ψ:B × K → ℝ, there is dense  δ subset H of B such that Ψ is (jointly) continuous at all points of H × K. Although the terminology is more recent, the idea of looking at properties of this kind goes back to Namioka's paper [6] on separate and joint continuity. Talagrand [8] gave the first example of a compact space that is not in
δ subset H of B such that Ψ is (jointly) continuous at all points of H × K. Although the terminology is more recent, the idea of looking at properties of this kind goes back to Namioka's paper [6] on separate and joint continuity. Talagrand [8] gave the first example of a compact space that is not in 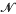 * and it is now known [4] that there are even examples of scattered compact spaces that are not in
* and it is now known [4] that there are even examples of scattered compact spaces that are not in 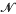 *. On the other hand, many good classes of compact spaces have been shown to be contained in
*. On the other hand, many good classes of compact spaces have been shown to be contained in 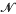 *, probably the most general being the class of continuous images of Valdivia compacts [2]. The aim of this note is to prove the following stability result: a compact space which is a countable union of closed subsets with the Namioka Property does itself possess that property.
*, probably the most general being the class of continuous images of Valdivia compacts [2]. The aim of this note is to prove the following stability result: a compact space which is a countable union of closed subsets with the Namioka Property does itself possess that property.