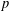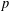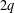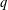Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Strzelecka, Marta
Strzelecki, Michal
and
Tkocz, Tomasz
2017.
On the convex infimum convolution inequality
with optimal cost function
.
Latin American Journal of Probability and Mathematical Statistics,
Vol. 14,
Issue. 1,
p.
903.
Strzelecka, Marta
2019.
Estimates of norms of log-concave random matrices with dependent entries.
Electronic Journal of Probability,
Vol. 24,
Issue. none,
Latała, Rafał
and
Strzelecka, Marta
2020.
Geometric Aspects of Functional Analysis.
Vol. 2266,
Issue. ,
p.
65.
Lata, Rafał
and
Świątkowski, Witold
2022.
Norms of randomized circulant matrices.
Electronic Journal of Probability,
Vol. 27,
Issue. none,
Latała, Rafał
2023.
High Dimensional Probability IX.
Vol. 80,
Issue. ,
p.
325.
Adamczak, Radosław
Prochno, Joscha
Strzelecka, Marta
and
Strzelecki, Michał
2024.
Norms of structured random matrices.
Mathematische Annalen,
Vol. 388,
Issue. 4,
p.
3463.
Latała, Rafał
and
Strzelecka, Marta
2024.
Chevet-type inequalities for subexponential Weibull variables and estimates for norms of random matrices.
Electronic Journal of Probability,
Vol. 29,
Issue. none,
Dai, Guozheng
and
Su, Zhonggen
2025.
Deviation inequalities for the spectral norm of structured random matrices.
Statistics & Probability Letters,
Vol. 221,
Issue. ,
p.
110378.
Meller, Rafał
2025.
Moments and tails of Lq-valued chaoses of order two based on independent variables with log-concave tails.
Electronic Journal of Probability,
Vol. 30,
Issue. none,
Latała, Rafał
and
Strzelecka, Marta
2025.
Operator ℓ → ℓ norms of random matrices with iid entries.
Journal of Functional Analysis,
Vol. 288,
Issue. 3,
p.
110720.