Hostname: page-component-669899f699-g7b4s Total loading time: 0 Render date: 2025-04-25T16:19:54.515Z Has data issue: false hasContentIssue false
  • English
  • Français

Wb-sober spaces and the core-coherence of dcpo models

Published online by Cambridge University Press:  06 November 2024

Chong Shen Open the ORCID record for Chong Shen [Opens in a new window]  and
Xinchao Zhao
Chong Shen*
Affiliation:
School of Science, Beijing University of Posts and Telecommunications, Beijing, China Key Laboratory of Mathematics and Information Networks (Beijing University of Posts and Telecommunications), Ministry of Education, Beijing, China
Xinchao Zhao
Affiliation:
School of Science, Beijing University of Posts and Telecommunications, Beijing, China Key Laboratory of Mathematics and Information Networks (Beijing University of Posts and Telecommunications), Ministry of Education, Beijing, China
*
Corresponding author: Chong Shen; Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

In this paper, we introduce a new class of 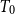$T_0$ spaces called wb-sober spaces, which is strictly larger than the class of open well-filtered spaces. Unlike open well-filtered spaces, wb-sober spaces are defined more intuitively by requiring certain special subsets, termed wb-irreducible closed sets, to have singleton closures. We establish several key results about these spaces, including (1) every open well-filtered space is wb-sober, but not vice versa; (2) every strongly core-coherent wb-sober space is open well-filtered; (3) a space is core-compact iff its irreducible closed sets are wb-irreducible, providing a characterization of core-compactness; (4) every core-compact wb-sober space is sober, thereby generalizing the Jia-Jung problem. In addition, we investigate the core-coherence of the Xi-Zhao model. We prove that a 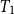$T_1$ space contains finite number of isolated points iff its Xi-Zhao model is core-coherent iff its Xi-Zhao model is strongly core-coherent. Based on this result, we then propose a general approach to constructing a non-routine open well-filtered but not well-filtered dcpo.

Keywords

Core-coherencecore-compact spaceopen well-filtered spaceposet modelScott topologywb-sober space
Type
Paper
Information
Mathematical Structures in Computer Science , Volume 34 , Issue 6 , June 2024 , pp. 529 - 550
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Chen, S. and Li, Q. (2022). On some topological properties of dcpo models of T 1 topological spaces. Results in Mathematics 77 (92) 1–14.CrossRefGoogle Scholar
Chen, S. and Li, Q. (2023). The Xi-Zhao model of T 1 spaces. Topology and Its Applications 333 108537.CrossRefGoogle Scholar
Engelking, R. (1989). General Topology, Sigma Series in Pure Mathematics vol. 6. Berlin: Heldermann Verlag.Google Scholar
Gierz, G., Hofmann, K., Keimel, K., Lawson, J., Mislove, M. and Scott, D. (2003) Continuous lattices and domains, Encyclopedia of Mathematics and Its Applications, vol. 93, Cambridge University Press.Google Scholar
Goubault-Larrecq, J. (2013). Non-Hausdorff Topology and Domain Theory, New York: Cambridge University Press.CrossRefGoogle Scholar
He, Q., Xi, X. and Zhao, D. (2019). Dcpo models of Choquet complete and Baire spaces. Results in Mathematics 74 87. https://doi.org/10.1007/s00025-019-1011-1.CrossRefGoogle Scholar
Heckmann, R. (1990). Power Domain Constructions. PhD thesis, Universität des Saarlandes.Google Scholar
Heckmann, R. (1991). An upper power domain construction in terms of strongly compact sets. Lecture Notes in Computer Science 598 272–293.CrossRefGoogle Scholar
Ho, W., Goubault-Larrecq, J., Jung, A. and Xi, X. (2018). The Ho-Zhao problem. Logical Methods in Computer Science 14 1–19.Google Scholar
Jia, X. (2018). Meet-continuity and locally compact sober dcpos. PhD thesis, University of Birmingham.Google Scholar
Jia, X., Jung, A. and Li, Q. (2016). A note on coherence of dcpos. Topology and Its Applications 209 235–238.CrossRefGoogle Scholar
Johnstone, P. T. (1981) Scott is not always sober. In: Banaschewski, B. and Hoffmann, R. E. (eds.) Continuous Lattices. Lecture Notes in Mathematics, vol. 871. Berlin, Heidelberg: Springer, pp. 282–283. https://doi.org/10.1007/BFb0089911.CrossRefGoogle Scholar
Kou, H. (2001) $\mathcal U_k$ -admitting dcpos need not be sober . In: K. Keimel, G. -Q. Zhang, Y.-M. Liu, and Y.-X. Chen (eds.) Domain and Processes, volume 1 of Semantic Structures in Computation. Kluwer Academic Publishers, pp. 41–50.Google Scholar
Lawson, J., Wu, G. and Xi, X. (2020). Well-filtered spaces, compactness, and the lower topology. Houston Journal of Mathematics 46 (1) 283–294.Google Scholar
Liu, B., Li, Q. and Wu, G. (2020). Well-filterifications of topological spaces. Topology and Its Applications 279 107245.CrossRefGoogle Scholar
Shen, C., Wu, G. and Zhao, D. (2019). Weak domain models of T 1 spaces. Electronic Notes in Theoretical Computer Science 345 219–232.CrossRefGoogle Scholar
Shen, C., Xi, X., Xu, X. and Zhao, D. (2020). On open well-filtered spaces. Logical Methods of Computational Science 16(4) 10.Google Scholar
Shen, C., Xi, X. and Zhao, D. (2022a). Further studies on open well-filtered spaces. In: Electronic Notes in Theoretical Informatics and Computer Science 2, Proceedings of ISDT 2022, arXiv:2211.16133v1 [math.GN].CrossRefGoogle Scholar
Shen, C., Xi, X. and Zhao, D. (2022b). The non-reflectivity of open well-filtered spaces via b-topology. Houston Journal of Mathematics 48 (4) 843–854.Google Scholar
Wu, G., Xi, X., Xu, X. and Zhao, D. (2020). Existence of well-filtered reflections of T 0 topological spaces. Topology and Its Applications 270 107044.CrossRefGoogle Scholar
Xi, X. and Lawson, J. (2017). On well-filtered spaces and ordered sets. Topology and Its Applications 228 139–144.CrossRefGoogle Scholar
Xi, X. and Zhao, D. (2017). Well-filtered spaces and their dcpo models. Mathematical Structures in Computer Science 27(4) 507–515.CrossRefGoogle Scholar
Xu, X. (2021). On H-sober spaces and H-sobrifications of T 0 spaces. Topology and Its Applications 289 107548.CrossRefGoogle Scholar
Xu, X., Shen, C., Xi, X. and Zhao, D. (2020a). First countability, ω-well-filtered spaces and reflections. Topology and Its Applications 279 107255.CrossRefGoogle Scholar
Xu, X., Shen, C., Xi, X. and Zhao, D. (2020b). On T 0 spaces determined by well-filtered spaces. Topology and Its Applications 282 107323.CrossRefGoogle Scholar
Xu, X., Shen, C., Xi, X., Zhao, D. and First-countability (2021). ω-Rudin spaces and well-filtered determined spaces. Topology and Its Applications 300 CrossRefGoogle Scholar
Zhao, D. (2009). Poset models of topological spaces . In: Proceeding of International Conference on Quantitative Logic and Quantification of Software Global–Link Publisher. 229–238.Google Scholar
Zhao, D. and Xi, X. (2018). Directed complete poset models of T 1 spaces. Mathematical Proceedings of the Cambridge Philosophical Society 164 125–134.CrossRefGoogle Scholar
Zhao, D., Xi, X. and Chen, Y. (2019). A new dcpo whose Scott topology is well-filtered but not sober. Topology and Its Applications 252 97–102.Google Scholar