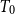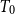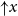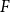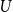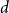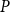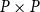1. Introduction
In his pioneering work in what has come to be called “domain theory,” which provides a mathematical foundation for the denotational semantics of programming languages, Dana Scott introduced a crucial
![]() $T_0$
-topology which came to be called the Scott topology. In domain theory and non-Hausdorff topology, we encounter numerous links between topology and order theory (cf. Abramsky and Jung Reference Abramsky and Jung1994; Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Goubault-Larrecq Reference Goubault-Larrecq2013). Sobriety is probably the most important and useful property of
$T_0$
-topology which came to be called the Scott topology. In domain theory and non-Hausdorff topology, we encounter numerous links between topology and order theory (cf. Abramsky and Jung Reference Abramsky and Jung1994; Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Goubault-Larrecq Reference Goubault-Larrecq2013). Sobriety is probably the most important and useful property of
![]() $T_0$
-spaces. The Hofmann-Mislove Theorem reveals a very distinct characterization for the sober spaces via open filters and illustrates the close relationship between domain theory and topology.
$T_0$
-spaces. The Hofmann-Mislove Theorem reveals a very distinct characterization for the sober spaces via open filters and illustrates the close relationship between domain theory and topology.
With the development of domain theory and non-Hausdorff topology, another two properties also emerged as the very useful and important properties for
![]() $T_0$
-spaces: the property of being a
$T_0$
-spaces: the property of being a
![]() $d$
-space and the well-filteredness (see Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Goubault-Larrecq Reference Goubault-Larrecq2013; Heckmann Reference Heckmann1992; Jia Reference Jia2018; Keimel and Lawson Reference Keimel and Lawson2009; Wyler Reference Wyler1981; Xu et al. Reference Xu, Shen, Xi and Zhao2020b; Xu and Zhao Reference Xu and Zhao2021). In order to uncover more finer links between
$d$
-space and the well-filteredness (see Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Goubault-Larrecq Reference Goubault-Larrecq2013; Heckmann Reference Heckmann1992; Jia Reference Jia2018; Keimel and Lawson Reference Keimel and Lawson2009; Wyler Reference Wyler1981; Xu et al. Reference Xu, Shen, Xi and Zhao2020b; Xu and Zhao Reference Xu and Zhao2021). In order to uncover more finer links between
![]() $d$
-spaces and well-filtered spaces, the notion of strong
$d$
-spaces and well-filtered spaces, the notion of strong
![]() $d$
-spaces has been introduced in Xu and Zhao (Reference Xu and Zhao2020).
$d$
-spaces has been introduced in Xu and Zhao (Reference Xu and Zhao2020).
It is worth noting that through other authors of Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003), another topology was added in domain theory, the Lawson topology, which was the join of the Scott topology and a third topology, called the lower topology in Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003) and other names elsewhere. The joining of the Scott and lower topology has proved a quite fruitful part of the overall theory and presented some important connections between domain theory and classical topology (which generally assumes the Hausdorff separation condition).
In this paper, we seek to extend this program to
![]() $T_0$
-spaces and explore much more general settings where one can fruitfully combine the study of a
$T_0$
-spaces and explore much more general settings where one can fruitfully combine the study of a
![]() $T_0$
-space with the lower topology and the topology generated by the two together.
$T_0$
-space with the lower topology and the topology generated by the two together.
Taking a mildly different point of view, one can regard the investigation from the point of view of bitopological spaces, triples
![]() $(X,\tau, \nu )$
where
$(X,\tau, \nu )$
where
![]() $\tau$
and
$\tau$
and
![]() $\nu$
are topologies on
$\nu$
are topologies on
![]() $X$
and morphisms are maps that are continuous in both topologies. If one is considering
$X$
and morphisms are maps that are continuous in both topologies. If one is considering
![]() $T_0$
-spaces, it is natural to consider order-dual topologies, topologies
$T_0$
-spaces, it is natural to consider order-dual topologies, topologies
![]() $\tau$
and
$\tau$
and
![]() $\nu$
for which the orders of specialization for
$\nu$
for which the orders of specialization for
![]() $\tau$
and
$\tau$
and
![]() $\nu$
are opposites (see, e.g., Xu Reference Xu2016b). In this setting, the join
$\nu$
are opposites (see, e.g., Xu Reference Xu2016b). In this setting, the join
![]() $\tau \bigvee \nu$
of the two topologies, the smallest topology containing both
$\tau \bigvee \nu$
of the two topologies, the smallest topology containing both
![]() $\tau$
and
$\tau$
and
![]() $\nu$
, frequently plays an important role. From this viewpoint, we are looking at bitopological spaces
$\nu$
, frequently plays an important role. From this viewpoint, we are looking at bitopological spaces
![]() $(X,\tau, \omega )$
, where
$(X,\tau, \omega )$
, where
![]() $(X,\tau )$
is a
$(X,\tau )$
is a
![]() $T_0$
-space and
$T_0$
-space and
![]() $\omega$
is the lower topology defined from the order of specialization of
$\omega$
is the lower topology defined from the order of specialization of
![]() $(X,\tau )$
. We are interested in how these two topologies interact and focus primarily on the equivalent properties of
$(X,\tau )$
. We are interested in how these two topologies interact and focus primarily on the equivalent properties of
![]() $\Omega ^*$
-compactness (each
$\Omega ^*$
-compactness (each
![]() $\tau$
-closed set is compact in the lower topology) and property
$\tau$
-closed set is compact in the lower topology) and property
![]() $R$
(a kind of well-filtered property for the closed subsets of the lower topology). We also study strong
$R$
(a kind of well-filtered property for the closed subsets of the lower topology). We also study strong
![]() $d$
-spaces and its relationship to the preceding notions and investigate the conditions under which the Scott topology on a dcpo is sober.
$d$
-spaces and its relationship to the preceding notions and investigate the conditions under which the Scott topology on a dcpo is sober.
2. Preliminaries
In this section, we briefly recall some fundamental concepts and basic results about ordered structures and
![]() $T_0$
-spaces that will be used in the paper. For further details, we refer the reader to Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003), Goubault-Larrecq (Reference Goubault-Larrecq2013).
$T_0$
-spaces that will be used in the paper. For further details, we refer the reader to Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003), Goubault-Larrecq (Reference Goubault-Larrecq2013).
For a poset
![]() $P$
and
$P$
and
![]() $A\subseteq P$
, let
$A\subseteq P$
, let
![]() $\mathord{\downarrow }A=\{x\in P: x\leq a \mbox{ for some } a\in A\}$
and
$\mathord{\downarrow }A=\{x\in P: x\leq a \mbox{ for some } a\in A\}$
and
![]() $\mathord{\uparrow }A=\{x\in P: x\geq a \mbox{ for some } a\in A\}$
. For
$\mathord{\uparrow }A=\{x\in P: x\geq a \mbox{ for some } a\in A\}$
. For
![]() $x\in P$
, we write
$x\in P$
, we write
![]() $\mathord{\downarrow }x$
for
$\mathord{\downarrow }x$
for
![]() $\mathord{\downarrow }\{x\}$
and
$\mathord{\downarrow }\{x\}$
and
![]() $\mathord{\uparrow }x$
for
$\mathord{\uparrow }x$
for
![]() $\mathord{\uparrow }\{x\}$
. The set
$\mathord{\uparrow }\{x\}$
. The set
![]() $A$
is called a lower set (resp., an upper set) if
$A$
is called a lower set (resp., an upper set) if
![]() $A=\mathord{\downarrow }A$
(resp.,
$A=\mathord{\downarrow }A$
(resp.,
![]() $A=\mathord{\uparrow }A$
). The family of all upper subsets of
$A=\mathord{\uparrow }A$
). The family of all upper subsets of
![]() $P$
is denoted by
$P$
is denoted by
![]() $\mathbf{up}(P)$
. Let
$\mathbf{up}(P)$
. Let
![]() $P^{(\lt \omega )}=\{F\subseteq P : F\, \mbox{is a nonempty finite set}\}$
and
$P^{(\lt \omega )}=\{F\subseteq P : F\, \mbox{is a nonempty finite set}\}$
and
![]() $\mathbf{Fin} P=\{\uparrow F$
:
$\mathbf{Fin} P=\{\uparrow F$
:
![]() $F\in P^{(\lt \omega )}\}$
. An upper set
$F\in P^{(\lt \omega )}\}$
. An upper set
![]() $B$
of
$B$
of
![]() $P$
is said to be finitely generated if there is
$P$
is said to be finitely generated if there is
![]() $F\in P^{(\lt \omega )}$
such that
$F\in P^{(\lt \omega )}$
such that
![]() $B=\mathord{\uparrow } F$
. For a nonempty subset
$B=\mathord{\uparrow } F$
. For a nonempty subset
![]() $C$
of
$C$
of
![]() $P$
, define
$P$
, define
![]() $\mathrm{max}(C)=\{c\in C : c\, \mbox{is a maximal element of}\, C\}$
and
$\mathrm{max}(C)=\{c\in C : c\, \mbox{is a maximal element of}\, C\}$
and
![]() $\mathrm{min}(C)=\{c\in A : c\, \mbox{is a minimal element of}\, C\}$
.
$\mathrm{min}(C)=\{c\in A : c\, \mbox{is a minimal element of}\, C\}$
.
For a set
![]() $X$
, let
$X$
, let
![]() $|X|$
be the cardinality of
$|X|$
be the cardinality of
![]() $X$
and
$X$
and
![]() $2^X$
the set of all subsets of
$2^X$
the set of all subsets of
![]() $X$
. The set of all natural numbers is denoted by
$X$
. The set of all natural numbers is denoted by
![]() $\mathbb{N}$
. When
$\mathbb{N}$
. When
![]() $\mathbb{N}$
is regarded as a poset (in fact, a chain), the order on
$\mathbb{N}$
is regarded as a poset (in fact, a chain), the order on
![]() $\mathbb{N}$
is the usual order of natural numbers. Let
$\mathbb{N}$
is the usual order of natural numbers. Let
![]() $\omega =|\mathbb{N}|$
.
$\omega =|\mathbb{N}|$
.
A poset
![]() $P$
is called an inf semilattice (shortly a semilattice) if any two elements
$P$
is called an inf semilattice (shortly a semilattice) if any two elements
![]() $a, b\in P$
have the greatest lower bound in
$a, b\in P$
have the greatest lower bound in
![]() $P$
, denoted by
$P$
, denoted by
![]() $a\wedge b$
. Dually,
$a\wedge b$
. Dually,
![]() $P$
is a sup semilattice if any two elements
$P$
is a sup semilattice if any two elements
![]() $a, b\in P$
have the least upper bound in
$a, b\in P$
have the least upper bound in
![]() $P$
, denoted by
$P$
, denoted by
![]() $a\vee b$
. The poset
$a\vee b$
. The poset
![]() $P$
is called sup complete, if every nonempty subset of
$P$
is called sup complete, if every nonempty subset of
![]() $P$
has a sup (i.e., the least upper bound). In particular, a sup complete poset has a greatest element, the sup of
$P$
has a sup (i.e., the least upper bound). In particular, a sup complete poset has a greatest element, the sup of
![]() $P$
.
$P$
.
![]() $P$
is called a complete lattice if every subset (including the empty set) has a sup and an inf. A totally ordered complete lattice is called a complete chain.
$P$
is called a complete lattice if every subset (including the empty set) has a sup and an inf. A totally ordered complete lattice is called a complete chain.
A nonempty subset
![]() $D$
of a poset
$D$
of a poset
![]() $P$
is directed if every two elements in
$P$
is directed if every two elements in
![]() $D$
have an upper bound in
$D$
have an upper bound in
![]() $D$
. The set of all directed sets of
$D$
. The set of all directed sets of
![]() $P$
is denoted by
$P$
is denoted by
![]() $\mathscr{D}(P)$
. The poset
$\mathscr{D}(P)$
. The poset
![]() $P$
is called a directed complete poset, or dcpo for short, if for any
$P$
is called a directed complete poset, or dcpo for short, if for any
![]() $D\in \mathscr{D}(P)$
,
$D\in \mathscr{D}(P)$
,
![]() $\bigvee D$
exists in
$\bigvee D$
exists in
![]() $P$
. Clearly, a poset
$P$
. Clearly, a poset
![]() $Q$
is sup complete iff
$Q$
is sup complete iff
![]() $Q$
is both a dcpo and a sup semilattice.
$Q$
is both a dcpo and a sup semilattice.
Lemma 1.
Let
![]() $P$
be a poset and
$P$
be a poset and
![]() $D$
a countable directed subset of
$D$
a countable directed subset of
![]() $P$
. Then there exists a countable chain
$P$
. Then there exists a countable chain
![]() $C\subseteq D$
such that
$C\subseteq D$
such that
![]() $\mathord{\downarrow } D=\mathord{\downarrow } C$
. Hence,
$\mathord{\downarrow } D=\mathord{\downarrow } C$
. Hence,
![]() $\bigvee C$
exists and
$\bigvee C$
exists and
![]() $\bigvee C=\bigvee D$
whenever
$\bigvee C=\bigvee D$
whenever
![]() $\bigvee D$
exists. If
$\bigvee D$
exists. If
![]() $D$
has no largest element, then
$D$
has no largest element, then
![]() $C$
can be chosen to be a strictly ascending chain.
$C$
can be chosen to be a strictly ascending chain.
Proof. If
![]() $|D|\lt \omega$
, then
$|D|\lt \omega$
, then
![]() $D$
contains a largest element
$D$
contains a largest element
![]() $d$
, so let
$d$
, so let
![]() $C=\{d\}$
, which satisfies the requirement.
$C=\{d\}$
, which satisfies the requirement.
Now assume
![]() $|D|=\omega$
and let
$|D|=\omega$
and let
![]() $D=\{d_n:n\in \mathbb{N}\}$
. We use induction on
$D=\{d_n:n\in \mathbb{N}\}$
. We use induction on
![]() $n\in \mathbb{N}$
to define
$n\in \mathbb{N}$
to define
![]() $C=\{c_n:n\in \mathbb{N}\}$
. More precisely, let
$C=\{c_n:n\in \mathbb{N}\}$
. More precisely, let
![]() $c_1=d_1$
and let
$c_1=d_1$
and let
![]() $c_{n+1}$
(
$c_{n+1}$
(
![]() $n\in \mathbb{N}$
) be an upper bound of
$n\in \mathbb{N}$
) be an upper bound of
![]() $\{d_{n+1},c_0, c_1,c_2\ldots, c_n\}$
in
$\{d_{n+1},c_0, c_1,c_2\ldots, c_n\}$
in
![]() $D$
. It is clear that
$D$
. It is clear that
![]() $C$
is a chain and
$C$
is a chain and
![]() $\mathord{\downarrow } D=\mathord{\downarrow } C$
.
$\mathord{\downarrow } D=\mathord{\downarrow } C$
.
Suppose that
![]() $D=\{d_n:n\in \mathbb{N}\}$
is a countable directed and has no largest element. Let
$D=\{d_n:n\in \mathbb{N}\}$
is a countable directed and has no largest element. Let
![]() $c_1=d_1$
. Since
$c_1=d_1$
. Since
![]() $D$
has no largest element, there is
$D$
has no largest element, there is
![]() $d_{m_1}\in D$
such that
$d_{m_1}\in D$
such that
![]() $d_{m_1}\not \leq c_1$
. Let
$d_{m_1}\not \leq c_1$
. Let
![]() $c_2$
be an upper bound of
$c_2$
be an upper bound of
![]() $\{d_2,c_1, d_{m_1}\}$
in
$\{d_2,c_1, d_{m_1}\}$
in
![]() $D$
. Then
$D$
. Then
![]() $c_1\lt c_2$
and
$c_1\lt c_2$
and
![]() $\{d_1, d_2\}\subseteq \downarrow c_2$
. We assume generally that for
$\{d_1, d_2\}\subseteq \downarrow c_2$
. We assume generally that for
![]() $n\in \mathbb{N}$
we have chosen in
$n\in \mathbb{N}$
we have chosen in
![]() $D$
finite elements
$D$
finite elements
![]() $c_i$
(
$c_i$
(
![]() $1\leq i\leq n$
) such that
$1\leq i\leq n$
) such that
![]() $ c_1\lt c_2\lt \ldots \lt c_n$
and
$ c_1\lt c_2\lt \ldots \lt c_n$
and
![]() $\{d_1, d_2, \ldots, d_n\}\subseteq \downarrow c_n$
. Then as
$\{d_1, d_2, \ldots, d_n\}\subseteq \downarrow c_n$
. Then as
![]() $D$
has no largest element, there is
$D$
has no largest element, there is
![]() $d_{m_n}\in D$
such that
$d_{m_n}\in D$
such that
![]() $d_{m_n}\not \leq c_n$
. Let
$d_{m_n}\not \leq c_n$
. Let
![]() $c_{n+1}$
be an upper bound of
$c_{n+1}$
be an upper bound of
![]() $\{d_{n+1},c_n, d_{m_n}\}$
in
$\{d_{n+1},c_n, d_{m_n}\}$
in
![]() $D$
. Then
$D$
. Then
![]() $c_n\lt c_{n+1}$
and
$c_n\lt c_{n+1}$
and
![]() $\{d_1, d_2, \ldots, d_{n+1}\}\subseteq \downarrow c_{n+1}$
. So by induction we get a strictly ascending chain
$\{d_1, d_2, \ldots, d_{n+1}\}\subseteq \downarrow c_{n+1}$
. So by induction we get a strictly ascending chain
![]() $C=\{c_n : n\in \mathbb{N}\}$
satisfying
$C=\{c_n : n\in \mathbb{N}\}$
satisfying
![]() $\mathord{\downarrow } D=\mathord{\downarrow } C$
.
$\mathord{\downarrow } D=\mathord{\downarrow } C$
.
The category of all
![]() $T_0$
-spaces and continuous mappings is denoted by
$T_0$
-spaces and continuous mappings is denoted by
![]() $\mathbf{Top}_0$
. For a
$\mathbf{Top}_0$
. For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
, let
$X$
, let
![]() $\mathscr{O}(X)$
(resp.,
$\mathscr{O}(X)$
(resp.,
![]() $\mathscr{C}(X)$
) be the set of all open subsets (resp., closed subsets) of
$\mathscr{C}(X)$
) be the set of all open subsets (resp., closed subsets) of
![]() $X$
. The closure of a subset
$X$
. The closure of a subset
![]() $A$
in
$A$
in
![]() $X$
will be denoted by
$X$
will be denoted by
![]() ${\textrm{cl}}_X A$
(or simply by
${\textrm{cl}}_X A$
(or simply by
![]() ${\textrm{cl}} A$
if there is no ambiguity) or
${\textrm{cl}} A$
if there is no ambiguity) or
![]() $\overline{A}$
, and the interior of
$\overline{A}$
, and the interior of
![]() $A$
will be denoted by
$A$
will be denoted by
![]() ${\textrm{int}}_X A$
or simply by
${\textrm{int}}_X A$
or simply by
![]() ${\textrm{int}} A$
. Let
${\textrm{int}} A$
. Let
![]() $\mathscr{D}_c(X)=\{\overline{D} : D\in \mathscr{D}(X)\}$
. We use
$\mathscr{D}_c(X)=\{\overline{D} : D\in \mathscr{D}(X)\}$
. We use
![]() $\leq _X$
to denote the specialization order of
$\leq _X$
to denote the specialization order of
![]() $X$
:
$X$
:
![]() $x\leq _X y$
iff
$x\leq _X y$
iff
![]() $x\in \overline{\{y\}}$
. Clearly, all open sets (resp., closed sets) of
$x\in \overline{\{y\}}$
. Clearly, all open sets (resp., closed sets) of
![]() $X$
are upper sets (resp., lower sets) of
$X$
are upper sets (resp., lower sets) of
![]() $X$
. A subset
$X$
. A subset
![]() $B$
of
$B$
of
![]() $X$
is called saturated if
$X$
is called saturated if
![]() $B$
equals the intersection of all open sets containing it (equivalently,
$B$
equals the intersection of all open sets containing it (equivalently,
![]() $B$
is an upper set in the specialization order). For a poset
$B$
is an upper set in the specialization order). For a poset
![]() $P$
, a
$P$
, a
![]() $T_0$
-topology
$T_0$
-topology
![]() $\tau$
on
$\tau$
on
![]() $P$
is said to be order-compatible if
$P$
is said to be order-compatible if
![]() $\leq _{(P, \tau )}$
(shortly
$\leq _{(P, \tau )}$
(shortly
![]() $\leq _\tau$
) agrees with the original order on
$\leq _\tau$
) agrees with the original order on
![]() $P$
.
$P$
.
In what follows, when a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is considered as a poset, the order always refers to the specialization order if no other explanation is given. We will use
$X$
is considered as a poset, the order always refers to the specialization order if no other explanation is given. We will use
![]() $\Omega X$
or simply
$\Omega X$
or simply
![]() $X$
to denote the poset
$X$
to denote the poset
![]() $(X, \leq _X)$
.
$(X, \leq _X)$
.
Definition 2.
Let
![]() $P$
be a poset equipped with a topology. The partial order is said to be upper-semiclosed if each
$P$
be a poset equipped with a topology. The partial order is said to be upper-semiclosed if each
![]() $\mathord{\uparrow } x$
is closed.
$\mathord{\uparrow } x$
is closed.
Definition 3.
A topological space
![]() $X$
with a partial order is called upper-semicompact, if
$X$
with a partial order is called upper-semicompact, if
![]() $\mathord{\uparrow } x$
is compact for any
$\mathord{\uparrow } x$
is compact for any
![]() $x\in X$
, or equivalently, if
$x\in X$
, or equivalently, if
![]() $\mathord{\uparrow } x\cap A$
is compact for any
$\mathord{\uparrow } x\cap A$
is compact for any
![]() $x\in X$
and
$x\in X$
and
![]() $A\in \mathscr{C}(X)$
.
$A\in \mathscr{C}(X)$
.
For a set
![]() $X$
and two topologies
$X$
and two topologies
![]() $\tau$
and
$\tau$
and
![]() $\nu$
on
$\nu$
on
![]() $X$
, the join
$X$
, the join
![]() $\tau \bigvee \nu$
is the topology generated by
$\tau \bigvee \nu$
is the topology generated by
![]() $\tau \bigcup \nu$
. It is the smallest topology on
$\tau \bigcup \nu$
. It is the smallest topology on
![]() $X$
containing both
$X$
containing both
![]() $\tau$
and
$\tau$
and
![]() $\nu$
.
$\nu$
.
A subset
![]() $U$
of a poset
$U$
of a poset
![]() $P$
is said to be Scott-open if (i)
$P$
is said to be Scott-open if (i)
![]() $U=\mathord{\uparrow }U$
, and (ii) for any directed subset
$U=\mathord{\uparrow }U$
, and (ii) for any directed subset
![]() $D$
with
$D$
with
![]() $\bigvee D$
existing,
$\bigvee D$
existing,
![]() $\bigvee D\in U$
implies
$\bigvee D\in U$
implies
![]() $D\cap U\neq \emptyset$
. All Scott-open subsets of
$D\cap U\neq \emptyset$
. All Scott-open subsets of
![]() $P$
form a topology, called the Scott topology on
$P$
form a topology, called the Scott topology on
![]() $P$
and denoted by
$P$
and denoted by
![]() $\sigma (P)$
. The space
$\sigma (P)$
. The space
![]() $\Sigma P=(P,\sigma (P))$
is called the Scott space of
$\Sigma P=(P,\sigma (P))$
is called the Scott space of
![]() $P$
. A subset
$P$
. A subset
![]() $C$
of
$C$
of
![]() $P$
is said to be Scott-compact if it is compact in
$P$
is said to be Scott-compact if it is compact in
![]() $\Sigma P$
. For the chain
$\Sigma P$
. For the chain
![]() $2 =\{0, 1\}$
(with the order
$2 =\{0, 1\}$
(with the order
![]() $0\lt 1$
), we have
$0\lt 1$
), we have
![]() $\sigma (2)=\{\emptyset, \{1\}, \{0, 1\}\}$
. The space
$\sigma (2)=\{\emptyset, \{1\}, \{0, 1\}\}$
. The space
![]() $\Sigma 2$
is well-known under the name of Sierpiński space.
$\Sigma 2$
is well-known under the name of Sierpiński space.
The lower topology on
![]() $P$
, generated by
$P$
, generated by
![]() $\{P\setminus \mathord{\uparrow } x : x\in P\}$
(as a subbase), is denoted by
$\{P\setminus \mathord{\uparrow } x : x\in P\}$
(as a subbase), is denoted by
![]() $\omega (P)$
. Dually, define the upper topology on
$\omega (P)$
. Dually, define the upper topology on
![]() $P$
(generated by
$P$
(generated by
![]() $\{P\setminus \mathord{\downarrow } x : x\in P\}$
) and denote it by
$\{P\setminus \mathord{\downarrow } x : x\in P\}$
) and denote it by
![]() $\upsilon (P)$
. The topology
$\upsilon (P)$
. The topology
![]() $\sigma (P)\bigvee \omega (P)$
is called the Lawson topology on
$\sigma (P)\bigvee \omega (P)$
is called the Lawson topology on
![]() $P$
and is denoted by
$P$
and is denoted by
![]() $\lambda (P)$
. The collection of all upper sets of
$\lambda (P)$
. The collection of all upper sets of
![]() $P$
forms the (upper) Alexandroff topology
$P$
forms the (upper) Alexandroff topology
![]() $\alpha (P)$
. For a
$\alpha (P)$
. For a
![]() $T_0$
-topology
$T_0$
-topology
![]() $\tau$
on
$\tau$
on
![]() $P$
, it is easy to verify that
$P$
, it is easy to verify that
![]() $\tau$
is order-compatible iff
$\tau$
is order-compatible iff
![]() $\upsilon (P) \subseteq \tau \subseteq \alpha (P)$
.
$\upsilon (P) \subseteq \tau \subseteq \alpha (P)$
.
In the following, when a poset
![]() $P$
is considered as a
$P$
is considered as a
![]() $T_0$
-space, the topology on
$T_0$
-space, the topology on
![]() $P$
always refers to the Scott topology unless stated otherwise.
$P$
always refers to the Scott topology unless stated otherwise.
The following three results are well-known (see Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003, Proposition II-2.1, Theorem III-1.9, and Proposition VI-1.6). The first is a key feature of the Scott topology.
Lemma 4.
Let
![]() $P, Q$
be posets and
$P, Q$
be posets and
![]() $f : P \longrightarrow Q$
. Then the following two conditions are equivalent:
$f : P \longrightarrow Q$
. Then the following two conditions are equivalent:
-
(1)
 $f$
is Scott continuous, that is,
$f$
is Scott continuous, that is,
 $f : \Sigma P \longrightarrow \Sigma Q$
is continuous.
$f : \Sigma P \longrightarrow \Sigma Q$
is continuous.
-
(2) For any
 $D\in \mathscr{D}(P)$
for which
$D\in \mathscr{D}(P)$
for which
 $\bigvee D$
exists,
$\bigvee D$
exists,
 $f(\bigvee D)=\bigvee f(D)$
.
$f(\bigvee D)=\bigvee f(D)$
.
Lemma 5.
Let
![]() $X$
be a topological space with an upper-semiclosed partial order. If
$X$
be a topological space with an upper-semiclosed partial order. If
![]() $A$
is a compact subset of
$A$
is a compact subset of
![]() $X$
, then
$X$
, then
![]() $\mathord{\downarrow } A$
is Scott-closed.
$\mathord{\downarrow } A$
is Scott-closed.
Lemma 6.
For a complete lattice
![]() $L$
,
$L$
,
![]() $(L, \lambda (L))$
is a compact
$(L, \lambda (L))$
is a compact
![]() $T_1$
-space.
$T_1$
-space.
Lemma 7.
(Jia Reference Jia2018
, Theorem 3.4) For a poset
![]() $P$
,
$P$
,
![]() $\Sigma P$
is compact iff
$\Sigma P$
is compact iff
![]() $P$
is finitely generated.
$P$
is finitely generated.
Lemma 8.
(Rudin’s Lemma) Let
![]() $P$
be a poset,
$P$
be a poset,
![]() $C$
a nonempty lower subset of
$C$
a nonempty lower subset of
![]() $P$
, and
$P$
, and
![]() $\mathscr{F} \subseteq \mathbf{Fin} P$
a filtered family. If
$\mathscr{F} \subseteq \mathbf{Fin} P$
a filtered family. If
![]() $C$
meets all members of
$C$
meets all members of
![]() $\mathscr{F}$
, then
$\mathscr{F}$
, then
![]() $C$
contains a directed subset
$C$
contains a directed subset
![]() $D$
that still meets all members of
$D$
that still meets all members of
![]() $\mathscr{F}$
.
$\mathscr{F}$
.
Rudin’s Lemma, given by Rudin (Reference Rudin1981), is a useful tool in topology and plays a crucial role in domain theory (see Gierz et al. Reference Gierz, Lawson and Stralka1983, Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Goubault-Larrecq Reference Goubault-Larrecq2013). On some occasions, we only need the following consequence of Rudin’s Lemma.
Corollary 9.
(Heckmann Reference Heckmann1992, Lemma 2.4) Let
![]() $P$
be a dcpo,
$P$
be a dcpo,
![]() $U$
a nonempty Scott-open subset of
$U$
a nonempty Scott-open subset of
![]() $P$
and
$P$
and
![]() $\mathscr{F}\subseteq \mathbf{Fin} P$
a filtered family such that
$\mathscr{F}\subseteq \mathbf{Fin} P$
a filtered family such that
![]() $\bigcap \mathscr{F}\subseteq U$
holds. Then
$\bigcap \mathscr{F}\subseteq U$
holds. Then
![]() $\mathord{\uparrow } F\subseteq U$
for some
$\mathord{\uparrow } F\subseteq U$
for some
![]() $\mathord{\uparrow } F\in \mathscr{F}$
.
$\mathord{\uparrow } F\in \mathscr{F}$
.
Definition 10.
A poset
![]() $P$
is said to be Noetherian if it satisfies the ascending chain condition (ACC for short): every ascending chain has a greatest member or, equivalently, every chain of
$P$
is said to be Noetherian if it satisfies the ascending chain condition (ACC for short): every ascending chain has a greatest member or, equivalently, every chain of
![]() $P$
has a largest member.
$P$
has a largest member.
A
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is said to be hyper-sober if for any
$X$
is said to be hyper-sober if for any
![]() $F\in{\mathsf{Irr}} (X)$
, there is a unique
$F\in{\mathsf{Irr}} (X)$
, there is a unique
![]() $x\in F$
such that
$x\in F$
such that
![]() $F\subseteq{\textrm{cl}} \{x\}$
(cf. Zhao and Ho Reference Zhao and Ho2015).
$F\subseteq{\textrm{cl}} \{x\}$
(cf. Zhao and Ho Reference Zhao and Ho2015).
Proposition 11.
(Zhao and Ho Reference Zhao and Ho2015, Proposition 5.4 and Theorem 5.7) (Xu et al. Reference Xu, Shen, Xi and Zhao2020a, Proposition 3.8) For a poset
![]() $P$
, the following conditions are equivalent:
$P$
, the following conditions are equivalent:
-
(1)
 $P$
is a Noetherian poset.
$P$
is a Noetherian poset.
-
(2) Every directed subset of
 $P$
has a largest member.
$P$
has a largest member.
-
(3) Every ideal of
 $P$
is principal.
$P$
is principal.
-
(4) Every countable directed set of
 $P$
has a largest member.
$P$
has a largest member.
-
(5) Every countable chain of
 $P$
has a largest member.
$P$
has a largest member.
-
(6) Every countable ascending chain of
 $P$
has a largest member.
$P$
has a largest member.
-
(7)
 $P$
is a dcpo and every element of
$P$
is a dcpo and every element of
 $P$
is compact.
$P$
is compact.
-
(8)
 $P$
is a dcpo and
$P$
is a dcpo and
 $\sigma (P)=\alpha (P)$
.
$\sigma (P)=\alpha (P)$
. -
(9) The Alexandroff topology
 $\alpha (P)$
is sober.
$\alpha (P)$
is sober.
-
(10) The Scott topology
 $\sigma (P)$
is hyper-sober.
$\sigma (P)$
is hyper-sober.
Proposition 12.
For a poset
![]() $P$
, the following two conditions are equivalent:
$P$
, the following two conditions are equivalent:
-
(1)
 $P$
is a Noetherian poset.
$P$
is a Noetherian poset.
-
(2)
 $\mathbf{Fin} P$
(with the inverse inclusion order) is a Noetherian poset.
$\mathbf{Fin} P$
(with the inverse inclusion order) is a Noetherian poset.
Proof. (1)
![]() $\Rightarrow$
(2): Let
$\Rightarrow$
(2): Let
![]() $P$
be a Noetherian poset. Then by Proposition 11,
$P$
be a Noetherian poset. Then by Proposition 11,
![]() $P$
is a dcpo and
$P$
is a dcpo and
![]() $\sigma (P)=\alpha (P)$
. Hence by Proposition 3 of Xi and Zhao (Reference Xi and Zhao2017) (see Lemma 24 below),
$\sigma (P)=\alpha (P)$
. Hence by Proposition 3 of Xi and Zhao (Reference Xi and Zhao2017) (see Lemma 24 below),
![]() $\mathord{\mathsf{K}} (\Sigma P)=\mathord{\mathsf{K}} ((P, \alpha (P))=\mathbf{Fin} P$
is a dcpo. Now we show that
$\mathord{\mathsf{K}} (\Sigma P)=\mathord{\mathsf{K}} ((P, \alpha (P))=\mathbf{Fin} P$
is a dcpo. Now we show that
![]() $\mathord{\uparrow } F\ll \mathord{\uparrow } F$
in
$\mathord{\uparrow } F\ll \mathord{\uparrow } F$
in
![]() $\mathbf{Fin} P$
for all
$\mathbf{Fin} P$
for all
![]() $\mathord{\uparrow } F\in \mathbf{Fin} P$
. Suppose that
$\mathord{\uparrow } F\in \mathbf{Fin} P$
. Suppose that
![]() $\{\mathord{\uparrow } F_d : d\in D\}\in \mathscr{D}(\mathbf{Fin} P)$
such that
$\{\mathord{\uparrow } F_d : d\in D\}\in \mathscr{D}(\mathbf{Fin} P)$
such that
![]() $\mathord{\uparrow } F \sqsubseteq \bigvee _{\mathbf{Fin} P}\{\mathord{\uparrow } F_d : d\in D\}$
. Then by Lemma 23 below, we have that
$\mathord{\uparrow } F \sqsubseteq \bigvee _{\mathbf{Fin} P}\{\mathord{\uparrow } F_d : d\in D\}$
. Then by Lemma 23 below, we have that
![]() $\bigcap _{d\in D}\mathord{\uparrow } F_d\subseteq \mathord{\uparrow } F\in \sigma (P)$
, whence by Corollary 9 there is
$\bigcap _{d\in D}\mathord{\uparrow } F_d\subseteq \mathord{\uparrow } F\in \sigma (P)$
, whence by Corollary 9 there is
![]() $d\in D$
such that
$d\in D$
such that
![]() $\mathord{\uparrow } F_d\subseteq \mathord{\uparrow } F$
, that is
$\mathord{\uparrow } F_d\subseteq \mathord{\uparrow } F$
, that is
![]() $\mathord{\uparrow } F\sqsubseteq \mathord{\uparrow } F_d$
. Thus,
$\mathord{\uparrow } F\sqsubseteq \mathord{\uparrow } F_d$
. Thus,
![]() $\mathord{\uparrow } F\ll _{\mathbf{Fin} P}\mathord{\uparrow } F$
. By Proposition 11,
$\mathord{\uparrow } F\ll _{\mathbf{Fin} P}\mathord{\uparrow } F$
. By Proposition 11,
![]() $\mathbf{Fin}P$
is a Noetherian poset.
$\mathbf{Fin}P$
is a Noetherian poset.
(2)
![]() $\Rightarrow$
(1): Suppose that
$\Rightarrow$
(1): Suppose that
![]() $\mathbf{Fin}P$
is a Noetherian poset and
$\mathbf{Fin}P$
is a Noetherian poset and
![]() $D\in \mathscr{D}(P)$
. If
$D\in \mathscr{D}(P)$
. If
![]() $D$
has no largest element, the
$D$
has no largest element, the
![]() $\{\mathord{\uparrow } d : d\in D\}$
is a directed subset of
$\{\mathord{\uparrow } d : d\in D\}$
is a directed subset of
![]() $\mathbf{Fin}P$
having no largest member, which is a contradiction with the Noetherian property of
$\mathbf{Fin}P$
having no largest member, which is a contradiction with the Noetherian property of
![]() $\mathbf{Fin}P$
. So
$\mathbf{Fin}P$
. So
![]() $P$
is a Noetherian poset.
$P$
is a Noetherian poset.
For the following definition and related conceptions, please refer to Abramsky and Jung (Reference Abramsky and Jung1994), Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003), Goubault-Larrecq (Reference Goubault-Larrecq2013).
Definition 13.
For a dcpo
![]() $P$
and
$P$
and
![]() $A, B\subseteq P$
, we say
$A, B\subseteq P$
, we say
![]() $A$
is way below
$A$
is way below
![]() $B$
, written
$B$
, written
![]() $A\ll B$
, if for each
$A\ll B$
, if for each
![]() $D\in \mathscr{D}(P)$
,
$D\in \mathscr{D}(P)$
,
![]() $\bigvee D\in \mathord{\uparrow } B$
implies
$\bigvee D\in \mathord{\uparrow } B$
implies
![]() $D\cap \mathord{\uparrow } A\neq \emptyset$
. For
$D\cap \mathord{\uparrow } A\neq \emptyset$
. For
![]() $B=\{x\}$
, a singleton,
$B=\{x\}$
, a singleton,
![]() $A\ll B$
is written
$A\ll B$
is written
![]() $A\ll x$
for short. For
$A\ll x$
for short. For
![]() $x\in P$
, let
$x\in P$
, let
![]() $w(x)=\{F\in P^{(\lt \omega )} : F\ll x\}$
,
$w(x)=\{F\in P^{(\lt \omega )} : F\ll x\}$
,
![]() $\Downarrow x = \{u\in P : u\ll x\}$
and
$\Downarrow x = \{u\in P : u\ll x\}$
and
![]() $K(P)=\{k\in P : k\ll k\}$
. Points in
$K(P)=\{k\in P : k\ll k\}$
. Points in
![]() $K(P)$
are called compact elements of
$K(P)$
are called compact elements of
![]() $P$
.
$P$
.
Definition 14.
Let
![]() $P$
be a dcpo and
$P$
be a dcpo and
![]() $X$
a
$X$
a
![]() $T_0$
-space.
$T_0$
-space.
-
(1)
 $P$
is called a continuous domain, if for each
$P$
is called a continuous domain, if for each
 $x\in P$
,
$x\in P$
,
 $\Downarrow x$
is directed and
$\Downarrow x$
is directed and
 $x=\bigvee \Downarrow x$
.
$x=\bigvee \Downarrow x$
. -
(2)
 $P$
is called an algebraic domain, if for each
$P$
is called an algebraic domain, if for each
 $x\in P$
,
$x\in P$
,
 $\downarrow x\cap K(P)$
is directed and
$\downarrow x\cap K(P)$
is directed and
 $x=\bigvee (\downarrow x\cap K(P))$
.
$x=\bigvee (\downarrow x\cap K(P))$
. -
(3)
 $P$
is called a quasicontinuous domain, if for each
$P$
is called a quasicontinuous domain, if for each
 $x\in P$
,
$x\in P$
,
 $\{\mathord{\uparrow } F : F\in w(x)\}$
is filtered and
$\{\mathord{\uparrow } F : F\in w(x)\}$
is filtered and
 $\mathord{\uparrow } x=\bigcap \{\mathord{\uparrow } F : F\in w(x)\}$
.
$\mathord{\uparrow } x=\bigcap \{\mathord{\uparrow } F : F\in w(x)\}$
. -
(4)
 $X$
is called core-compact if
$X$
is called core-compact if
 $\mathscr{O}(X)$
is a continuous lattice.
$\mathscr{O}(X)$
is a continuous lattice.
It is well-known that every algebraic domain is a continuous domain and every continuous domain is a quasicontinuous domain but the converse implications do not hold in general (see Gierz et al. Reference Gierz, Lawson and Stralka1983, Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003).
For the concepts in the following definition, please refer to Erné (Reference Erné2018), Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003), Heckmann (Reference Heckmann1992), Heckmann and Keimel (Reference Heckmann and Keimel2013).
Definition 15.
Let
![]() $X$
be a topological space and
$X$
be a topological space and
![]() $S\subseteq X$
.
$S\subseteq X$
.
-
(1)
 $S$
is called strongly compact if for any open set
$S$
is called strongly compact if for any open set
 $U$
with
$U$
with
 $S \subseteq U$
, there is a finite set
$S \subseteq U$
, there is a finite set
 $F$
with
$F$
with
 $S\subseteq \uparrow F \subseteq U$
.
$S\subseteq \uparrow F \subseteq U$
. -
(2)
 $S$
is called supercompact if for any family
$S$
is called supercompact if for any family
 $\{U_i : i\in I\}\subseteq \mathscr{O}(X)$
,
$\{U_i : i\in I\}\subseteq \mathscr{O}(X)$
,
 $S\subseteq \bigcup _{i\in I} U_i$
implies
$S\subseteq \bigcup _{i\in I} U_i$
implies
 $S\subseteq U_i$
for some
$S\subseteq U_i$
for some
 $i\in I$
.
$i\in I$
. -
(3)
 $X$
is called locally hypercompact if for each
$X$
is called locally hypercompact if for each
 $x\in X$
and each open neighborhood
$x\in X$
and each open neighborhood
 $U$
of
$U$
of
 $x$
, there is a strongly compact set
$x$
, there is a strongly compact set
 $S$
such that
$S$
such that
 $x\in{\textrm{int}}\,\mathord{\uparrow } S\subseteq \mathord{\uparrow } S\subseteq U$
or, equivalently, there is
$x\in{\textrm{int}}\,\mathord{\uparrow } S\subseteq \mathord{\uparrow } S\subseteq U$
or, equivalently, there is
 $\mathord{\uparrow } F\in \mathbf{Fin}X$
such that
$\mathord{\uparrow } F\in \mathbf{Fin}X$
such that
 $x\in{\textrm{int}}\,\mathord{\uparrow } F\subseteq \mathord{\uparrow } F\subseteq U$
.
$x\in{\textrm{int}}\,\mathord{\uparrow } F\subseteq \mathord{\uparrow } F\subseteq U$
. -
(4)
 $X$
is called a
$X$
is called a
 $C$
-space if for each
$C$
-space if for each
 $x\in X$
and each open neighborhood
$x\in X$
and each open neighborhood
 $U$
of
$U$
of
 $x$
, there is a supercompact set
$x$
, there is a supercompact set
 $S$
such that
$S$
such that
 $x\in{\textrm{int}}\,\mathord{\uparrow } S\subseteq \mathord{\uparrow } S\subseteq U$
or, equivalently, there is
$x\in{\textrm{int}}\,\mathord{\uparrow } S\subseteq \mathord{\uparrow } S\subseteq U$
or, equivalently, there is
 $u\in U$
such that
$u\in U$
such that
 $x\in{\textrm{int}}\,\mathord{\uparrow } u\subseteq \mathord{\uparrow } u\subseteq U$
.
$x\in{\textrm{int}}\,\mathord{\uparrow } u\subseteq \mathord{\uparrow } u\subseteq U$
.
The following result is well-known (see Gierz et al. Reference Gierz, Lawson and Stralka1983, Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Heckmann Reference Heckmann1992).
Lemma 16.
For a dcpo
![]() $P$
, the following three conditions are equivalent:
$P$
, the following three conditions are equivalent:
-
(1)
 $P$
is continuous (resp., quasicontinuous).
$P$
is continuous (resp., quasicontinuous).
-
(2)
 $\Sigma P$
is a C-space (resp., a locally hypercompact space).
$\Sigma P$
is a C-space (resp., a locally hypercompact space).
-
(3) For each
 $U\in \sigma (P)$
and
$U\in \sigma (P)$
and
 $x\in U$
, there is
$x\in U$
, there is
 $u\in U$
such that
$u\in U$
such that
 $x\in{\textrm{int}}\,\!_{\sigma (P)}\mathord{\uparrow } u\subseteq \mathord{\uparrow } u\subseteq U$
(resp., there is
$x\in{\textrm{int}}\,\!_{\sigma (P)}\mathord{\uparrow } u\subseteq \mathord{\uparrow } u\subseteq U$
(resp., there is
 $\mathord{\uparrow } F\in \mathbf{Fin}X$
such that
$\mathord{\uparrow } F\in \mathbf{Fin}X$
such that
 $x\in{\textrm{int}}\,\mathord{\uparrow } F\subseteq \mathord{\uparrow } F\subseteq U$
).
$x\in{\textrm{int}}\,\mathord{\uparrow } F\subseteq \mathord{\uparrow } F\subseteq U$
).
Theorem 17.
(Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003
, Theorem II-4.13) Let
![]() $P$
be a poset. Then, the following statements are equivalent:
$P$
be a poset. Then, the following statements are equivalent:
-
(1)
 $\Sigma P$
is core-compact (i.e.,
$\Sigma P$
is core-compact (i.e.,
 $\sigma (P)$
is a continuous lattice).
$\sigma (P)$
is a continuous lattice).
-
(2) For every poset
 $S$
one has
$S$
one has
 $\Sigma (P\times S)=\Sigma P\times \Sigma S$
, that is, the Scott topology of
$\Sigma (P\times S)=\Sigma P\times \Sigma S$
, that is, the Scott topology of
 $P\times S$
is equal to the product of the individual Scott topologies.
$P\times S$
is equal to the product of the individual Scott topologies.
-
(3) For every dcpo or complete lattice
 $S$
one has
$S$
one has
 $\Sigma (P\times S)=\Sigma P\times \Sigma S$
.
$\Sigma (P\times S)=\Sigma P\times \Sigma S$
. -
(4)
 $\Sigma (P\times \sigma (P))=\Sigma P\times \Sigma \sigma (P)$
.
$\Sigma (P\times \sigma (P))=\Sigma P\times \Sigma \sigma (P)$
.
Proof. It was proved in Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003) for dcpos (see the proof of Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003, Theorem II-4.13) and the proof is valid for posets.
Corollary 18.
Suppose that
![]() $P$
is a poset for which
$P$
is a poset for which
![]() $\Sigma P$
is locally compact. Then for every poset
$\Sigma P$
is locally compact. Then for every poset
![]() $S$
,
$S$
,
![]() $\Sigma (P\times S)=\Sigma P\times \Sigma S$
.
$\Sigma (P\times S)=\Sigma P\times \Sigma S$
.
A
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is called a
$X$
is called a
![]() $d$
-space (or monotone convergence space) if
$d$
-space (or monotone convergence space) if
![]() $X$
(with the specialization order) is a dcpo and
$X$
(with the specialization order) is a dcpo and
![]() $\mathscr{O}(X) \subseteq \sigma (X)$
(cf. Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Wyler Reference Wyler1981).
$\mathscr{O}(X) \subseteq \sigma (X)$
(cf. Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003; Wyler Reference Wyler1981).
The
![]() $d$
-space has the following useful property.
$d$
-space has the following useful property.
Lemma 19.
Let
![]() $X$
be a
$X$
be a
![]() $d$
-space. Then for any nonempty closed set
$d$
-space. Then for any nonempty closed set
![]() $A$
of
$A$
of
![]() $X$
,
$X$
,
![]() $A=\downarrow \mathrm{max}(A)$
, whence
$A=\downarrow \mathrm{max}(A)$
, whence
![]() $\mathrm{max}(A)\neq \emptyset$
.
$\mathrm{max}(A)\neq \emptyset$
.
Proof. For
![]() $a\in A$
, by Zorn’s Lemma there is a maximal chain
$a\in A$
, by Zorn’s Lemma there is a maximal chain
![]() $C$
in
$C$
in
![]() $A$
with
$A$
with
![]() $a\in C$
. As
$a\in C$
. As
![]() $X$
is a
$X$
is a
![]() $d$
-space,
$d$
-space,
![]() $c=\bigvee C$
exists in
$c=\bigvee C$
exists in
![]() $X$
(with the specialization order) and
$X$
(with the specialization order) and
![]() $c\in A$
. Hence,
$c\in A$
. Hence,
![]() $a\leq c\in \max (A)$
since
$a\leq c\in \max (A)$
since
![]() $C$
is a maximal chain in
$C$
is a maximal chain in
![]() $A$
with
$A$
with
![]() $a\in C$
. Therefore,
$a\in C$
. Therefore,
![]() $A=\downarrow \mathrm{max}(A)$
.
$A=\downarrow \mathrm{max}(A)$
.
Proposition 20.
For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
, the following conditions are equivalent:
$X$
, the following conditions are equivalent:
-
(1)
 $X$
is a
$X$
is a
 $d$
-space.
$d$
-space.
-
(2) For any
 $D\in \mathscr{D}(X)$
and
$D\in \mathscr{D}(X)$
and
 $U\in \mathscr{O}(X)$
,
$U\in \mathscr{O}(X)$
,
 $\bigcap \limits \limits _{d\in D}\mathord{\uparrow } d\subseteq U$
implies
$\bigcap \limits \limits _{d\in D}\mathord{\uparrow } d\subseteq U$
implies
 $\mathord{\uparrow } d \subseteq U$
(i.e.,
$\mathord{\uparrow } d \subseteq U$
(i.e.,
 $d\in U$
) for some
$d\in U$
) for some
 $d\in D$
.
$d\in D$
. -
(3) For any filtered family
 $\{\mathord{\uparrow }F_{i}:i\in I\}\subseteq \mathbf{Fin}X$
and any
$\{\mathord{\uparrow }F_{i}:i\in I\}\subseteq \mathbf{Fin}X$
and any
 $U\in \mathscr{O}(X)$
,
$U\in \mathscr{O}(X)$
,
 $\bigcap _{i\in I}\mathord{\uparrow }F_{i}\subseteq U$
implies
$\bigcap _{i\in I}\mathord{\uparrow }F_{i}\subseteq U$
implies
 $\mathord{\uparrow }F_{i}\subseteq U$
for some
$\mathord{\uparrow }F_{i}\subseteq U$
for some
 $i\in I$
.
$i\in I$
.
Proof. (1)
![]() $\Leftrightarrow$
(2): See (Xu et al. Reference Xu, Shen, Xi and Zhao2020b, Proposition 3.3).
$\Leftrightarrow$
(2): See (Xu et al. Reference Xu, Shen, Xi and Zhao2020b, Proposition 3.3).
(3)
![]() $\Rightarrow$
(2): Trivial.
$\Rightarrow$
(2): Trivial.
(1)
![]() $\Rightarrow$
(3): Let
$\Rightarrow$
(3): Let
![]() $U$
be an open subset of
$U$
be an open subset of
![]() $X$
and
$X$
and
![]() $\mathscr{F}\subseteq \mathbf{Fin} X$
a filtered family such that
$\mathscr{F}\subseteq \mathbf{Fin} X$
a filtered family such that
![]() $\bigcap \mathscr{F}\subseteq U$
holds. As
$\bigcap \mathscr{F}\subseteq U$
holds. As
![]() $X$
is a
$X$
is a
![]() $d$
-space,
$d$
-space,
![]() $X$
(with the specialization order)) is a dcpo and
$X$
(with the specialization order)) is a dcpo and
![]() $U\in \sigma (X)$
. By Corollary 9,
$U\in \sigma (X)$
. By Corollary 9,
![]() $\mathord{\uparrow }F_{i}\subseteq U$
for some
$\mathord{\uparrow }F_{i}\subseteq U$
for some
![]() $i\in I$
.
$i\in I$
.
For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
and a nonempty subset
$X$
and a nonempty subset
![]() $A$
of
$A$
of
![]() $X$
,
$X$
,
![]() $A$
is irreducible if for any
$A$
is irreducible if for any
![]() $\{F_1, F_2\}\subseteq \mathscr{C}(X)$
,
$\{F_1, F_2\}\subseteq \mathscr{C}(X)$
,
![]() $A \subseteq F_1\cup F_2$
implies
$A \subseteq F_1\cup F_2$
implies
![]() $A \subseteq F_1$
or
$A \subseteq F_1$
or
![]() $A \subseteq F_2$
. Denote by
$A \subseteq F_2$
. Denote by
![]() ${\mathsf{Irr}}(X)$
(resp.,
${\mathsf{Irr}}(X)$
(resp.,
![]() ${\mathsf{Irr}}_c(X)$
) the set of all irreducible (resp., irreducible closed) subsets of
${\mathsf{Irr}}_c(X)$
) the set of all irreducible (resp., irreducible closed) subsets of
![]() $X$
. Clearly, every subset of
$X$
. Clearly, every subset of
![]() $X$
that is directed under
$X$
that is directed under
![]() $\leq _X$
is irreducible.
$\leq _X$
is irreducible.
Remark 21.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space and
$T_0$
-space and
![]() $A$
a nonempty subset of
$A$
a nonempty subset of
![]() $X$
. Then
$X$
. Then
![]() $A\in{\mathsf{Irr}} (X)$
iff for any
$A\in{\mathsf{Irr}} (X)$
iff for any
![]() $U, V\in \mathscr{O}(X)$
,
$U, V\in \mathscr{O}(X)$
,
![]() $A\cap U\neq \emptyset$
and
$A\cap U\neq \emptyset$
and
![]() $A\cap V\neq \emptyset$
imply
$A\cap V\neq \emptyset$
imply
![]() $A\cap U\cap V\neq \emptyset$
.
$A\cap U\cap V\neq \emptyset$
.
A topological space
![]() $X$
is called sober, if for any
$X$
is called sober, if for any
![]() $A\in{\mathsf{Irr}}_c(X)$
, there is a unique point
$A\in{\mathsf{Irr}}_c(X)$
, there is a unique point
![]() $x\in X$
such that
$x\in X$
such that
![]() $A=\overline{\{x\}}$
. It is straightforward to verify that every sober space is a
$A=\overline{\{x\}}$
. It is straightforward to verify that every sober space is a
![]() $d$
-space (cf. Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003). For simplicity, if a dcpo has a sober (resp., non-sober) Scott topology, then we will call
$d$
-space (cf. Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003). For simplicity, if a dcpo has a sober (resp., non-sober) Scott topology, then we will call
![]() $P$
a sober (resp., non-sober) dcpo.
$P$
a sober (resp., non-sober) dcpo.
The following result is well-known.
Proposition 22.
(Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003
, Proposition III-3.7) (Gierz et al. Reference Gierz, Lawson and Stralka1983
, Proposition 4.4) For a quasicontinuous domain (especially, a continuous domain)
![]() $P$
,
$P$
,
![]() $\Sigma \,\!\! P$
is sober.
$\Sigma \,\!\! P$
is sober.
For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
, we shall use
$X$
, we shall use
![]() $\mathord{\mathsf{K}}(X)$
to denote the set of all nonempty compact saturated subsets of
$\mathord{\mathsf{K}}(X)$
to denote the set of all nonempty compact saturated subsets of
![]() $X$
and endow it with the Smyth order
$X$
and endow it with the Smyth order
![]() $\sqsubseteq$
, that is, for
$\sqsubseteq$
, that is, for
![]() $K_1,K_2\in \mathord{\mathsf{K}}(X)$
,
$K_1,K_2\in \mathord{\mathsf{K}}(X)$
,
![]() $K_1\sqsubseteq K_2$
iff
$K_1\sqsubseteq K_2$
iff
![]() $K_2\subseteq K_1$
. Let
$K_2\subseteq K_1$
. Let
![]() $\mathscr{S}^u(X)=\{\mathord{\uparrow } x : x\in X\}$
and
$\mathscr{S}^u(X)=\{\mathord{\uparrow } x : x\in X\}$
and
![]() $\mathscr{S}^u_2(X)=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in X\}$
. Obviously,
$\mathscr{S}^u_2(X)=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in X\}$
. Obviously,
![]() $\mathscr{S}^u(X)\subseteq \mathscr{S}^u_2(X)$
. The space
$\mathscr{S}^u(X)\subseteq \mathscr{S}^u_2(X)$
. The space
![]() $X$
is called well-filtered if it is
$X$
is called well-filtered if it is
![]() $T_0$
, and for any open set
$T_0$
, and for any open set
![]() $U$
and filtered family
$U$
and filtered family
![]() $\mathscr{K}\subseteq \mathord{\mathsf{K}}(X)$
,
$\mathscr{K}\subseteq \mathord{\mathsf{K}}(X)$
,
![]() $\bigcap \mathscr{K}{\subseteq } U$
implies
$\bigcap \mathscr{K}{\subseteq } U$
implies
![]() $K{\subseteq } U$
for some
$K{\subseteq } U$
for some
![]() $K{\in }\mathscr{K}$
. It is called coherent if the intersection of any two compact saturated sets of
$K{\in }\mathscr{K}$
. It is called coherent if the intersection of any two compact saturated sets of
![]() $X$
is compact.
$X$
is compact.
Lemma 23.
(Xu et al. Reference Xu, Shen, Xi and Zhao2021
, Lemma 2.6) Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space. For any nonempty family
$T_0$
-space. For any nonempty family
![]() $\{K_i : i\in I\}\subseteq \mathord{\mathsf{K}} (X)$
,
$\{K_i : i\in I\}\subseteq \mathord{\mathsf{K}} (X)$
,
![]() $\bigvee _{i\in I} K_i$
exists in
$\bigvee _{i\in I} K_i$
exists in
![]() $\mathord{\mathsf{K}} (X)$
iff
$\mathord{\mathsf{K}} (X)$
iff
![]() $\bigcap _{i\in I} K_i\in \mathord{\mathsf{K}} (X)$
. In this case
$\bigcap _{i\in I} K_i\in \mathord{\mathsf{K}} (X)$
. In this case
![]() $\bigvee _{i\in I} K_i=\bigcap _{i\in I} K_i$
.
$\bigvee _{i\in I} K_i=\bigcap _{i\in I} K_i$
.
The following result is well-known (see, e.g., Xi and Zhao Reference Xi and Zhao2017, Proposition 3 or Xu et al. Reference Xu, Shen, Xi and Zhao2020b, Lemma 2.6).
Lemma 24.
For a well-filtered space
![]() $X$
,
$X$
,
![]() $\mathord{\mathsf{K}} (X)$
is a dcpo.
$\mathord{\mathsf{K}} (X)$
is a dcpo.
For a dcpo with well-filtered Scott topology, Jia et al. (2018) gave the following useful characterization of coherence of its Scott space.
Lemma 25.
(Jia Reference Jia2018
, Lemma 3.1) Let
![]() $P$
be a dcpo for which
$P$
be a dcpo for which
![]() $\Sigma P$
is well-filtered. Then the following three conditions are equivalent:
$\Sigma P$
is well-filtered. Then the following three conditions are equivalent:
-
(1)
 $\Sigma P$
is coherent.
$\Sigma P$
is coherent.
-
(2)
 $\mathord{\uparrow }x_{1}\cap \mathord{\uparrow }x_{2}\cap \ldots \cap \mathord{\uparrow }x_{n}$
is Scott-compact for all finite nonempty set
$\mathord{\uparrow }x_{1}\cap \mathord{\uparrow }x_{2}\cap \ldots \cap \mathord{\uparrow }x_{n}$
is Scott-compact for all finite nonempty set
 $\{x_1, x_2, \ldots, x_n\}$
of
$\{x_1, x_2, \ldots, x_n\}$
of
 $P$
.
$P$
. -
(3)
 $\uparrow x \cap \uparrow y$
is Scott-compact for all
$\uparrow x \cap \uparrow y$
is Scott-compact for all
 $x, y\in P$
.
$x, y\in P$
.
It is well-known that every sober space is well-filtered and every well-filtered space is a
![]() $d$
-space. Kou (Reference Kou2001) gave the first example of a dcpo whose Scott space is well-filtered but non-sober. Another simpler dcpo whose Scott topology is well-filtered but not sober was presented in Zhao et al. (Reference Zhao, Xi and Chen2019). Jia (Reference Jia2018) constructed a countable infinite dcpo whose Scott topology is well-filtered but non-sober. It is worth noting that Johnstone (Reference Johnstone1981) constructed the first example of a dcpo whose Scott space is non-sober (indeed, it is not well-filtered) and Isbell (Reference Isbell1982) constructed a complete lattice whose Scott space is non-sober. Xi and Lawson (Reference Xi and Lawson2017) showed that every complete lattice is well-filtered in its Scott topology.
$d$
-space. Kou (Reference Kou2001) gave the first example of a dcpo whose Scott space is well-filtered but non-sober. Another simpler dcpo whose Scott topology is well-filtered but not sober was presented in Zhao et al. (Reference Zhao, Xi and Chen2019). Jia (Reference Jia2018) constructed a countable infinite dcpo whose Scott topology is well-filtered but non-sober. It is worth noting that Johnstone (Reference Johnstone1981) constructed the first example of a dcpo whose Scott space is non-sober (indeed, it is not well-filtered) and Isbell (Reference Isbell1982) constructed a complete lattice whose Scott space is non-sober. Xi and Lawson (Reference Xi and Lawson2017) showed that every complete lattice is well-filtered in its Scott topology.
Proposition 26.
(Xi and Lawson Reference Xi and Lawson2017, Proposition 2.4) Let
![]() $X$
be a
$X$
be a
![]() $d$
-space such that
$d$
-space such that
![]() $\downarrow (A\cap K)$
is closed for all
$\downarrow (A\cap K)$
is closed for all
![]() $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
![]() $K\in \mathord{\mathsf{K}} (X)$
. Then
$K\in \mathord{\mathsf{K}} (X)$
. Then
![]() $X$
is well-filtered.
$X$
is well-filtered.
Proposition 27.
(Xi and Lawson Reference Xi and Lawson2017, Proposition 3.1 and Corollary 3.2) For a dcpo
![]() $P$
, if
$P$
, if
![]() $(P, \lambda (P))$
is upper-semicompact (in particular, if
$(P, \lambda (P))$
is upper-semicompact (in particular, if
![]() $(P, \lambda (P))$
is compact or
$(P, \lambda (P))$
is compact or
![]() $P$
is a complete lattice), then
$P$
is a complete lattice), then
![]() $(P, \sigma (P))$
is well-filtered.
$(P, \sigma (P))$
is well-filtered.
The following result is well-known (see Gierz et al. Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003, Kou Reference Kou2001).
Theorem 28.
For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
, the following conditions are equivalent:
$X$
, the following conditions are equivalent:
-
(1)
 $X$
is locally compact and sober.
$X$
is locally compact and sober.
-
(2)
 $X$
is locally compact and well-filtered.
$X$
is locally compact and well-filtered.
-
(3)
 $X$
is core-compact and sober.
$X$
is core-compact and sober.
The above result was improved in Lawson et al. (Reference Lawson, Wu and Xi2020) and Xu et al. (Reference Xu, Shen, Xi and Zhao2020b) by two different methods.
Theorem 29. (Lawson et al. Reference Lawson, Wu and Xi2020 , Theorem 3.1) (Xu et al. Reference Xu, Shen, Xi and Zhao2020b, Theorem 6.16) Every core-compact well-filtered space is sober.
For any topological space
![]() $X$
,
$X$
,
![]() $\mathscr{G}\subseteq 2^{X}$
and
$\mathscr{G}\subseteq 2^{X}$
and
![]() $A\subseteq X$
, let
$A\subseteq X$
, let
![]() $\Diamond _{\mathscr{G}} A=\{G\in \mathscr{G} : G\cap A\neq \emptyset \}$
and
$\Diamond _{\mathscr{G}} A=\{G\in \mathscr{G} : G\cap A\neq \emptyset \}$
and
![]() $\Box _{\mathscr{G}} A=\{G\in \mathscr{G} : G\subseteq A\}$
. The symbols
$\Box _{\mathscr{G}} A=\{G\in \mathscr{G} : G\subseteq A\}$
. The symbols
![]() $\Diamond _{\mathscr{G}} A$
and
$\Diamond _{\mathscr{G}} A$
and
![]() $\Box _{\mathscr{G}} A$
will be simply written as
$\Box _{\mathscr{G}} A$
will be simply written as
![]() $\Diamond A$
and
$\Diamond A$
and
![]() $\Box A$
respectively if there is no ambiguity. The upper Vietoris topology on
$\Box A$
respectively if there is no ambiguity. The upper Vietoris topology on
![]() $\mathscr{G}$
is the topology that has
$\mathscr{G}$
is the topology that has
![]() $\{\Box _{\mathscr{G}} U : U\in \mathscr{O}(X)\}$
as a base, and the resulting space is denoted by
$\{\Box _{\mathscr{G}} U : U\in \mathscr{O}(X)\}$
as a base, and the resulting space is denoted by
![]() $P_S(\mathscr{G})$
.
$P_S(\mathscr{G})$
.
The space
![]() $P_S(\mathord{\mathsf{K}}(X))$
, denoted briefly by
$P_S(\mathord{\mathsf{K}}(X))$
, denoted briefly by
![]() $P_S(X)$
, is called the Smyth power space or upper space of
$P_S(X)$
, is called the Smyth power space or upper space of
![]() $X$
(cf. Heckmann Reference Heckmann1992; Schalk Reference Schalk1993). It is easy to verify that the specialization order of
$X$
(cf. Heckmann Reference Heckmann1992; Schalk Reference Schalk1993). It is easy to verify that the specialization order of
![]() $P_S(X)$
is the Smyth order (i.e.,,
$P_S(X)$
is the Smyth order (i.e.,,
![]() $\leq _{P_S(X)}=\sqsupseteq$
). The canonical mapping
$\leq _{P_S(X)}=\sqsupseteq$
). The canonical mapping
![]() $\xi _X: X\longrightarrow P_S(X)$
,
$\xi _X: X\longrightarrow P_S(X)$
,
![]() $x\mapsto \mathord{\uparrow } x$
, is a topological embedding (cf. Heckmann Reference Heckmann1992; Heckmann and Keimel Reference Heckmann and Keimel2013; Schalk Reference Schalk1993).
$x\mapsto \mathord{\uparrow } x$
, is a topological embedding (cf. Heckmann Reference Heckmann1992; Heckmann and Keimel Reference Heckmann and Keimel2013; Schalk Reference Schalk1993).
3. Property R and
 $\Omega ^*$
-Compactness
$\Omega ^*$
-Compactness
In this section, we consider
![]() $T_0$
-spaces and posets equipped with the Scott topology and equip them also with the lower topology denoted by
$T_0$
-spaces and posets equipped with the Scott topology and equip them also with the lower topology denoted by
![]() $\omega$
. We study in particular property R and the property of
$\omega$
. We study in particular property R and the property of
![]() $\Omega ^*$
-compactness, the equivalence between them, and their main properties.
$\Omega ^*$
-compactness, the equivalence between them, and their main properties.
Definition 30.
A
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is said to have property R if for any family
$X$
is said to have property R if for any family
![]() $\{\mathord{\uparrow }F_{i}:i\in I\}\subseteq \mathbf{Fin} P$
and any
$\{\mathord{\uparrow }F_{i}:i\in I\}\subseteq \mathbf{Fin} P$
and any
![]() $U\in \mathscr{O}(X)$
,
$U\in \mathscr{O}(X)$
,
![]() $\bigcap _{i\in I}\mathord{\uparrow }F_{i}\subseteq U$
implies
$\bigcap _{i\in I}\mathord{\uparrow }F_{i}\subseteq U$
implies
![]() $\bigcap _{i\in I_{0}}\mathord{\uparrow }F_{i}\subseteq U$
for some
$\bigcap _{i\in I_{0}}\mathord{\uparrow }F_{i}\subseteq U$
for some
![]() $I_{0}\in I^{(\lt \omega )}$
. For a poset
$I_{0}\in I^{(\lt \omega )}$
. For a poset
![]() $P$
, when
$P$
, when
![]() $\Sigma P$
has property R, we will simply say that
$\Sigma P$
has property R, we will simply say that
![]() $P$
has property R.
$P$
has property R.
The property R was first introduced in Xu (Reference Xu2016a, Definition 10.2.11) (see also Wen and Xu Reference Wen and Xu2018). Clearly, every
![]() $T_1$
-space has property R and the Sierpiński space
$T_1$
-space has property R and the Sierpiński space
![]() $\Sigma 2$
has property R. We are particularly interested in the conditions under which a
$\Sigma 2$
has property R. We are particularly interested in the conditions under which a
![]() $T_0$
-space or a poset equipped with the Scott topology has property R.
$T_0$
-space or a poset equipped with the Scott topology has property R.
We next recall the definition of
![]() $\Omega ^*$
-compactness from Lawson et al. (Reference Lawson, Wu and Xi2020).
$\Omega ^*$
-compactness from Lawson et al. (Reference Lawson, Wu and Xi2020).
Definition 31.
(Lawson et al. Reference Lawson, Wu and Xi2020
, Definition 5.1) A
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is said to be
$X$
is said to be
![]() $\Omega ^{\ast }$
-compact if every closed subset of
$\Omega ^{\ast }$
-compact if every closed subset of
![]() $X$
is compact in
$X$
is compact in
![]() $(X, \omega (X))$
.
$(X, \omega (X))$
.
And lastly, we introduce a new concept.
Definition 32.
A
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is well-filtered with respect to the family of
$X$
is well-filtered with respect to the family of
![]() $\omega$
-closed sets if any filtered family
$\omega$
-closed sets if any filtered family
![]() $\mathscr{D}$
of
$\mathscr{D}$
of
![]() $\omega$
-closed sets has intersection contained in an open subset
$\omega$
-closed sets has intersection contained in an open subset
![]() $U$
of
$U$
of
![]() $X$
, then some member of
$X$
, then some member of
![]() $\mathscr{D}$
is contained in
$\mathscr{D}$
is contained in
![]() $U$
. A poset
$U$
. A poset
![]() $P$
has this property if the space
$P$
has this property if the space
![]() $\Sigma$
P has it.
$\Sigma$
P has it.
Proposition 33.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space.
$T_0$
-space.
-
(1) If
 $X$
is a
$X$
is a
 $d$
-space and
$d$
-space and
 $\mathord{\uparrow } x\cap \mathord{\uparrow } y\in \mathbf{Fin} X\cup \{\emptyset \}$
for all
$\mathord{\uparrow } x\cap \mathord{\uparrow } y\in \mathbf{Fin} X\cup \{\emptyset \}$
for all
 $x, y\in X$
, then
$x, y\in X$
, then
 $X$
has property R
$X$
has property R
-
(2) If
 $X$
is well-filtered and
$X$
is well-filtered and
 $\bigcap _{u\in F}\mathord{\uparrow } u$
is compact for all
$\bigcap _{u\in F}\mathord{\uparrow } u$
is compact for all
 $F\in P^{(\lt \omega )}$
(especially, if
$F\in P^{(\lt \omega )}$
(especially, if
 $X$
is well-filtered and coherent), then
$X$
is well-filtered and coherent), then
 $X$
has property R.
$X$
has property R.
Proof. We prove (1) and (2) in a uniform manner. Suppose that
![]() $\{x_{i}:i\in I\}\subseteq X$
and
$\{x_{i}:i\in I\}\subseteq X$
and
![]() $U\in \mathscr{O}(X)$
such that
$U\in \mathscr{O}(X)$
such that
![]() $\bigcap _{i\in I}\mathord{\uparrow }x_{i}\subseteq U$
. For each
$\bigcap _{i\in I}\mathord{\uparrow }x_{i}\subseteq U$
. For each
![]() $J\in I^{(\lt \omega )}$
, let
$J\in I^{(\lt \omega )}$
, let
![]() $G_{J}=\bigcap _{i\in J}\mathord{\uparrow } x_{i}$
. If there is
$G_{J}=\bigcap _{i\in J}\mathord{\uparrow } x_{i}$
. If there is
![]() $J_0\in I^{(\lt \omega )}$
such that
$J_0\in I^{(\lt \omega )}$
such that
![]() $G_{J_0}=\emptyset$
, then
$G_{J_0}=\emptyset$
, then
![]() $\bigcap _{i\in J_0}\mathord{\uparrow } x_{i}\subseteq U$
. Now we assume that
$\bigcap _{i\in J_0}\mathord{\uparrow } x_{i}\subseteq U$
. Now we assume that
![]() $G_{J}\neq \emptyset$
for all
$G_{J}\neq \emptyset$
for all
![]() $J\in I^{(\lt \omega )}$
.
$J\in I^{(\lt \omega )}$
.
(1): Assume that
![]() $X$
is a
$X$
is a
![]() $d$
-space and
$d$
-space and
![]() $\mathord{\uparrow } x\cap \mathord{\uparrow } y\in \mathbf{Fin} X\cup \{\emptyset \}$
for all
$\mathord{\uparrow } x\cap \mathord{\uparrow } y\in \mathbf{Fin} X\cup \{\emptyset \}$
for all
![]() $x, y\in X$
. Then
$x, y\in X$
. Then
![]() $\{G_J : J\in I^{(\lt \omega )}\}\subseteq \mathbf{Fin} X$
and it is a filtered family. Since
$\{G_J : J\in I^{(\lt \omega )}\}\subseteq \mathbf{Fin} X$
and it is a filtered family. Since
![]() $X$
is a
$X$
is a
![]() $d$
-space,
$d$
-space,
![]() $X$
(with the specialization order) is a dcpo and
$X$
(with the specialization order) is a dcpo and
![]() $U\in \mathscr{O}(X)\subseteq \sigma (X)$
. Clearly,
$U\in \mathscr{O}(X)\subseteq \sigma (X)$
. Clearly,
![]() $\bigcap _{J\in I^{(\lt \omega )}}G_J=\bigcap _{i\in I}\mathord{\uparrow }x_{i}\subseteq U$
. By Corollary 9,
$\bigcap _{J\in I^{(\lt \omega )}}G_J=\bigcap _{i\in I}\mathord{\uparrow }x_{i}\subseteq U$
. By Corollary 9,
![]() $G_J \subseteq U$
for some
$G_J \subseteq U$
for some
![]() $J\in I^{(\lt \omega )}$
. Thus,
$J\in I^{(\lt \omega )}$
. Thus,
![]() $X$
has property R.
$X$
has property R.
(2): Assume that
![]() $X$
is well-filtered and
$X$
is well-filtered and
![]() $\bigcap _{u\in F}\mathord{\uparrow } u$
is compact for all
$\bigcap _{u\in F}\mathord{\uparrow } u$
is compact for all
![]() $F\in P^{(\lt \omega )}$
. Then
$F\in P^{(\lt \omega )}$
. Then
![]() $\{G_J : J\in I^{(\lt \omega )}\}$
is a filtered family of compact saturated subsets of
$\{G_J : J\in I^{(\lt \omega )}\}$
is a filtered family of compact saturated subsets of
![]() $X$
and
$X$
and
![]() $\bigcap \limits _{J\in I^{(\lt \omega )}}G_J= \bigcap _{i\in I}\mathord{\uparrow }x_{i}\subseteq U$
. By the well-filteredness of
$\bigcap \limits _{J\in I^{(\lt \omega )}}G_J= \bigcap _{i\in I}\mathord{\uparrow }x_{i}\subseteq U$
. By the well-filteredness of
![]() $X$
, there is
$X$
, there is
![]() $J^{\prime }\in I^{(\lt \omega )}$
such that
$J^{\prime }\in I^{(\lt \omega )}$
such that
![]() $\bigcap _{i\in J^{\prime }}\mathord{\uparrow } x_{i}=G_{J^{\prime }}\subseteq U$
, proving the property R of
$\bigcap _{i\in J^{\prime }}\mathord{\uparrow } x_{i}=G_{J^{\prime }}\subseteq U$
, proving the property R of
![]() $X$
.
$X$
.
Corollary 34.
For a
![]() $d$
-space
$d$
-space
![]() $X$
, if
$X$
, if
![]() $X$
(with the specialization order) is a sup semilattice (especially,
$X$
(with the specialization order) is a sup semilattice (especially,
![]() $X$
is a complete lattice), then
$X$
is a complete lattice), then
![]() $X$
has property R. In particular, for any complete lattice
$X$
has property R. In particular, for any complete lattice
![]() $L$
, the Scott space
$L$
, the Scott space
![]() $\Sigma L$
has property R.
$\Sigma L$
has property R.
In what follows, we will be working with the lattice of closed sets of the lower topology on a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
resp. poset
$X$
resp. poset
![]() $P$
, which we denote by
$P$
, which we denote by
![]() $\omega ^*(X)$
resp.
$\omega ^*(X)$
resp.
![]() $\omega ^*(P)$
. The following is a key theorem.
$\omega ^*(P)$
. The following is a key theorem.
Theorem 35.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space and
$T_0$
-space and
![]() $\mathscr{Q}=(\omega ^{*}(X),\supseteq )$
. Then the following conditions are equivalent:
$\mathscr{Q}=(\omega ^{*}(X),\supseteq )$
. Then the following conditions are equivalent:
-
(1)
 $X$
is well-filtered with respect to the family of
$X$
is well-filtered with respect to the family of
 $\omega$
-closed sets.
$\omega$
-closed sets.
-
(2)
 $X$
satisfies property R.
$X$
satisfies property R.
-
(3)
 $X$
is
$X$
is
 $\Omega ^*$
-compact, that is all closed subspaces of
$\Omega ^*$
-compact, that is all closed subspaces of
 $X$
are compact in the
$X$
are compact in the
 $\omega$
-topology.
$\omega$
-topology.
-
(4) For any subset
 $S$
of
$S$
of
 $X$
and any open set
$X$
and any open set
 $U$
,
$U$
,
 $\bigcap \{\mathord{\uparrow } x: x\in S\}\subseteq U$
implies there exists a finite subset
$\bigcap \{\mathord{\uparrow } x: x\in S\}\subseteq U$
implies there exists a finite subset
 $S_0$
of
$S_0$
of
 $S$
such that
$S$
such that
 $\bigcap \{\mathord{\uparrow } x: x\in S_0\}\subseteq U$
.
$\bigcap \{\mathord{\uparrow } x: x\in S_0\}\subseteq U$
. -
(5) Every basic open set
 $\Box U$
in the upper Vietoris topology on
$\Box U$
in the upper Vietoris topology on
 $\omega ^*(X)$
belongs to the Scott topology of
$\omega ^*(X)$
belongs to the Scott topology of
 $Q$
.
$Q$
.
Proof. (1)
![]() $\Rightarrow$
(2): Let
$\Rightarrow$
(2): Let
![]() $U$
be an open subset of
$U$
be an open subset of
![]() $X$
and let
$X$
and let
![]() $\{\mathord{\uparrow } F_{i}:i\in I\}\subseteq \mathbf{Fin} X$
satisfy
$\{\mathord{\uparrow } F_{i}:i\in I\}\subseteq \mathbf{Fin} X$
satisfy
As
![]() $I_0$
varies over the finite subsets of
$I_0$
varies over the finite subsets of
![]() $I$
, the sets
$I$
, the sets
![]() $\bigcap _{i\in I_0} \uparrow F_i$
form a filtered family of closed sets in the
$\bigcap _{i\in I_0} \uparrow F_i$
form a filtered family of closed sets in the
![]() $\omega$
-topology with intersection
$\omega$
-topology with intersection
![]() $C$
. By the hypothesized well-filtering property (1), one of these sets must be contained in
$C$
. By the hypothesized well-filtering property (1), one of these sets must be contained in
![]() $U$
, and thus property R holds.
$U$
, and thus property R holds.
(2)
![]() $\Rightarrow$
(1): Let
$\Rightarrow$
(1): Let
![]() $U$
be an open subset of
$U$
be an open subset of
![]() $X$
and let
$X$
and let
![]() $\mathscr{A}$
be a filtered family of sets closed in the
$\mathscr{A}$
be a filtered family of sets closed in the
![]() $\omega$
-topology such that
$\omega$
-topology such that
![]() $\bigcap _{A\in \mathscr{A}} A\subseteq U$
. Consider the family
$\bigcap _{A\in \mathscr{A}} A\subseteq U$
. Consider the family
Each
![]() $A\in \mathscr{A}$
is the intersection of members of
$A\in \mathscr{A}$
is the intersection of members of
![]() $\mathscr{F}$
since the sets
$\mathscr{F}$
since the sets
![]() $\mathord{\uparrow } F$
,
$\mathord{\uparrow } F$
,
![]() $F$
finite, form a basis for the closed sets of the
$F$
finite, form a basis for the closed sets of the
![]() $\omega$
-topology, and thus
$\omega$
-topology, and thus
![]() $\bigcap \mathscr{F}=\bigcap \mathscr{A}\subseteq U$
. By property R there exists finitely many members
$\bigcap \mathscr{F}=\bigcap \mathscr{A}\subseteq U$
. By property R there exists finitely many members
![]() $\mathord{\uparrow } F_1,\ldots, \mathord{\uparrow } F_n$
of
$\mathord{\uparrow } F_1,\ldots, \mathord{\uparrow } F_n$
of
![]() $\mathscr{F}$
such that
$\mathscr{F}$
such that
![]() $\bigcap _{i=1}^n \mathord{\uparrow } F_i\subseteq U$
. By choice of the
$\bigcap _{i=1}^n \mathord{\uparrow } F_i\subseteq U$
. By choice of the
![]() $F_i$
we may pick for each
$F_i$
we may pick for each
![]() $i$
some
$i$
some
![]() $A_i\in \mathscr{A}$
such that
$A_i\in \mathscr{A}$
such that
![]() $A_i\subseteq \mathord{\uparrow } F_i$
. By the filteredness of
$A_i\subseteq \mathord{\uparrow } F_i$
. By the filteredness of
![]() $\mathscr{A}$
, we may pick
$\mathscr{A}$
, we may pick
![]() $A\in \mathscr{A}$
such that
$A\in \mathscr{A}$
such that
![]() $A\subseteq \bigcap _{i=1}^n A_i$
. Then
$A\subseteq \bigcap _{i=1}^n A_i$
. Then
![]() $A\subseteq U$
, and hence the well-filtering property is established.
$A\subseteq U$
, and hence the well-filtering property is established.
(2)
![]() $\Leftrightarrow$
(3): The complements of the sets
$\Leftrightarrow$
(3): The complements of the sets
![]() $\mathord{\uparrow } F$
,
$\mathord{\uparrow } F$
,
![]() $F$
finite, form a basis for the
$F$
finite, form a basis for the
![]() $\omega$
-topology. By taking compliments, we can read property R to say any open cover of a closed set
$\omega$
-topology. By taking compliments, we can read property R to say any open cover of a closed set
![]() $A=X\setminus U$
by such basic open sets has a finite subcover. Equivalently by the Alexander Subbasis Theorem, the closed set
$A=X\setminus U$
by such basic open sets has a finite subcover. Equivalently by the Alexander Subbasis Theorem, the closed set
![]() $A$
is compact in the
$A$
is compact in the
![]() $\omega$
-topology.
$\omega$
-topology.
(2)
![]() $\Leftrightarrow$
(4): The property of (4) is essentially property R restricted to sets of the form
$\Leftrightarrow$
(4): The property of (4) is essentially property R restricted to sets of the form
![]() $\mathord{\uparrow } x$
instead of
$\mathord{\uparrow } x$
instead of
![]() $\mathord{\uparrow } F$
,
$\mathord{\uparrow } F$
,
![]() $F$
finite. These sets form a subbasis for the
$F$
finite. These sets form a subbasis for the
![]() $\omega$
-closed sets, so the proof follows along the lines of (2)
$\omega$
-closed sets, so the proof follows along the lines of (2)
![]() $\Leftrightarrow$
(3).
$\Leftrightarrow$
(3).
(1)
![]() $\Rightarrow$
(5): It follows directly from (1) that any basic open set
$\Rightarrow$
(5): It follows directly from (1) that any basic open set
![]() $\Box U$
in the upper Vietoris topology on
$\Box U$
in the upper Vietoris topology on
![]() $\omega ^*(X)$
is Scott-open in the lattice
$\omega ^*(X)$
is Scott-open in the lattice
![]() $\mathscr{Q}$
.
$\mathscr{Q}$
.
(5)
![]() $\Rightarrow$
(2): It is straightforward to deduce property R from the hypothesis that each
$\Rightarrow$
(2): It is straightforward to deduce property R from the hypothesis that each
![]() $\Box U$
is Scott-open in the lattice
$\Box U$
is Scott-open in the lattice
![]() $\mathscr{Q}$
.
$\mathscr{Q}$
.
We can enhance the preceding slightly if we are working with dcpos equipped with the Scott topology.
Corollary 36.
A dcpo
![]() $L$
endowed with the Scott topology satisfies any
$L$
endowed with the Scott topology satisfies any
![]() $($
and hence all
$($
and hence all
![]() $)$
of the preceding five properties if and only if the Scott-closed sets of
$)$
of the preceding five properties if and only if the Scott-closed sets of
![]() $L$
are precisely the saturated compact sets for the lower topology.
$L$
are precisely the saturated compact sets for the lower topology.
Proof. Assume
![]() $L$
is
$L$
is
![]() $\Omega ^*$
-compact. Then, the Scott-closed sets are compact with respect to the lower topology and they are saturated since that are lower sets. Conversely let
$\Omega ^*$
-compact. Then, the Scott-closed sets are compact with respect to the lower topology and they are saturated since that are lower sets. Conversely let
![]() $A=\mathord{\downarrow } A$
be
$A=\mathord{\downarrow } A$
be
![]() $\omega$
-compact. Let
$\omega$
-compact. Let
![]() $D$
be a directed subset of
$D$
be a directed subset of
![]() $A$
with supremum
$A$
with supremum
![]() $e$
. Then,
$e$
. Then,
![]() $\{\mathord{\uparrow } d: d\in D\}$
is a filtered family of
$\{\mathord{\uparrow } d: d\in D\}$
is a filtered family of
![]() $\omega$
-closed sets each of which meets the
$\omega$
-closed sets each of which meets the
![]() $\omega$
-compact set
$\omega$
-compact set
![]() $A$
, and hence, their intersection must contain some
$A$
, and hence, their intersection must contain some
![]() $y\in A$
, which must be an upper bound for
$y\in A$
, which must be an upper bound for
![]() $D$
. Thus,
$D$
. Thus,
![]() $\sup D\leq y\in A=\mathord{\downarrow } A$
. Thus,
$\sup D\leq y\in A=\mathord{\downarrow } A$
. Thus,
![]() $\sup D\in A$
, and we see that
$\sup D\in A$
, and we see that
![]() $A$
is Scott-closed.
$A$
is Scott-closed.
Recall that for a topological space
![]() $(X, \tau )$
, the de Groot dual
$(X, \tau )$
, the de Groot dual
![]() $\tau ^d$
of
$\tau ^d$
of
![]() $\tau$
is defined by taking as a subbasis for the closed sets all compact saturated sets in
$\tau$
is defined by taking as a subbasis for the closed sets all compact saturated sets in
![]() $(X, \tau )$
. The patch topology
$(X, \tau )$
. The patch topology
![]() $\tau ^{\sharp }$
on
$\tau ^{\sharp }$
on
![]() $X$
is the coarsest topology that is finer than the original topology
$X$
is the coarsest topology that is finer than the original topology
![]() $\tau$
and its de Groot dual
$\tau$
and its de Groot dual
![]() $\tau ^d$
, namely,
$\tau ^d$
, namely,
![]() $\tau ^{\sharp }=\tau \bigvee \tau ^d$
.
$\tau ^{\sharp }=\tau \bigvee \tau ^d$
.
Remark 37.
The preceding corollary shows for a dcpo
![]() $L$
equipped with Scott topology and satisfying any of the conditions of Theorem
35
that
$L$
equipped with Scott topology and satisfying any of the conditions of Theorem
35
that
![]() $\omega (L)^d=\sigma (L)$
and hence
$\omega (L)^d=\sigma (L)$
and hence
![]() $\lambda (L)=\omega (L)^{\sharp }$
.
$\lambda (L)=\omega (L)^{\sharp }$
.
See Example 56 of the next section for an example of the Scott space of a dcpo (indeed an algebraic domain) that does not satisfy property R (and hence the equivalent properties).
Remark 38.
It will be convenient to have a short name for the
![]() $T_0$
-spaces that satisfy the previous five equivalent properties. For the purposes of this paper, we refer to them as
$T_0$
-spaces that satisfy the previous five equivalent properties. For the purposes of this paper, we refer to them as
![]() $R$
-spaces. Let
$R$
-spaces. Let
![]() $\mathbf{Top}_r$
denote the category of all R-spaces and continuous mappings.
$\mathbf{Top}_r$
denote the category of all R-spaces and continuous mappings.
The following corollary follows directly from Proposition 20 and the Theorem35(4) by taking directed sets for
![]() $S$
.
$S$
.
Corollary 39.
An
![]() $R$
-space is a
$R$
-space is a
![]() $d$
-space.
$d$
-space.
A significant question in the study of
![]() $T_0$
-spaces equipped with the lower topology is the identification of useful conditions for the Lawson topology induced by the given topology, the topology generated by the given topology and the lower topology, to be compact. Using property R we can specify necessary and sufficient conditions.
$T_0$
-spaces equipped with the lower topology is the identification of useful conditions for the Lawson topology induced by the given topology, the topology generated by the given topology and the lower topology, to be compact. Using property R we can specify necessary and sufficient conditions.
Proposition 40.
For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
, the following are equivalent:
$X$
, the following are equivalent:
-
(1) The joint topology
 $\mathscr{O}(X)\bigvee \omega (X)$
is compact.
$\mathscr{O}(X)\bigvee \omega (X)$
is compact.
-
(2)
 $X$
is a compact
$X$
is a compact
 $R$
-space and
$R$
-space and
 $\bigcap \{\mathord{\uparrow } x: x\in F\}$
is compact for all finite subsets
$\bigcap \{\mathord{\uparrow } x: x\in F\}$
is compact for all finite subsets
 $F$
of
$F$
of
 $X$
.
$X$
.
Proof. (1)
![]() $\Rightarrow$
(2): As the joint topology
$\Rightarrow$
(2): As the joint topology
![]() $\mathscr{O}(X)\bigvee \omega (X)$
is compact,
$\mathscr{O}(X)\bigvee \omega (X)$
is compact,
![]() $X$
is a compact space. Each
$X$
is a compact space. Each
![]() $\bigcap \{\mathord{\uparrow } x: x\in F\}$
for
$\bigcap \{\mathord{\uparrow } x: x\in F\}$
for
![]() $F$
finite is closed in the lower topology, hence closed in the join
$F$
finite is closed in the lower topology, hence closed in the join
![]() $\mathscr{O}(X)\bigvee \omega (X)$
and thus compact in
$\mathscr{O}(X)\bigvee \omega (X)$
and thus compact in
![]() $\mathscr{O}(X)\bigvee \omega (X)$
since the joint topology
$\mathscr{O}(X)\bigvee \omega (X)$
since the joint topology
![]() $\mathscr{O}(X)\bigvee \omega (X)$
is compact by hypothesis. But then it is certainly compact in the weaker topology of
$\mathscr{O}(X)\bigvee \omega (X)$
is compact by hypothesis. But then it is certainly compact in the weaker topology of
![]() $X$
. Every closed subset
$X$
. Every closed subset
![]() $A$
of
$A$
of
![]() $X$
in its given topology is closed in
$X$
in its given topology is closed in
![]() $\mathscr{O}(X)\bigvee \omega (X)$
, whence compact in
$\mathscr{O}(X)\bigvee \omega (X)$
, whence compact in
![]() $\mathscr{O}(X)\bigvee \omega (X)$
, and hence compact in the lower topology. Thus,
$\mathscr{O}(X)\bigvee \omega (X)$
, and hence compact in the lower topology. Thus,
![]() $X$
is
$X$
is
![]() $\Omega ^*$
-compact and hence satisfies the equivalent property R by Theorem35.
$\Omega ^*$
-compact and hence satisfies the equivalent property R by Theorem35.
(2)
![]() $\Rightarrow$
(1): We assume
$\Rightarrow$
(1): We assume
![]() $X$
is covered by an open covering from the subbasis consisting of sets open in
$X$
is covered by an open covering from the subbasis consisting of sets open in
![]() $X$
and sets
$X$
and sets
![]() $X\setminus \mathord{\uparrow } x$
for
$X\setminus \mathord{\uparrow } x$
for
![]() $x\in X$
. Let
$x\in X$
. Let
![]() $S$
be the set of
$S$
be the set of
![]() $x$
such that
$x$
such that
![]() $X\setminus \mathord{\uparrow } x$
is in the cover and
$X\setminus \mathord{\uparrow } x$
is in the cover and
![]() $\mathscr{U}$
the family of sets
$\mathscr{U}$
the family of sets
![]() $U$
in the cover with
$U$
in the cover with
![]() $U\not \in \{X\setminus \mathord{\uparrow } x : x\in S\}$
. If
$U\not \in \{X\setminus \mathord{\uparrow } x : x\in S\}$
. If
![]() $S$
is empty, then
$S$
is empty, then
![]() $\mathscr{U}$
is an open cover of
$\mathscr{U}$
is an open cover of
![]() $X$
. As
$X$
. As
![]() $X$
is compact, there is a finite subcover
$X$
is compact, there is a finite subcover
![]() $\mathscr{U}_0$
. If
$\mathscr{U}_0$
. If
![]() $\mathscr{U}$
is empty, then the family
$\mathscr{U}$
is empty, then the family
![]() $\{X\setminus \mathord{\uparrow } x: x\in S\}$
is a lower-open cover
$\{X\setminus \mathord{\uparrow } x: x\in S\}$
is a lower-open cover
![]() $X$
, whence
$X$
, whence
![]() $\bigcap \{\mathord{\uparrow } x:x\in S\}=\emptyset$
. By Theorem35(4) (with
$\bigcap \{\mathord{\uparrow } x:x\in S\}=\emptyset$
. By Theorem35(4) (with
![]() $U=\emptyset$
) there exists a finite subset
$U=\emptyset$
) there exists a finite subset
![]() $S_0$
of
$S_0$
of
![]() $S$
such that
$S$
such that
![]() $\bigcap \{\mathord{\uparrow } x:x\in S_0\}=\emptyset$
. Then
$\bigcap \{\mathord{\uparrow } x:x\in S_0\}=\emptyset$
. Then
![]() $\{X\setminus \mathord{\uparrow } x: x\in S_0\}$
is a finite subcover of
$\{X\setminus \mathord{\uparrow } x: x\in S_0\}$
is a finite subcover of
![]() $X$
.
$X$
.
In the remaining case, let
![]() $A=\bigcap \{\mathord{\uparrow } x:x\in S\}$
, a nonempty set. Then, the union
$A=\bigcap \{\mathord{\uparrow } x:x\in S\}$
, a nonempty set. Then, the union
![]() $U$
of all the sets in
$U$
of all the sets in
![]() $\mathscr{U}$
is an open set containing
$\mathscr{U}$
is an open set containing
![]() $A$
. Again from Theorem35(4) there exist a finite subset
$A$
. Again from Theorem35(4) there exist a finite subset
![]() $S_0$
of
$S_0$
of
![]() $S$
such that
$S$
such that
![]() $F=\bigcap \{\mathord{\uparrow } x:x\in S_0\}\subseteq U$
. By hypothesis
$F=\bigcap \{\mathord{\uparrow } x:x\in S_0\}\subseteq U$
. By hypothesis
![]() $F$
is compact in
$F$
is compact in
![]() $X$
, so finitely many of the open sets in
$X$
, so finitely many of the open sets in
![]() $\mathscr{U}$
must cover
$\mathscr{U}$
must cover
![]() $F$
. These finitely many open sets combined with
$F$
. These finitely many open sets combined with
![]() $\{X\setminus \mathord{\uparrow } x:x\in S_0\}$
are then a finite subcover of the original cover. By the Alexander Subbasis Theorem
$\{X\setminus \mathord{\uparrow } x:x\in S_0\}$
are then a finite subcover of the original cover. By the Alexander Subbasis Theorem
![]() $X$
is compact in the joint topology
$X$
is compact in the joint topology
![]() $\mathscr{O}(X)\bigvee \omega (X)$
.
$\mathscr{O}(X)\bigvee \omega (X)$
.
Example 41.
Construct a Noetherian poset
![]() $P$
by taking an infinite antichain
$P$
by taking an infinite antichain
![]() $A$
and attaching two incomparable lower bounds
$A$
and attaching two incomparable lower bounds
![]() $y,z$
to
$y,z$
to
![]() $A$
and equipping it with the Scott topology, which is the Alexandroff discrete topology. Clearly,
$A$
and equipping it with the Scott topology, which is the Alexandroff discrete topology. Clearly,
![]() $\Sigma P$
is compact and satisfies Theorem
35
(4) since any subset of
$\Sigma P$
is compact and satisfies Theorem
35
(4) since any subset of
![]() $S$
of cardinality greater than 3 has no upper bound, and hence, is an R-space. However,
$S$
of cardinality greater than 3 has no upper bound, and hence, is an R-space. However,
![]() $\mathord{\uparrow } y\cap \mathord{\uparrow } z$
is not Scott-compact, and the joint topology
$\mathord{\uparrow } y\cap \mathord{\uparrow } z$
is not Scott-compact, and the joint topology
![]() $\sigma (P)\bigvee \omega )P)$
(i.e., the Lawson topology) on
$\sigma (P)\bigvee \omega )P)$
(i.e., the Lawson topology) on
![]() $P$
is discrete and hence noncompact.
$P$
is discrete and hence noncompact.
We can modify the preceding ideas to derive a sufficient condition for a space to be an R-space.
Proposition 42.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space for which the joint topology
$T_0$
-space for which the joint topology
![]() $\mathscr{O}(X)\bigvee \omega (X)$
is compact when restricted to any
$\mathscr{O}(X)\bigvee \omega (X)$
is compact when restricted to any
![]() $\mathord{\uparrow } x$
. Then
$\mathord{\uparrow } x$
. Then
![]() $X$
is an R-space.
$X$
is an R-space.
Proof. Suppose that
![]() $\{\mathord{\uparrow }F_{j}:j\in J\}\subseteq \mathbf{Fin} X$
and
$\{\mathord{\uparrow }F_{j}:j\in J\}\subseteq \mathbf{Fin} X$
and
![]() $U$
is an open subset of
$U$
is an open subset of
![]() $X$
with
$X$
with
![]() $\bigcap _{j\in J}\mathord{\uparrow }F_{j}\subseteq U$
. Select an
$\bigcap _{j\in J}\mathord{\uparrow }F_{j}\subseteq U$
. Select an
![]() $j_0\in J$
. Then
$j_0\in J$
. Then
![]() $\uparrow F_{j_0}\subseteq U\cup \bigcup _{j\in J\setminus \{j_0\}}( x\setminus \mathord{\uparrow }F_{j})$
. As the joint topology
$\uparrow F_{j_0}\subseteq U\cup \bigcup _{j\in J\setminus \{j_0\}}( x\setminus \mathord{\uparrow }F_{j})$
. As the joint topology
![]() $\mathscr{O}(X)\bigvee \omega (X)$
restricted to each
$\mathscr{O}(X)\bigvee \omega (X)$
restricted to each
![]() $\mathord{\uparrow } x$
is compact and
$\mathord{\uparrow } x$
is compact and
![]() $F_{j_0}$
is finite,
$F_{j_0}$
is finite,
![]() $\uparrow F_{j_0}$
is compact in
$\uparrow F_{j_0}$
is compact in
![]() $(X, \mathscr{O}(X)\bigvee \omega (X))$
, whence there exists
$(X, \mathscr{O}(X)\bigvee \omega (X))$
, whence there exists
![]() $J_{0}\in (J\setminus \{j_0\})^{(\lt \omega )}$
such that
$J_{0}\in (J\setminus \{j_0\})^{(\lt \omega )}$
such that
![]() $\uparrow F_{j_0}\subseteq U\cup \bigcup _{j\in J_0}(X\setminus \mathord{\uparrow }F_{j})$
or, equivalently,
$\uparrow F_{j_0}\subseteq U\cup \bigcup _{j\in J_0}(X\setminus \mathord{\uparrow }F_{j})$
or, equivalently,
![]() $\bigcap _{j\in J_{0}\cup \{j_o\}}\mathord{\uparrow }F_{j}\subseteq U$
. Thus,
$\bigcap _{j\in J_{0}\cup \{j_o\}}\mathord{\uparrow }F_{j}\subseteq U$
. Thus,
![]() $X$
is an R-space.
$X$
is an R-space.
We recall a result important for our purposes from Lawson et al. (Reference Lawson, Wu and Xi2020, Theorem 7.1).
Theorem 43.
If the Scott space
![]() $\Sigma P$
for a dcpo
$\Sigma P$
for a dcpo
![]() $P$
is
$P$
is
![]() $\Omega ^*$
-compact (i.e.,
$\Omega ^*$
-compact (i.e.,
![]() $\Sigma P$
is an R-space), then it is well-filtered.
$\Sigma P$
is an R-space), then it is well-filtered.
From the preceding Lemma 25 and Jia et al. (Reference Jia2018, Theorem 3.4), we derive the following equivalences.
Theorem 44.
Let
![]() $L$
be a dcpo equipped with the Scott topology. Then the following conditions are equivalent:
$L$
be a dcpo equipped with the Scott topology. Then the following conditions are equivalent:
-
(1)
 $L$
is a compact,
$L$
is a compact,
 $\Omega ^*$
-compact (i.e.,
$\Omega ^*$
-compact (i.e.,
 $\Sigma L$
is a compact R-space), and
$\Sigma L$
is a compact R-space), and
 $\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for each
$\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for each
 $x,y\in L$
.
$x,y\in L$
. -
(2)
 $L$
is compact, well-filtered, and
$L$
is compact, well-filtered, and
 $\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for each
$\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for each
 $x,y\in L$
.
$x,y\in L$
. -
(3)
 $L$
is finitely generated, well-filtered, and coherent.
$L$
is finitely generated, well-filtered, and coherent.
-
(4)
 $L$
is well-filtered and patch-compact (i.e.,
$L$
is well-filtered and patch-compact (i.e.,
 $L$
is compact in the patch topology
$L$
is compact in the patch topology
 $\sigma (L)^{\sharp }$
).
$\sigma (L)^{\sharp }$
).
-
(5)
 $L$
is well-filtered and Lawson-compact.
$L$
is well-filtered and Lawson-compact.
-
(6)
 $L$
is patch-compact.
$L$
is patch-compact.
-
(7)
 $L$
is Lawson-compact.
$L$
is Lawson-compact.
Proof. Items (2) through (5) are shown to be equivalent in Jia et al. (Reference Jia2018, Theorem 3.4).
(1)
![]() $\Rightarrow$
(2): Follows directly from Theorem43.
$\Rightarrow$
(2): Follows directly from Theorem43.
(5)
![]() $\Rightarrow$
(7): Trivial.
$\Rightarrow$
(7): Trivial.
(6)
![]() $\Rightarrow$
(7): Since the patch topology
$\Rightarrow$
(7): Since the patch topology
![]() $\sigma (L)^{\sharp }$
is finer than the Lawson topology
$\sigma (L)^{\sharp }$
is finer than the Lawson topology
![]() $\lambda (L)$
.
$\lambda (L)$
.
(7)
![]() $\Rightarrow$
(1): By Proposition 40 (for
$\Rightarrow$
(1): By Proposition 40 (for
![]() $X=\Sigma L$
).
$X=\Sigma L$
).
Remark 45.
By Lemma 7, the conditions of
![]() $L$
being compact and being finitely generated are interchangeable in the various conditions of Theorem
44
.
$L$
being compact and being finitely generated are interchangeable in the various conditions of Theorem
44
.
Now we turn to locally hypercompact spaces (see Definition 15), also called locally finitary compact spaces (Goubault-Larrecq Reference Goubault-Larrecq2013, Exercise 5.1.42) or
![]() $qc$
-spaces.
$qc$
-spaces.
Lemma 46.
Let
![]() $(X,\tau )$
be a locally hypercompact space. Then
$(X,\tau )$
be a locally hypercompact space. Then
-
(1) Every compact saturated set is closed in the
 $\omega$
-topology.
$\omega$
-topology.
-
(2) The order of specialization is a closed order in the product space
 $(X, \mathscr{O}(X)\bigvee \omega (X))\times (X, \mathscr{O}(X)\bigvee \omega (X))$
. In particular, the joint topology
$(X, \mathscr{O}(X)\bigvee \omega (X))\times (X, \mathscr{O}(X)\bigvee \omega (X))$
. In particular, the joint topology
 $\mathscr{O}(X)\bigvee \omega (X)$
is Hausdorff.
$\mathscr{O}(X)\bigvee \omega (X)$
is Hausdorff.
Proof. (1): Let
![]() $K$
be a compact saturated set and let
$K$
be a compact saturated set and let
![]() $U$
be an open set containing
$U$
be an open set containing
![]() $K$
. By local hypercompactness, we can choose for each
$K$
. By local hypercompactness, we can choose for each
![]() $x\in K$
a finite set
$x\in K$
a finite set
![]() $F_x$
such that
$F_x$
such that
![]() $x\in \mathrm{int}\mathord{\uparrow } F_x\subseteq \mathord{\uparrow } F_x\subseteq U$
. By the compactness of
$x\in \mathrm{int}\mathord{\uparrow } F_x\subseteq \mathord{\uparrow } F_x\subseteq U$
. By the compactness of
![]() $K$
, there is a finite set
$K$
, there is a finite set
![]() $\{x_1, x_2, \ldots, x_n\}$
of
$\{x_1, x_2, \ldots, x_n\}$
of
![]() $K$
such that
$K$
such that
![]() $K\subseteq \bigcup _{i=1}^n \mathrm{int}\mathord{\uparrow } F_{x_i}$
. Let
$K\subseteq \bigcup _{i=1}^n \mathrm{int}\mathord{\uparrow } F_{x_i}$
. Let
![]() $F=\bigcup _{i=1}^n \mathord{\uparrow } F_{x_i}$
. Then
$F=\bigcup _{i=1}^n \mathord{\uparrow } F_{x_i}$
. Then
![]() $\mathord{\uparrow } F$
is an
$\mathord{\uparrow } F$
is an
![]() $\omega$
-closed set containing
$\omega$
-closed set containing
![]() $K$
and contained in
$K$
and contained in
![]() $U$
. By saturation of
$U$
. By saturation of
![]() $K$
, it is the intersection of all such
$K$
, it is the intersection of all such
![]() $U$
, thus the intersection of the finitely generated upper sets just constructed for each
$U$
, thus the intersection of the finitely generated upper sets just constructed for each
![]() $U$
.
$U$
.
(2): Assume that
![]() $x\not \leq y$
. Then there exists a finite set
$x\not \leq y$
. Then there exists a finite set
![]() $F\subseteq X\setminus \mathord{\downarrow } y$
and a
$F\subseteq X\setminus \mathord{\downarrow } y$
and a
![]() $\tau$
-open set
$\tau$
-open set
![]() $V$
such that
$V$
such that
![]() $x\in V\subseteq \mathord{\uparrow } F$
. Then
$x\in V\subseteq \mathord{\uparrow } F$
. Then
![]() $V\times (X\setminus \mathord{\uparrow } F)$
is an open set in the product space
$V\times (X\setminus \mathord{\uparrow } F)$
is an open set in the product space
![]() $(X, \mathscr{O}(X)\bigvee \omega (X))\times (X, \mathscr{O}(X)\bigvee \omega (X))$
that misses the graph
$(X, \mathscr{O}(X)\bigvee \omega (X))\times (X, \mathscr{O}(X)\bigvee \omega (X))$
that misses the graph
![]() $\{(u,v)\in X\times X: u\leq v\}$
of the order of specialization. We conclude that
$\{(u,v)\in X\times X: u\leq v\}$
of the order of specialization. We conclude that
![]() $\leq$
and
$\leq$
and
![]() $\geq$
are closed in the product space
$\geq$
are closed in the product space
![]() $(X, \mathscr{O}(X)\bigvee \omega (X))\times (X, \mathscr{O}(X)\bigvee \omega (X))$
. Then the diagonal
$(X, \mathscr{O}(X)\bigvee \omega (X))\times (X, \mathscr{O}(X)\bigvee \omega (X))$
. Then the diagonal
![]() $\leq \cap \geq$
is also closed, so
$\leq \cap \geq$
is also closed, so
![]() $X$
with the joint topology
$X$
with the joint topology
![]() $\mathscr{O}(X)\bigvee \omega (X)$
is Hausdorff.
$\mathscr{O}(X)\bigvee \omega (X)$
is Hausdorff.
We refer the reader to Lawson (Reference Lawson1998) for results related to the previous lemma and following proposition.
Proposition 47.
Let
![]() $P$
be a quasicontinuous domain for which the Scott space
$P$
be a quasicontinuous domain for which the Scott space
![]() $(P,\sigma (P))$
is an R-space. Then
$(P,\sigma (P))$
is an R-space. Then
![]() $(P,\sigma (P))$
and
$(P,\sigma (P))$
and
![]() $(P,\omega (P))$
are de Groot duals of each other.
$(P,\omega (P))$
are de Groot duals of each other.
Proof. By Lemma 16
![]() $\Sigma X$
is a locally hypercompact space. So by Lemma 46(1) the de Groot dual topology of
$\Sigma X$
is a locally hypercompact space. So by Lemma 46(1) the de Groot dual topology of
![]() $\sigma (P)$
is contained in the lower topology. Since each
$\sigma (P)$
is contained in the lower topology. Since each
![]() $\mathord{\uparrow } x$
is compact in the Scott topology, it is closed in the de Groot dual topology, and hence the lower topology is contained in the de Groot dual topology since the sets
$\mathord{\uparrow } x$
is compact in the Scott topology, it is closed in the de Groot dual topology, and hence the lower topology is contained in the de Groot dual topology since the sets
![]() $\mathord{\uparrow } x$
form a subbasis for the closed sets.
$\mathord{\uparrow } x$
form a subbasis for the closed sets.
Since the Scott space
![]() $(P,\sigma (P))$
is an R-space, by Corollary 36 the de Groot dual of the lower topology of
$(P,\sigma (P))$
is an R-space, by Corollary 36 the de Groot dual of the lower topology of
![]() $P$
is the Scott topology of
$P$
is the Scott topology of
![]() $P$
.
$P$
.
Example 48.
Let
![]() $P$
be the negative integers (equipped with the usual order of integers) with two incomparable lower bounds
$P$
be the negative integers (equipped with the usual order of integers) with two incomparable lower bounds
![]() $\bot _0$
and
$\bot _0$
and
![]() $\bot _1$
adjoined. Then
$\bot _1$
adjoined. Then
![]() $P$
is Noetherian, hence an algebraic domain with all elements compact, in particular a quasicontinuous domain. All order consistent and dual order consistent topologies collapse to all upper sets and all lower sets respectively, more precisely,
$P$
is Noetherian, hence an algebraic domain with all elements compact, in particular a quasicontinuous domain. All order consistent and dual order consistent topologies collapse to all upper sets and all lower sets respectively, more precisely,
![]() $\upsilon (P)=\alpha (P)$
and
$\upsilon (P)=\alpha (P)$
and
![]() $\omega (P)=\alpha (P^{op})$
. Clearly, both the (upper) Alexandroff topology
$\omega (P)=\alpha (P^{op})$
. Clearly, both the (upper) Alexandroff topology
![]() $\alpha (P)$
and the (lower) Alexandroff topology
$\alpha (P)$
and the (lower) Alexandroff topology
![]() $\alpha (P^{op})$
are compact. So both
$\alpha (P^{op})$
are compact. So both
![]() $(P, \omega (P))$
and
$(P, \omega (P))$
and
![]() $\Sigma P$
are compact R-spaces, whence the conditions of Proposition 47 are satisfied, and hence
$\Sigma P$
are compact R-spaces, whence the conditions of Proposition 47 are satisfied, and hence
![]() $\sigma (P)^{\sharp }=\omega (P)^{\sharp }=\sigma (P)\bigvee \omega (P)=\lambda (P)$
. Since
$\sigma (P)^{\sharp }=\omega (P)^{\sharp }=\sigma (P)\bigvee \omega (P)=\lambda (P)$
. Since
![]() $P$
is Noetherian and the dual poset
$P$
is Noetherian and the dual poset
![]() $P^{op}$
of
$P^{op}$
of
![]() $P$
is not a dcpo,
$P$
is not a dcpo,
![]() $\Sigma P$
is sober and
$\Sigma P$
is sober and
![]() $(P, \omega (P))$
is not a
$(P, \omega (P))$
is not a
![]() $d$
-space (and hence not well-filtered). Clearly,
$d$
-space (and hence not well-filtered). Clearly,
![]() $\mathsf{K}((P, \omega (P))=\{\mathord{\downarrow } A : \emptyset \neq A\subseteq P\}$
. So
$\mathsf{K}((P, \omega (P))=\{\mathord{\downarrow } A : \emptyset \neq A\subseteq P\}$
. So
![]() $((P, \omega (P))$
is coherent, but
$((P, \omega (P))$
is coherent, but
![]() $((P, \sigma (P))$
is not coherent since
$((P, \sigma (P))$
is not coherent since
![]() $\mathord{\uparrow } \bot _0\cap \mathord{\uparrow } \bot _1$
is not Scott-compact. The Lawson topology
$\mathord{\uparrow } \bot _0\cap \mathord{\uparrow } \bot _1$
is not Scott-compact. The Lawson topology
![]() $\lambda (P)$
is discrete and hence noncompact.
$\lambda (P)$
is discrete and hence noncompact.
4. Strong
 $\textit{d}$
-Spaces
$\textit{d}$
-Spaces
As a strengthened version of
![]() $d$
-spaces, the notion of strong
$d$
-spaces, the notion of strong
![]() $d$
-spaces was introduced in Xu and Zhao (Reference Xu and Zhao2020). In this section, we will give some characterizations of strong
$d$
-spaces was introduced in Xu and Zhao (Reference Xu and Zhao2020). In this section, we will give some characterizations of strong
![]() $d$
-spaces. These characterizations indicate that the notion of a strong
$d$
-spaces. These characterizations indicate that the notion of a strong
![]() $d$
-space is, in some sense, a variant of join continuity. We also find conditions on a
$d$
-space is, in some sense, a variant of join continuity. We also find conditions on a
![]() $d$
-space
$d$
-space
![]() $X$
under which
$X$
under which
![]() $X$
is sober. In particular, we show that for a dcpo
$X$
is sober. In particular, we show that for a dcpo
![]() $P$
, if
$P$
, if
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space and
$d$
-space and
![]() $\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then
$\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
Definition 49.
(Xu and Zhao Reference Xu and Zhao2020, Definition 3.18) A
![]() $T_0$
-space
$T_0$
-space
![]() $X$
is called a strong
$X$
is called a strong
![]() $d$
-space if for any
$d$
-space if for any
![]() $D\in \mathscr{D}(X)$
,
$D\in \mathscr{D}(X)$
,
![]() $x\in X$
and
$x\in X$
and
![]() $U\in \mathscr{O}(X)$
,
$U\in \mathscr{O}(X)$
,
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
implies
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
implies
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
for some
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
for some
![]() $d\in D$
. The category of all strong
$d\in D$
. The category of all strong
![]() $d$
-spaces and continuous mappings is denoted by
$d$
-spaces and continuous mappings is denoted by
![]() $\mathbf{S}$
-
$\mathbf{S}$
-
![]() $\mathbf{Top}_d$
.
$\mathbf{Top}_d$
.
We list some elementary properties of strong
![]() $d$
-spaces.
$d$
-spaces.
Lemma 50.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space.
$T_0$
-space.
-
(1) If
 $X$
is a strong
$X$
is a strong
 $d$
-space, then it is a
$d$
-space, then it is a
 $d$
-space.
$d$
-space.
-
(2) If
 $X$
is a strong
$X$
is a strong
 $d$
-space,
$d$
-space,
 $D$
is directed,
$D$
is directed,
 $A$
is closed, and
$A$
is closed, and
 $\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
for all
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
for all
 $d\in D$
, then
$d\in D$
, then
 $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
.
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
. -
(3) If
 $X$
is an R-space, then
$X$
is an R-space, then
 $X$
is a strong
$X$
is a strong
 $d$
-space.
$d$
-space.
Proof. (1): Suppose that
![]() $D\in \mathscr{D}(X)$
and
$D\in \mathscr{D}(X)$
and
![]() $U\in \mathscr{O}(X)$
with
$U\in \mathscr{O}(X)$
with
![]() $\bigcap \limits \limits _{d\in D}\mathord{\uparrow } d\subseteq U$
. Select any
$\bigcap \limits \limits _{d\in D}\mathord{\uparrow } d\subseteq U$
. Select any
![]() $x\in D$
. Then
$x\in D$
. Then
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x=\bigcap _{d\in D}\mathord{\uparrow } d\subseteq U$
. As
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x=\bigcap _{d\in D}\mathord{\uparrow } d\subseteq U$
. As
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space, there exists
$d$
-space, there exists
![]() $d_0\in \overline{D}$
such that
$d_0\in \overline{D}$
such that
![]() $\mathord{\uparrow } d_0\cap \mathord{\uparrow } x\subseteq U$
. By the directedness of
$\mathord{\uparrow } d_0\cap \mathord{\uparrow } x\subseteq U$
. By the directedness of
![]() $D$
, there is
$D$
, there is
![]() $d\in D$
with
$d\in D$
with
![]() $d_0\leq d$
and
$d_0\leq d$
and
![]() $x\leq d$
. Then
$x\leq d$
. Then
![]() $\mathord{\uparrow } d\subseteq \mathord{\uparrow } d_0\cap \mathord{\uparrow } x\subseteq U$
. By Proposition 20
$\mathord{\uparrow } d\subseteq \mathord{\uparrow } d_0\cap \mathord{\uparrow } x\subseteq U$
. By Proposition 20
![]() $X$
is a
$X$
is a
![]() $d$
-space.
$d$
-space.
(2): Follows immediately from the definition by taking
![]() $U=X\setminus A$
.
$U=X\setminus A$
.
(3): Suppose
![]() $\bigcap \{\mathord{\uparrow } x\cap \mathord{\uparrow } d: d\in D\}\subseteq U$
, where
$\bigcap \{\mathord{\uparrow } x\cap \mathord{\uparrow } d: d\in D\}\subseteq U$
, where
![]() $D$
is a directed set and
$D$
is a directed set and
![]() $U$
is open. Setting
$U$
is open. Setting
![]() $S=D\cup \{x\}$
and applying Theorem35(4), we conclude there exists a finite subset
$S=D\cup \{x\}$
and applying Theorem35(4), we conclude there exists a finite subset
![]() $S_0$
such that
$S_0$
such that
![]() $\bigcap \{\mathord{\uparrow } q : q\in S_0\}\subseteq U$
. Then
$\bigcap \{\mathord{\uparrow } q : q\in S_0\}\subseteq U$
. Then
![]() $\bigcap _{d\in S_0\setminus \{x\}}\mathord{\uparrow } d\cap \mathord{\uparrow } x \subseteq U$
. Hence,
$\bigcap _{d\in S_0\setminus \{x\}}\mathord{\uparrow } d\cap \mathord{\uparrow } x \subseteq U$
. Hence,
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space.
$d$
-space.
Fig. 1 shows certain relations of some spaces lying between
![]() $d$
-spaces and
$d$
-spaces and
![]() $T_2$
-spaces (all implications in Fig. 1 are irreversible).
$T_2$
-spaces (all implications in Fig. 1 are irreversible).

Figure 1. Relations of some spaces lying between
![]() $d$
-spaces and
$d$
-spaces and
![]() $T_2$
spaces.
$T_2$
spaces.
In Li et al. (Reference Li, Jin, Miao and Chen2023, Example 5.2), a poset
![]() $P$
was given to show that
$P$
was given to show that
![]() $P$
equipped with a certain topology
$P$
equipped with a certain topology
![]() $\tau$
is a strong
$\tau$
is a strong
![]() $d$
-space but the product space
$d$
-space but the product space
![]() $(P, \tau )\times (P, \tau )$
is not a strong
$(P, \tau )\times (P, \tau )$
is not a strong
![]() $d$
-space (and hence the category
$d$
-space (and hence the category
![]() $\mathbf{S}$
-
$\mathbf{S}$
-
![]() $\mathbf{Top}_d$
is not a reflective subcategory of
$\mathbf{Top}_d$
is not a reflective subcategory of
![]() $\mathbf{Top}_0$
). Using this space we will show that the product of two R-spaces is not an R-space in general.
$\mathbf{Top}_0$
). Using this space we will show that the product of two R-spaces is not an R-space in general.
Example 51.
Let
![]() $P=\mathbb{N}\cup \{\omega \}\cup \{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}\cup \{a_1, a_2, \ldots, a_n, \ldots \}\cup \{a\}$
. Define an order on
$P=\mathbb{N}\cup \{\omega \}\cup \{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}\cup \{a_1, a_2, \ldots, a_n, \ldots \}\cup \{a\}$
. Define an order on
![]() $P$
as follows (see Fig.
2
):
$P$
as follows (see Fig.
2
):
-
(i)
 $1\lt 2\lt 3\lt \ldots \lt n\lt n+1\lt \omega \textrm{ for all }n\in \mathbf{N}$
;
$1\lt 2\lt 3\lt \ldots \lt n\lt n+1\lt \omega \textrm{ for all }n\in \mathbf{N}$
; -
(ii)
 $\omega \lt \beta _n \textrm{ for all }n\in \mathbf{N}$
;
$\omega \lt \beta _n \textrm{ for all }n\in \mathbf{N}$
; -
(iii)
 $n\lt a_m \textrm{ iff }n\leq m$
;
$n\lt a_m \textrm{ iff }n\leq m$
; -
(iv)
 $a\lt \beta _n \textrm{ and } a\lt a_n \textrm{ for all }n\in \mathbf{N}$
.
$a\lt \beta _n \textrm{ and } a\lt a_n \textrm{ for all }n\in \mathbf{N}$
.

Figure 2. The poset
![]() $P$
in Example 51.
$P$
in Example 51.
Let
![]() $B=\{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}$
and
$B=\{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}$
and
![]() $A=\{a_1, a_2, \ldots, a_n, \ldots \}$
, and let
$A=\{a_1, a_2, \ldots, a_n, \ldots \}$
, and let
![]() $\tau _1=\{U\in \sigma (P) : A\setminus U \textrm{ is finite} \}$
and
$\tau _1=\{U\in \sigma (P) : A\setminus U \textrm{ is finite} \}$
and
![]() $\tau _2=\{U\in \sigma (P) : U\subseteq A\}$
. Define
$\tau _2=\{U\in \sigma (P) : U\subseteq A\}$
. Define
![]() $\tau =\tau _1\bigcup \tau _2$
. Then
$\tau =\tau _1\bigcup \tau _2$
. Then
-
(a)
 $\textrm{max}(P)=A\cup B$
.
$\textrm{max}(P)=A\cup B$
. -
(b)
 $D\in \mathscr{D}(P)$
iff
$D\in \mathscr{D}(P)$
iff
 $D\subseteq \mathbb{N}$
is an infinite chain or
$D\subseteq \mathbb{N}$
is an infinite chain or
 $D$
has a largest element. So
$D$
has a largest element. So
 $P$
is a dcpo. If
$P$
is a dcpo. If
 $D\subseteq \mathbb{N}$
is an infinite chain or
$D\subseteq \mathbb{N}$
is an infinite chain or
 $D$
has a largest element, then
$D$
has a largest element, then
 $D\in \mathscr{D}(P)$
. Conversely, suppose that
$D\in \mathscr{D}(P)$
. Conversely, suppose that
 $D$
is a directed subset of
$D$
is a directed subset of
 $P$
and
$P$
and
 $D$
has no largest element. Then
$D$
has no largest element. Then
 $D$
is countably infinite and
$D$
is countably infinite and
 $|D\cap \textrm{max}(P)|\lt 2$
. If there exists
$|D\cap \textrm{max}(P)|\lt 2$
. If there exists
 $d^{\ast }\in D$
with
$d^{\ast }\in D$
with
 $d^{\ast }\in \textrm{max}(P)$
, then for any
$d^{\ast }\in \textrm{max}(P)$
, then for any
 $d\in D$
, by the directedness of
$d\in D$
, by the directedness of
 $D$
, there is
$D$
, there is
 $d^{\prime }\in D$
such that
$d^{\prime }\in D$
such that
 $d\leq d^{\prime }$
and
$d\leq d^{\prime }$
and
 $d^{\ast }\leq d^{\prime }$
. Then
$d^{\ast }\leq d^{\prime }$
. Then
 $d\leq d^{\prime }=d^{\ast }$
. So
$d\leq d^{\prime }=d^{\ast }$
. So
 $d^{\ast }$
is the largest element of
$d^{\ast }$
is the largest element of
 $D$
, a contradiction. Hence,
$D$
, a contradiction. Hence,
 $D\cap \textrm{max}(P)=\emptyset$
, that is,
$D\cap \textrm{max}(P)=\emptyset$
, that is,
 $D\subseteq \mathord{\downarrow } \omega \cup \{a\}$
. Since for any
$D\subseteq \mathord{\downarrow } \omega \cup \{a\}$
. Since for any
 $s\in \mathord{\downarrow } \omega$
,
$s\in \mathord{\downarrow } \omega$
,
 $s$
and
$s$
and
 $a$
have no upper bound in
$a$
have no upper bound in
 $D$
, we have
$D$
, we have
 $a\not \in D$
, and hence,
$a\not \in D$
, and hence,
 $D\subseteq \mathord{\downarrow } \omega$
. As
$D\subseteq \mathord{\downarrow } \omega$
. As
 $D$
has no largest element,
$D$
has no largest element,
 $D\subseteq \mathbb{N}$
is an infinite chain.
$D\subseteq \mathbb{N}$
is an infinite chain.
-
(c)
 $P$
is an algebraic domain. By (b),
$P$
is an algebraic domain. By (b),
 $x\ll x$
for all
$x\ll x$
for all
 $x\in P\setminus \{\omega \}$
. Hence,
$x\in P\setminus \{\omega \}$
. Hence,
 $P$
is an algebraic domain.
$P$
is an algebraic domain.
-
(d)
 $\Sigma P$
is sober, not a strong
$\Sigma P$
is sober, not a strong
 $d$
-space and not coherent. By (c) and Proposition 22,
$d$
-space and not coherent. By (c) and Proposition 22,
 $\Sigma P$
is sober. We have that
$\Sigma P$
is sober. We have that
 $\bigcap _{n\in \mathbb{N}}\mathord{\uparrow } n\cap \mathord{\uparrow } a=\{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}\in \sigma (P)$
, but
$\bigcap _{n\in \mathbb{N}}\mathord{\uparrow } n\cap \mathord{\uparrow } a=\{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}\in \sigma (P)$
, but
 $\mathord{\uparrow } m\cap \mathord{\uparrow } a=\{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}\cup \{a_m, a_{m+1}, \ldots \}\not \subseteq \{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}$
for any
$\mathord{\uparrow } m\cap \mathord{\uparrow } a=\{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}\cup \{a_m, a_{m+1}, \ldots \}\not \subseteq \{\beta _1, \beta _2, \ldots, \beta _n, \ldots \}$
for any
 $m\in \mathbb{N}$
. Hence,
$m\in \mathbb{N}$
. Hence,
 $\Sigma P$
is not a strong
$\Sigma P$
is not a strong
 $d$
-space. Clearly,
$d$
-space. Clearly,
 $\mathord{\uparrow } 1, \mathord{\uparrow } a\in \mathord{\mathsf{K}} (\Sigma P)$
, but
$\mathord{\uparrow } 1, \mathord{\uparrow } a\in \mathord{\mathsf{K}} (\Sigma P)$
, but
 $\mathord{\uparrow } 1\cap \mathord{\uparrow } a=A\cup B$
is not Scott-compact (note that
$\mathord{\uparrow } 1\cap \mathord{\uparrow } a=A\cup B$
is not Scott-compact (note that
 $\{\beta _n\}, \{a_n\}\in \sigma (P)$
for any
$\{\beta _n\}, \{a_n\}\in \sigma (P)$
for any
 $n\in \mathbb{N}$
). So
$n\in \mathbb{N}$
). So
 $\Sigma P$
is not coherent.
$\Sigma P$
is not coherent.
-
(e)
 $\tau$
is a topology on
$\tau$
is a topology on
 $P$
and
$P$
and
 $\upsilon (P)\subseteq \tau \subseteq \sigma (P)$
. Hence,
$\upsilon (P)\subseteq \tau \subseteq \sigma (P)$
. Hence,
 $\tau$
is an order-compatible topology of
$\tau$
is an order-compatible topology of
 $P$
and
$P$
and
 $(P, \tau ))$
is a
$(P, \tau ))$
is a
 $d$
-space. It was showed in (Li et al., 2023, Example 5.2).
$d$
-space. It was showed in (Li et al., 2023, Example 5.2).
-
(f)
 $(P, \tau )$
is sober and not coherent. Suppose that
$(P, \tau )$
is sober and not coherent. Suppose that
 $C\in{\mathsf{Irr}}_c((P, \tau ))$
. Then by (e) and Lemma 19,
$C\in{\mathsf{Irr}}_c((P, \tau ))$
. Then by (e) and Lemma 19,
 $C=\mathord{\downarrow } \textrm{max}(P)$
. We claim that
$C=\mathord{\downarrow } \textrm{max}(P)$
. We claim that
 $\textrm{max}(C)$
is finite. Assume, on the contrary, that
$\textrm{max}(C)$
is finite. Assume, on the contrary, that
 $\textrm{max}(C)$
is (countably) infinite. Since
$\textrm{max}(C)$
is (countably) infinite. Since
 $C$
is Scott-closed and
$C$
is Scott-closed and
 $\omega =\bigvee \mathbb{N}$
,
$\omega =\bigvee \mathbb{N}$
,
 $\textrm{max}(C)\cap (\mathord{\downarrow } \omega \cup \{a\})$
is a finite set, and consequently,
$\textrm{max}(C)\cap (\mathord{\downarrow } \omega \cup \{a\})$
is a finite set, and consequently,
 $\textrm{max}(C)\cap \textrm{max}(P)$
is infinite.
$\textrm{max}(C)\cap \textrm{max}(P)$
is infinite.
Case 1:
 $|\textrm{max}(C)\cap A|\geq 2$
. Select any
$|\textrm{max}(C)\cap A|\geq 2$
. Select any
 $a_l, a_k\in \textrm{max}(C)\cap A$
with
$a_l, a_k\in \textrm{max}(C)\cap A$
with
 $a_l\neq a_k$
. Let
$a_l\neq a_k$
. Let
 $U_1=\{a_l\}$
and
$U_1=\{a_l\}$
and
 $U_2=\{a_k\}$
. Then
$U_2=\{a_k\}$
. Then
 $U_1, U_2\in \tau _2\subseteq \tau$
,
$U_1, U_2\in \tau _2\subseteq \tau$
,
 $a_l\in C\cap U_1$
and
$a_l\in C\cap U_1$
and
 $a_k\in C\cap U_2$
. But
$a_k\in C\cap U_2$
. But
 $A\cap U_1\cap U_2=\emptyset$
, which is a contradiction with
$A\cap U_1\cap U_2=\emptyset$
, which is a contradiction with
 $C\in{\mathsf{Irr}}_c((P, \tau ))$
.
$C\in{\mathsf{Irr}}_c((P, \tau ))$
.Case 2:
 $|\textrm{max}(C)\cap A|\lt 2$
. As
$|\textrm{max}(C)\cap A|\lt 2$
. As
 $\textrm{max}(C)\cap \textrm{max}(P)=(\textrm{max}(C)\cap A)\cup (\textrm{max}(C)\cap B)$
is infinite,
$\textrm{max}(C)\cap \textrm{max}(P)=(\textrm{max}(C)\cap A)\cup (\textrm{max}(C)\cap B)$
is infinite,
 $\textrm{max}(C)\cap B$
must be infinite. Select any
$\textrm{max}(C)\cap B$
must be infinite. Select any
 $\beta _n, \beta _m\in \textrm{max}(C)\cap B$
with
$\beta _n, \beta _m\in \textrm{max}(C)\cap B$
with
 $\beta _n\neq \beta _m$
. Let
$\beta _n\neq \beta _m$
. Let
 $V_1=\{\beta _n\}\cup (A\setminus \textrm{max}(C)\cap A)$
and
$V_1=\{\beta _n\}\cup (A\setminus \textrm{max}(C)\cap A)$
and
 $V_2=\{\beta _m\}\cup (A\setminus \textrm{max}(C)\cap A)$
. Then
$V_2=\{\beta _m\}\cup (A\setminus \textrm{max}(C)\cap A)$
. Then
 $V_1, V_2\in \tau _1\subseteq \tau$
,
$V_1, V_2\in \tau _1\subseteq \tau$
,
 $\beta _n\in C\cap V_1$
and
$\beta _n\in C\cap V_1$
and
 $\beta _m\in C\cap V_2$
. But
$\beta _m\in C\cap V_2$
. But
 $A\cap V_1\cap V_2=\emptyset$
, which is in contradiction with
$A\cap V_1\cap V_2=\emptyset$
, which is in contradiction with
 $C\in{\mathsf{Irr}}_c((P, \tau ))$
. Hence,
$C\in{\mathsf{Irr}}_c((P, \tau ))$
. Hence,
 $\textrm{max}(C)=\{x_1, x_2, x_3, \ldots, x_n\}$
is finite, then
$\textrm{max}(C)=\{x_1, x_2, x_3, \ldots, x_n\}$
is finite, then
 $C=\mathord{\downarrow } \textrm{max}(C)=\bigcup \limits _{i=1}^n\mathord{\downarrow } x_i$
and hence
$C=\mathord{\downarrow } \textrm{max}(C)=\bigcup \limits _{i=1}^n\mathord{\downarrow } x_i$
and hence
 $C=\mathord{\downarrow } x_m={\textrm{cl}}_{\tau }\{x_m\}$
for some
$C=\mathord{\downarrow } x_m={\textrm{cl}}_{\tau }\{x_m\}$
for some
 $1\leq m\leq n$
by
$1\leq m\leq n$
by
 $C\in{\mathsf{Irr}}_c((P, \tau ))$
. Thus,
$C\in{\mathsf{Irr}}_c((P, \tau ))$
. Thus,
 $(P, \tau ))$
is sober. Clearly,
$(P, \tau ))$
is sober. Clearly,
 $\mathord{\uparrow } 1, \mathord{\uparrow } a\in \mathord{\mathsf{K}} (\Sigma P)$
, but
$\mathord{\uparrow } 1, \mathord{\uparrow } a\in \mathord{\mathsf{K}} (\Sigma P)$
, but
 $\mathord{\uparrow } 1\cap \mathord{\uparrow } a=A\cup B$
is not compact (note that
$\mathord{\uparrow } 1\cap \mathord{\uparrow } a=A\cup B$
is not compact (note that
 $\{\{\beta _n\}\cup A : n\in \mathbb{N}\}$
is a
$\{\{\beta _n\}\cup A : n\in \mathbb{N}\}$
is a
 $\tau$
-open cover of
$\tau$
-open cover of
 $A\cup B$
containing no finite subcover). Therefore,
$A\cup B$
containing no finite subcover). Therefore,
 $(P, \tau ))$
is not coherent.
$(P, \tau ))$
is not coherent.
-
(g)
 $(P, \tau ))$
is an R-space and hence a strong
$(P, \tau ))$
is an R-space and hence a strong
 $d$
-space. Suppose that
$d$
-space. Suppose that
 $S$
is a subset of
$S$
is a subset of
 $P$
and
$P$
and
 $U\in \tau$
with
$U\in \tau$
with
 $\bigcap _{s\in S}\mathord{\uparrow } s\subseteq U$
. We will show that there is a finite subset
$\bigcap _{s\in S}\mathord{\uparrow } s\subseteq U$
. We will show that there is a finite subset
 $S_0$
of
$S_0$
of
 $S$
such that
$S$
such that
 $\bigcap _{s\in S_0}\mathord{\uparrow } s\subseteq U$
. If
$\bigcap _{s\in S_0}\mathord{\uparrow } s\subseteq U$
. If
 $S$
itself is finite, then we let
$S$
itself is finite, then we let
 $S_0=S$
. Now we assume that
$S_0=S$
. Now we assume that
 $S$
is (countably) infinite.
$S$
is (countably) infinite.
Case 1:
 $|S\cap \textrm{max}(P)|\geq 2$
. Select any
$|S\cap \textrm{max}(P)|\geq 2$
. Select any
 $s_1, s_2\in S\cap \textrm{max}(P)$
with
$s_1, s_2\in S\cap \textrm{max}(P)$
with
 $s_l\neq s_2$
. Then
$s_l\neq s_2$
. Then
 $\mathord{\uparrow } s_1\cap \mathord{\uparrow } s_2=\emptyset \subseteq U$
.
$\mathord{\uparrow } s_1\cap \mathord{\uparrow } s_2=\emptyset \subseteq U$
.Case 2:
 $|S\cap A|=1$
and
$|S\cap A|=1$
and
 $S\cap B=\emptyset$
. In this case,
$S\cap B=\emptyset$
. In this case,
 $S\cap \textrm{max}(P)=\{a_m\}$
for some
$S\cap \textrm{max}(P)=\{a_m\}$
for some
 $m\in \mathbb{N}$
and
$m\in \mathbb{N}$
and
 $S\cap \mathbb{N}$
is infinite. Select any
$S\cap \mathbb{N}$
is infinite. Select any
 $k\in S\cap \mathbb{N}$
with
$k\in S\cap \mathbb{N}$
with
 $m\lt k$
. Then
$m\lt k$
. Then
 $\mathord{\uparrow } k\cap \mathord{\uparrow } a_m=\emptyset \subseteq U$
.
$\mathord{\uparrow } k\cap \mathord{\uparrow } a_m=\emptyset \subseteq U$
.Case 3:
 $|S\cap B|=1$
and
$|S\cap B|=1$
and
 $S\cap A=\emptyset$
. Then
$S\cap A=\emptyset$
. Then
 $S\cap \textrm{max}(P)=\{\beta _l\}$
for some
$S\cap \textrm{max}(P)=\{\beta _l\}$
for some
 $l\in \mathbb{N}$
and
$l\in \mathbb{N}$
and
 $S\subseteq \mathord{\downarrow } \omega \cup \{\beta _l\}\cup \{a\}$
. Select any
$S\subseteq \mathord{\downarrow } \omega \cup \{\beta _l\}\cup \{a\}$
. Select any
 $s\in S\cap \mathord{\downarrow } \omega$
, Then
$s\in S\cap \mathord{\downarrow } \omega$
, Then
 $\mathord{\uparrow } s\cap \mathord{\uparrow } \beta _l=\bigcap _{s\in S\cap \mathord{\downarrow } \omega }\mathord{\uparrow } s\cap \mathord{\uparrow } \beta _l\cap \mathord{\uparrow } a=\bigcap _{s\in S}\mathord{\uparrow } s=\{\beta _l\}\subseteq U$
.
$\mathord{\uparrow } s\cap \mathord{\uparrow } \beta _l=\bigcap _{s\in S\cap \mathord{\downarrow } \omega }\mathord{\uparrow } s\cap \mathord{\uparrow } \beta _l\cap \mathord{\uparrow } a=\bigcap _{s\in S}\mathord{\uparrow } s=\{\beta _l\}\subseteq U$
.Case 4:
 $S\cap \textrm{max}(P)=\emptyset$
. We have that
$S\cap \textrm{max}(P)=\emptyset$
. We have that
 $S\subseteq \mathord{\downarrow } \omega \cup \{a\}$
. If
$S\subseteq \mathord{\downarrow } \omega \cup \{a\}$
. If
 $a\in S$
, then
$a\in S$
, then
 $\bigcap _{s\in S}\mathord{\uparrow } s=B\subseteq U$
. So
$\bigcap _{s\in S}\mathord{\uparrow } s=B\subseteq U$
. So
 $A\setminus U$
is finite, and consequently, there is
$A\setminus U$
is finite, and consequently, there is
 $k\in \mathbb{N}$
such that
$k\in \mathbb{N}$
such that
 $\{a_k, a_{k+1}, \ldots \}\subseteq U$
. Select any
$\{a_k, a_{k+1}, \ldots \}\subseteq U$
. Select any
 $m\in S\cap \mathbb{N}$
with
$m\in S\cap \mathbb{N}$
with
 $m\gt k$
(note that
$m\gt k$
(note that
 $S\cap \mathbb{N}$
is infinite). Then
$S\cap \mathbb{N}$
is infinite). Then
 $\mathord{\uparrow } m\cap \mathord{\uparrow } a=B\cup \{a_m, a_{m+1}, \ldots \}\subseteq B\cup \{a_k, a_{k+1}, \ldots \}\subseteq U$
. If
$\mathord{\uparrow } m\cap \mathord{\uparrow } a=B\cup \{a_m, a_{m+1}, \ldots \}\subseteq B\cup \{a_k, a_{k+1}, \ldots \}\subseteq U$
. If
 $a\not \in S$
, then
$a\not \in S$
, then
 $\bigcap _{s\in S}\mathord{\uparrow } s=\mathord{\uparrow } \omega =B\cup \{\omega \}\subseteq U$
. By
$\bigcap _{s\in S}\mathord{\uparrow } s=\mathord{\uparrow } \omega =B\cup \{\omega \}\subseteq U$
. By
 $\omega =\bigvee (S\cap \mathbb{N})$
and
$\omega =\bigvee (S\cap \mathbb{N})$
and
 $U\in \tau \subseteq \sigma (P)$
, there is
$U\in \tau \subseteq \sigma (P)$
, there is
 $n\in S\cap \mathbb{N}$
with
$n\in S\cap \mathbb{N}$
with
 $\mathord{\uparrow } n\subseteq U$
. Thus, by Theorem
35
$\mathord{\uparrow } n\subseteq U$
. Thus, by Theorem
35
 $(P, \tau ))$
is an R-space.
$(P, \tau ))$
is an R-space.
-
(h) The product space
 $(P, \tau ))\times (P, \tau ))$
is not an R-space. It was proved in Li et al. (Reference Li, Jin, Miao and Chen2023, Example 5.2) that
$(P, \tau ))\times (P, \tau ))$
is not an R-space. It was proved in Li et al. (Reference Li, Jin, Miao and Chen2023, Example 5.2) that
 $(P, \tau ))\times (P, \tau ))$
is not a strong
$(P, \tau ))\times (P, \tau ))$
is not a strong
 $d$
-space. By Lemma 50,
$d$
-space. By Lemma 50,
 $(P, \tau ))\times (P, \tau ))$
is not an R-space.
$(P, \tau ))\times (P, \tau ))$
is not an R-space.
By Example 51 and Li et al. (Reference Li, Jin, Miao and Chen2023, Example 5.2), we pose the following question.
Question 52.
Let
![]() $P, Q$
be dcpos for which
$P, Q$
be dcpos for which
![]() $\Sigma P$
and
$\Sigma P$
and
![]() $\Sigma Q$
are strong
$\Sigma Q$
are strong
![]() $d$
-spaces (resp., R-spaces). Must the product space
$d$
-spaces (resp., R-spaces). Must the product space
![]() $\Sigma P\times \Sigma Q$
be a strong
$\Sigma P\times \Sigma Q$
be a strong
![]() $d$
-space (resp., an R-space)?
$d$
-space (resp., an R-space)?
By Example 51 and MacLane (Reference MacLane1997, pp. 92, Exercise 7) (or Nel and Wilson Reference Nel and Wilson1972, Remark 1.1), we get the following result.
Theorem 53.
The category
![]() $\mathbf{Top}_r$
is not a reflective subcategory of
$\mathbf{Top}_r$
is not a reflective subcategory of
![]() $\mathbf{Top}_0$
.
$\mathbf{Top}_0$
.
For a dcpo
![]() $P$
,
$P$
,
![]() $(P, \upsilon (P))$
and
$(P, \upsilon (P))$
and
![]() $(P, \sigma (P))$
need not be strong
$(P, \sigma (P))$
need not be strong
![]() $d$
-spaces, although they are always
$d$
-spaces, although they are always
![]() $d$
-spaces. Consider the Johnstone’s dcpo
$d$
-spaces. Consider the Johnstone’s dcpo
![]() $\mathbb{J}=\mathbb{N}\times (\mathbb{N}\cup \{\infty \})$
with ordering defined by
$\mathbb{J}=\mathbb{N}\times (\mathbb{N}\cup \{\infty \})$
with ordering defined by
![]() $(m,p)\leq (n,q)$
if
$(m,p)\leq (n,q)$
if
![]() $m=n$
and
$m=n$
and
![]() $p\leq q$
or if
$p\leq q$
or if
![]() $p\leq n$
and
$p\leq n$
and
![]() $q=\infty$
. Then
$q=\infty$
. Then
![]() $(\mathbb{J}, \upsilon (\mathbb{J}))$
and the Johnstone space
$(\mathbb{J}, \upsilon (\mathbb{J}))$
and the Johnstone space
![]() $\Sigma \,\!\mathbb{J}$
are
$\Sigma \,\!\mathbb{J}$
are
![]() $d$
-spaces. Clearly,
$d$
-spaces. Clearly,
![]() $\bigcap _{n\in \mathbb{N}}\mathord{\uparrow } (1, n)\cap \mathord{\uparrow } (2,1)=\emptyset$
, but
$\bigcap _{n\in \mathbb{N}}\mathord{\uparrow } (1, n)\cap \mathord{\uparrow } (2,1)=\emptyset$
, but
![]() $\mathord{\uparrow } (1, n)\cap \mathord{\uparrow } (2,1)=\{(m, \omega ): n\leq m\}\neq \emptyset$
for all
$\mathord{\uparrow } (1, n)\cap \mathord{\uparrow } (2,1)=\{(m, \omega ): n\leq m\}\neq \emptyset$
for all
![]() $n$
. Hence,
$n$
. Hence,
![]() $(\mathbb{J}, \upsilon (\mathbb{J}))$
and
$(\mathbb{J}, \upsilon (\mathbb{J}))$
and
![]() $\Sigma \,\!\mathbb{J}$
are not strong
$\Sigma \,\!\mathbb{J}$
are not strong
![]() $d$
-spaces. Example 58 below shows that there is even an algebraic domain
$d$
-spaces. Example 58 below shows that there is even an algebraic domain
![]() $P$
(hence
$P$
(hence
![]() $\Sigma P$
is sober) such that
$\Sigma P$
is sober) such that
![]() $\Sigma P$
is not a strong
$\Sigma P$
is not a strong
![]() $d$
-space.
$d$
-space.
The following example shows that a locally compact and second-countable strong
![]() $d$
-space may not be a well-filtered space in general (and hence not sober). So a locally compact strong
$d$
-space may not be a well-filtered space in general (and hence not sober). So a locally compact strong
![]() $d$
-space may not be sober (in contrast to Theorem29) and a second-countable strong
$d$
-space may not be sober (in contrast to Theorem29) and a second-countable strong
![]() $d$
-space need not be sober (in contrast to Xu et al. Reference Xu, Shen, Xi and Zhao2020a, Theorem 4.2).
$d$
-space need not be sober (in contrast to Xu et al. Reference Xu, Shen, Xi and Zhao2020a, Theorem 4.2).
Example 54.
Let
![]() $X$
be a countably infinite set and
$X$
be a countably infinite set and
![]() $X_{cof}$
the space equipped with the co-finite topology (the empty set and the complements of finite subsets of
$X_{cof}$
the space equipped with the co-finite topology (the empty set and the complements of finite subsets of
![]() $X$
are open). Then
$X$
are open). Then
-
(a)
 $\mathscr{O}(X_{cof})$
is countable, whence
$\mathscr{O}(X_{cof})$
is countable, whence
 $X_{cof}$
is second-countable.
$X_{cof}$
is second-countable.
-
(b)
 $\mathscr{C}(X_{cof})=\{\emptyset, X\}\bigcup X^{(\lt \omega )}$
,
$\mathscr{C}(X_{cof})=\{\emptyset, X\}\bigcup X^{(\lt \omega )}$
,
 $X_{cof}$
is
$X_{cof}$
is
 $T_1$
and hence a strong
$T_1$
and hence a strong
 $d$
-space.
$d$
-space.
-
(c)
 $\mathord{\mathsf{K}} (X_{cof})=2^X\setminus \{\emptyset \}$
. So
$\mathord{\mathsf{K}} (X_{cof})=2^X\setminus \{\emptyset \}$
. So
 $X_{cof}$
is locally compact.
$X_{cof}$
is locally compact.
-
(d)
 $X_{cof}$
is not well-filtered and hence non-sober. Let
$X_{cof}$
is not well-filtered and hence non-sober. Let
 $\mathscr{K}=\{X\setminus F : F\in X^{(\lt \omega )}\}$
. Then
$\mathscr{K}=\{X\setminus F : F\in X^{(\lt \omega )}\}$
. Then
 $\mathscr{K}$
is a filtered family of compact saturated sets of
$\mathscr{K}$
is a filtered family of compact saturated sets of
 $X_{cof}$
and
$X_{cof}$
and
 $\bigcap \mathscr{K}=\bigcap \limits _{F\in X^{(\lt \omega )}}(X\setminus F)=X\setminus \bigcup X^{(\lt \omega )}=\emptyset$
, but
$\bigcap \mathscr{K}=\bigcap \limits _{F\in X^{(\lt \omega )}}(X\setminus F)=X\setminus \bigcup X^{(\lt \omega )}=\emptyset$
, but
 $X\setminus F\neq \emptyset$
for any
$X\setminus F\neq \emptyset$
for any
 $F\in X^{(\lt \omega )}$
. So
$F\in X^{(\lt \omega )}$
. So
 $X_{cof}$
is not well-filtered.
$X_{cof}$
is not well-filtered.
The following result follows directly from Proposition 11(4).
Proposition 55.
Let
![]() $P$
be a Noetherian dcpo and
$P$
be a Noetherian dcpo and
![]() $\tau$
an order-compatible topology on
$\tau$
an order-compatible topology on
![]() $P$
(i.e.,
$P$
(i.e.,
![]() $\upsilon (P) \subseteq \tau \subseteq \alpha (P)$
). Then
$\upsilon (P) \subseteq \tau \subseteq \alpha (P)$
). Then
![]() $(P, \tau )$
is a strong
$(P, \tau )$
is a strong
![]() $d$
-space.
$d$
-space.
In the following example, we give a Noetherian dcpo
![]() $P$
such that
$P$
such that
![]() $\Sigma P$
is not an R-space, though it is both a strong
$\Sigma P$
is not an R-space, though it is both a strong
![]() $d$
-space and a sober space. It also shows that there exists a Noetherian dcpo
$d$
-space and a sober space. It also shows that there exists a Noetherian dcpo
![]() $P$
such that
$P$
such that
![]() $(P, \tau )$
is not an R-space for any order-compatible topology on
$(P, \tau )$
is not an R-space for any order-compatible topology on
![]() $P$
(in contrast to Proposition 55).
$P$
(in contrast to Proposition 55).
Example 56.
Let
![]() $P=\{a_1, a_2, \ldots, a_n, \ldots \}\cup \{b_1, b_2, \ldots, b_n, \ldots \}$
with the order generated by
$P=\{a_1, a_2, \ldots, a_n, \ldots \}\cup \{b_1, b_2, \ldots, b_n, \ldots \}$
with the order generated by
![]() $a_n\lt b_m$
iff
$a_n\lt b_m$
iff
![]() $n\leq m$
in
$n\leq m$
in
![]() $\mathbb{N}$
and
$\mathbb{N}$
and
![]() $\tau$
an order-compatible topology on
$\tau$
an order-compatible topology on
![]() $P$
. Then
$P$
. Then
![]() $P$
is a Noetherian dcpo. Hence by Proposition 55,
$P$
is a Noetherian dcpo. Hence by Proposition 55,
![]() $(P, \sigma (P))$
is both a strong
$(P, \sigma (P))$
is both a strong
![]() $d$
-space and a sober space. Clearly,
$d$
-space and a sober space. Clearly,
![]() $\bigcap _{n\in \mathbb N}\mathord{\uparrow } a_n=\bigcap _{n\in \mathbb N}(\{a_n\}\cup \{b_m : n\leq m\})=\emptyset$
, but for any finite subset
$\bigcap _{n\in \mathbb N}\mathord{\uparrow } a_n=\bigcap _{n\in \mathbb N}(\{a_n\}\cup \{b_m : n\leq m\})=\emptyset$
, but for any finite subset
![]() $\{n_1, n_2, \ldots, n_m\}$
of
$\{n_1, n_2, \ldots, n_m\}$
of
![]() $\mathbb N$
,
$\mathbb N$
,
![]() $\bigcap \limits _{j=1}^{m}\mathord{\uparrow } a_{n_j}\supseteq \{b_l : \mbox{max}\{n_1, n_2, \ldots, n_m\}\leq l\}\neq \emptyset$
. So
$\bigcap \limits _{j=1}^{m}\mathord{\uparrow } a_{n_j}\supseteq \{b_l : \mbox{max}\{n_1, n_2, \ldots, n_m\}\leq l\}\neq \emptyset$
. So
![]() $(P, \tau )$
is not an R-space. In particular, neither
$(P, \tau )$
is not an R-space. In particular, neither
![]() $(P, \upsilon (P))$
nor
$(P, \upsilon (P))$
nor
![]() $\Sigma P$
is an R-space.
$\Sigma P$
is an R-space.
Proposition 57.
Let
![]() $X$
be a well-filtered space and
$X$
be a well-filtered space and
![]() $\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for all
$\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for all
![]() $x, y\in X$
. Then
$x, y\in X$
. Then
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space.
$d$
-space.
Proof. Let
![]() $D\in \mathscr{D}(X)$
,
$D\in \mathscr{D}(X)$
,
![]() $x\in X$
and
$x\in X$
and
![]() $U\in \mathscr{O}(X)$
such that
$U\in \mathscr{O}(X)$
such that
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
holds. If there is
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
holds. If there is
![]() $d_0\in D$
such that
$d_0\in D$
such that
![]() $\mathord{\uparrow } d_0\cap \mathord{\uparrow } x=\emptyset$
, then
$\mathord{\uparrow } d_0\cap \mathord{\uparrow } x=\emptyset$
, then
![]() $\mathord{\uparrow } d_0\cap \mathord{\uparrow } x\subseteq U$
. Now assume that
$\mathord{\uparrow } d_0\cap \mathord{\uparrow } x\subseteq U$
. Now assume that
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\neq \emptyset$
for all
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\neq \emptyset$
for all
![]() $d\in D$
. Then by assumption
$d\in D$
. Then by assumption
![]() $\{\mathord{\uparrow } d\cap \mathord{\uparrow } x : d\in D\}$
is a filtered family of compact saturated subsets of
$\{\mathord{\uparrow } d\cap \mathord{\uparrow } x : d\in D\}$
is a filtered family of compact saturated subsets of
![]() $X$
and
$X$
and
![]() $\bigcap _{d\in D}(\mathord{\uparrow } d\cap \mathord{\uparrow } x)= \bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
. By the well-filteredness of
$\bigcap _{d\in D}(\mathord{\uparrow } d\cap \mathord{\uparrow } x)= \bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
. By the well-filteredness of
![]() $X$
, there is
$X$
, there is
![]() $d\in D$
such that
$d\in D$
such that
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
. So
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
. So
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space.
$d$
-space.
The following example shows that even for an algebraic domain
![]() $P$
, if the condition that
$P$
, if the condition that
![]() $\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for all
$\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is compact for all
![]() $x, y\in P$
is not satisfied,
$x, y\in P$
is not satisfied,
![]() $\Sigma P$
may not be a strong
$\Sigma P$
may not be a strong
![]() $d$
-space. It also shows that a sober space need not be a strong
$d$
-space. It also shows that a sober space need not be a strong
![]() $d$
-space, and hence, well-filtered spaces and
$d$
-space, and hence, well-filtered spaces and
![]() $d$
-spaces are generally not strong
$d$
-spaces are generally not strong
![]() $d$
-spaces.
$d$
-spaces.
Example 58.
Let
![]() $C=\{a_1, a_2, \ldots, a_n, \ldots \}\cup \{\omega _0\}$
and
$C=\{a_1, a_2, \ldots, a_n, \ldots \}\cup \{\omega _0\}$
and
![]() $P=C\cup \{b\}\cup \{\omega _1, \ldots, \omega _n, \ldots \}$
. Define an order on
$P=C\cup \{b\}\cup \{\omega _1, \ldots, \omega _n, \ldots \}$
. Define an order on
![]() $P$
as follows (see Fig.
3
):
$P$
as follows (see Fig.
3
):
-
(i)
 $a_1\lt a_2\lt \ldots \lt a_n\lt a_{n+1}\lt \ldots$
;
$a_1\lt a_2\lt \ldots \lt a_n\lt a_{n+1}\lt \ldots$
; -
(ii)
 $a_n\lt \omega _0$
for all
$a_n\lt \omega _0$
for all
 $n\in \mathbb{N}$
;
$n\in \mathbb{N}$
; -
(iii)
 $b\lt \omega _n$
and
$b\lt \omega _n$
and
 $a_m\lt \omega _n$
for all
$a_m\lt \omega _n$
for all
 $n, m\in \mathbb{N}$
with
$n, m\in \mathbb{N}$
with
 $m\leq n$
.
$m\leq n$
.

Figure 3. The poset
![]() $P$
in Example 58.
$P$
in Example 58.
-
(a)
 $D\in \mathscr{D}(P)$
iff
$D\in \mathscr{D}(P)$
iff
 $D\subseteq C$
is an infinite chain or
$D\subseteq C$
is an infinite chain or
 $D$
has a largest element. So
$D$
has a largest element. So
 $P$
is a dcpo. Clearly,
$P$
is a dcpo. Clearly,
 $\textrm{max}(P)=\{\omega _0, \omega _1, \omega _2, \ldots, \omega _n, \ldots \}$
. If
$\textrm{max}(P)=\{\omega _0, \omega _1, \omega _2, \ldots, \omega _n, \ldots \}$
. If
 $D\subseteq C$
is an infinite chain or
$D\subseteq C$
is an infinite chain or
 $D$
has a largest element, then
$D$
has a largest element, then
 $D\in \mathscr{D}(P)$
. Conversely, suppose
$D\in \mathscr{D}(P)$
. Conversely, suppose
 $D$
is a directed subset of
$D$
is a directed subset of
 $P$
and
$P$
and
 $D$
has no largest element. Then
$D$
has no largest element. Then
 $D$
is countably infinite and
$D$
is countably infinite and
 $|D\cap \textrm{max}(P)|\lt 2$
. If there exits
$|D\cap \textrm{max}(P)|\lt 2$
. If there exits
 $\omega _m\in D$
for some
$\omega _m\in D$
for some
 $m\in \mathbb{N}$
, then for any
$m\in \mathbb{N}$
, then for any
 $d\in D$
, by the directedness of
$d\in D$
, by the directedness of
 $D$
, there is
$D$
, there is
 $d^{\prime }\in D$
such that
$d^{\prime }\in D$
such that
 $d\leq d^{\prime }$
and
$d\leq d^{\prime }$
and
 $\omega _m\leq d^{\prime }$
. Then
$\omega _m\leq d^{\prime }$
. Then
 $d\leq d^{\prime }=\omega _m$
. So
$d\leq d^{\prime }=\omega _m$
. So
 $\omega _m$
is the largest element of
$\omega _m$
is the largest element of
 $D$
, a contradiction. Hence,
$D$
, a contradiction. Hence,
 $D\cap \textrm{max}(P)=\emptyset$
, that is,
$D\cap \textrm{max}(P)=\emptyset$
, that is,
 $D\subseteq C\cup \{b\}$
. Since for any
$D\subseteq C\cup \{b\}$
. Since for any
 $n\in \mathbb{N}$
,
$n\in \mathbb{N}$
,
 $a_n$
and
$a_n$
and
 $b$
have no upper bound in
$b$
have no upper bound in
 $D$
, we have
$D$
, we have
 $b\not \in D$
, and hence,
$b\not \in D$
, and hence,
 $D\subseteq C$
is an infinite chain.
$D\subseteq C$
is an infinite chain.
-
(b)
 $P$
is an algebraic domain. By (a),
$P$
is an algebraic domain. By (a),
 $x\ll x$
for all
$x\ll x$
for all
 $x\in P\setminus \{\omega _0\}$
. Hence,
$x\in P\setminus \{\omega _0\}$
. Hence,
 $P$
is an algebraic domain.
$P$
is an algebraic domain.
-
(c)
 $\Sigma P$
is sober and not coherent. By (b) and Proposition III-3.7 of Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003) or Proposition 4.4 of Gierz et al. (Reference Gierz, Lawson and Stralka1983),
$\Sigma P$
is sober and not coherent. By (b) and Proposition III-3.7 of Gierz et al. (Reference Gierz, Hofmann, Keimel, Lawson, Mislove and Scott2003) or Proposition 4.4 of Gierz et al. (Reference Gierz, Lawson and Stralka1983),
 $\Sigma P$
is sober. Clearly,
$\Sigma P$
is sober. Clearly,
 $\mathord{\uparrow } a_1, \mathord{\uparrow } b\in \mathord{\mathsf{K}} (\Sigma \,)$
, but
$\mathord{\uparrow } a_1, \mathord{\uparrow } b\in \mathord{\mathsf{K}} (\Sigma \,)$
, but
 $\mathord{\uparrow } a_1\cap \mathord{\uparrow } b=\{\omega _1, \omega _2, \ldots, \omega _n, \ldots \}$
is not Scott-compact (note
$\mathord{\uparrow } a_1\cap \mathord{\uparrow } b=\{\omega _1, \omega _2, \ldots, \omega _n, \ldots \}$
is not Scott-compact (note
 $\{\omega _n\}\in \sigma (P)$
for all
$\{\omega _n\}\in \sigma (P)$
for all
 $n\in \mathbb{N}$
) and hence
$n\in \mathbb{N}$
) and hence
 $\Sigma P$
is not coherent.
$\Sigma P$
is not coherent.
-
(d)
 $\Sigma P$
is not a strong
$\Sigma P$
is not a strong
 $d$
-space. Since
$d$
-space. Since
 $\bigcap _{n\in \mathbb{N}}\mathord{\uparrow } a_n\cap \mathord{\uparrow } b=\emptyset$
but
$\bigcap _{n\in \mathbb{N}}\mathord{\uparrow } a_n\cap \mathord{\uparrow } b=\emptyset$
but
 $\mathord{\uparrow } a_m\cap \mathord{\uparrow } b=\{\omega _m, \omega _{m+1}, \ldots \}\neq \emptyset$
for all
$\mathord{\uparrow } a_m\cap \mathord{\uparrow } b=\{\omega _m, \omega _{m+1}, \ldots \}\neq \emptyset$
for all
 $m\in \mathbb N$
,
$m\in \mathbb N$
,
 $P$
with any order-compatible topology is not a strong
$P$
with any order-compatible topology is not a strong
 $d$
-space. In particular,
$d$
-space. In particular,
 $(P, \upsilon (P))$
and
$(P, \upsilon (P))$
and
 $\Sigma P$
are not strong
$\Sigma P$
are not strong
 $d$
-spaces.
$d$
-spaces.
Remark 59.
Let
![]() $\hat{P}$
be the poset obtained from
$\hat{P}$
be the poset obtained from
![]() $P$
by adding an order relation
$P$
by adding an order relation
![]() $b\lt \omega _0$
(see Fig.
4
). Then
$b\lt \omega _0$
(see Fig.
4
). Then
![]() $\Sigma \hat{P}$
is a strong
$\Sigma \hat{P}$
is a strong
![]() $d$
-space. We will give a short proof. Suppose
$d$
-space. We will give a short proof. Suppose
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
, where
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
, where
![]() $D\in \mathscr{D}(\hat{P})$
,
$D\in \mathscr{D}(\hat{P})$
,
![]() $x\in P$
and
$x\in P$
and
![]() $U\in \mathcal \sigma (\hat{P})$
. If
$U\in \mathcal \sigma (\hat{P})$
. If
![]() $D$
has a largest element
$D$
has a largest element
![]() $s$
, then
$s$
, then
![]() $\mathord{\uparrow } s\cap \mathord{\uparrow } x=\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
. Now assume that
$\mathord{\uparrow } s\cap \mathord{\uparrow } x=\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq U$
. Now assume that
![]() $D$
has no largest element. Then
$D$
has no largest element. Then
![]() $D\subseteq C=\{a_1, a_2, \ldots, a_n, \ldots \}$
is an infinite chain and
$D\subseteq C=\{a_1, a_2, \ldots, a_n, \ldots \}$
is an infinite chain and
![]() $\omega _0=\bigvee D$
. Hence,
$\omega _0=\bigvee D$
. Hence,
![]() $\bigcap _{d\in D}\mathord{\uparrow } d=\{\omega _0\}$
. If
$\bigcap _{d\in D}\mathord{\uparrow } d=\{\omega _0\}$
. If
![]() $x=\omega _0$
, then
$x=\omega _0$
, then
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x=\{\omega _0\}\subseteq U$
and hence
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x=\{\omega _0\}\subseteq U$
and hence
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x=\{\omega _0\}\subseteq U$
for all
$\mathord{\uparrow } d\cap \mathord{\uparrow } x=\{\omega _0\}\subseteq U$
for all
![]() $d\in D$
. If
$d\in D$
. If
![]() $x=\omega _m$
for some
$x=\omega _m$
for some
![]() $m\in \mathbb{N}\setminus \{0\}$
, then
$m\in \mathbb{N}\setminus \{0\}$
, then
![]() $x\not \in \{\omega _0\}=\bigcap _{d\in D}\mathord{\uparrow } d$
and hence there is a
$x\not \in \{\omega _0\}=\bigcap _{d\in D}\mathord{\uparrow } d$
and hence there is a
![]() $d\in D$
such that
$d\in D$
such that
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x=\emptyset \subseteq U$
. If
$\mathord{\uparrow } d\cap \mathord{\uparrow } x=\emptyset \subseteq U$
. If
![]() $x\in C\cup \{b\}$
, then
$x\in C\cup \{b\}$
, then
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x=\{\omega _0\}\subseteq U$
. As
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x=\{\omega _0\}\subseteq U$
. As
![]() $\omega _0=\bigvee D$
, there is
$\omega _0=\bigvee D$
, there is
![]() $d\in D$
with
$d\in D$
with
![]() $\mathord{\uparrow } d\subseteq U$
, whence
$\mathord{\uparrow } d\subseteq U$
, whence
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq \mathord{\uparrow } d\subseteq U$
. Thus,
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\subseteq \mathord{\uparrow } d\subseteq U$
. Thus,
![]() $\Sigma \hat{P}$
is a strong
$\Sigma \hat{P}$
is a strong
![]() $d$
-space.
$d$
-space.

Figure 4. The poset
![]() $\hat{P}$
in Remark 59.
$\hat{P}$
in Remark 59.
Now we give some characterizations of strong
![]() $d$
-spaces. In the following, when a
$d$
-spaces. In the following, when a
![]() $T_0$
-space is also considered as a poset, the order refers to the specialization order.
$T_0$
-space is also considered as a poset, the order refers to the specialization order.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space and
$T_0$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
closed under arbitrary intersections such that
$X$
closed under arbitrary intersections such that
![]() $\mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}\subseteq \mathscr{G}\subseteq \mathbf{up}(X)$
. Endow
$\mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}\subseteq \mathscr{G}\subseteq \mathbf{up}(X)$
. Endow
![]() $\mathscr{G}$
with the reverse inclusion order
$\mathscr{G}$
with the reverse inclusion order
![]() $\sqsubseteq$
, that is, for
$\sqsubseteq$
, that is, for
![]() $G_1, G_2\in \mathscr{G}$
,
$G_1, G_2\in \mathscr{G}$
,
![]() $G_1\sqsubseteq G_2$
iff
$G_1\sqsubseteq G_2$
iff
![]() $G_2\subseteq G_1$
. Denote
$G_2\subseteq G_1$
. Denote
![]() $\mathscr{G}$
equipped with the Scott topology for this order by
$\mathscr{G}$
equipped with the Scott topology for this order by
![]() $\Sigma \mathscr{G}$
. For any
$\Sigma \mathscr{G}$
. For any
![]() $x\in X$
, it is straightforward to verify that the mapping
$x\in X$
, it is straightforward to verify that the mapping
![]() $y\mapsto \mathord{\uparrow } y\cap \mathord{\uparrow } x : X \rightarrow \mathscr{G}$
is Scott continuous. Furthermore, the mapping
$y\mapsto \mathord{\uparrow } y\cap \mathord{\uparrow } x : X \rightarrow \mathscr{G}$
is Scott continuous. Furthermore, the mapping
![]() $m_{\sigma } : X\times X\rightarrow \mathscr{G}, (x,y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is Scott continuous. Indeed, if
$m_{\sigma } : X\times X\rightarrow \mathscr{G}, (x,y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is Scott continuous. Indeed, if
![]() $\{(x_{d},y_{d}):d\in D\}\in \mathscr{D} (X\times X)$
for which
$\{(x_{d},y_{d}):d\in D\}\in \mathscr{D} (X\times X)$
for which
![]() $\bigvee _{d\in D}(x_d, y_d)$
exists in
$\bigvee _{d\in D}(x_d, y_d)$
exists in
![]() $X\times X$
, then
$X\times X$
, then
![]() $\{x_{d}:d\in D\}\in \mathscr{D}(X)$
,
$\{x_{d}:d\in D\}\in \mathscr{D}(X)$
,
![]() $\{y_{d}:d\in D\}\in \mathscr{D}(X)$
, and
$\{y_{d}:d\in D\}\in \mathscr{D}(X)$
, and
![]() $\bigvee _{d\in D}x_d$
and
$\bigvee _{d\in D}x_d$
and
![]() $\bigvee _{d\in D} y_d$
exist in
$\bigvee _{d\in D} y_d$
exist in
![]() $X$
. Clearly,
$X$
. Clearly,
![]() $m_{\sigma }(\bigvee _{d\in D}(x_{d},y_{d}))=m_{\sigma }((\bigvee _{d\in D}x_{d},\bigvee _{d\in D}y_{d}))=(\mathord{\uparrow } \bigvee _{d\in D}x_{d})\cap (\mathord{\uparrow }\bigvee _{d\in D}y_{d})=(\bigcap _{d\in D}\mathord{\uparrow } x_{d})\cap (\bigcap _{d\in D}\mathord{\uparrow } y_{d})=\bigcap _{d\in D}(\mathord{\uparrow } x_{d}\cap \mathord{\uparrow } y_{d})=\bigcap _{d\in D}m_{\sigma }(x_{d},y_{d})=\bigvee _{\mathscr{G}}\{m_{\sigma }(x_{d},y_{d}) : d\in D\}$
. By Lemma 4,
$m_{\sigma }(\bigvee _{d\in D}(x_{d},y_{d}))=m_{\sigma }((\bigvee _{d\in D}x_{d},\bigvee _{d\in D}y_{d}))=(\mathord{\uparrow } \bigvee _{d\in D}x_{d})\cap (\mathord{\uparrow }\bigvee _{d\in D}y_{d})=(\bigcap _{d\in D}\mathord{\uparrow } x_{d})\cap (\bigcap _{d\in D}\mathord{\uparrow } y_{d})=\bigcap _{d\in D}(\mathord{\uparrow } x_{d}\cap \mathord{\uparrow } y_{d})=\bigcap _{d\in D}m_{\sigma }(x_{d},y_{d})=\bigvee _{\mathscr{G}}\{m_{\sigma }(x_{d},y_{d}) : d\in D\}$
. By Lemma 4,
![]() $m_{\sigma }:\Sigma (X\times X) \rightarrow \Sigma \mathscr{G}$
is continuous.
$m_{\sigma }:\Sigma (X\times X) \rightarrow \Sigma \mathscr{G}$
is continuous.
Proposition 60.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space and
$T_0$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
closed under arbitrary intersections such that
$X$
closed under arbitrary intersections such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. Then the following four conditions are equivalent:
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. Then the following four conditions are equivalent:
-
(1) For any
 $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
 $x\in X$
,
$x\in X$
,
 $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. -
(2) For any
 $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
 $K\in \mathord{\mathsf{K}} (\Sigma \,\!\!X)$
,
$K\in \mathord{\mathsf{K}} (\Sigma \,\!\!X)$
,
 $\mathord{\downarrow } (K\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (K\cap A)\in \mathscr{C}(\Sigma X)$
. -
(3) For all
 $x\in X$
, the mapping
$x\in X$
, the mapping
 $m_x : \Sigma X \rightarrow P_S(\mathscr{G}), y\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m_x : \Sigma X \rightarrow P_S(\mathscr{G}), y\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
-
(4) The mapping
 $m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
Proof. (1)
![]() $\Leftrightarrow$
(2): It is proved in Xu and Zhao (Reference Xu and Zhao2020) (see the proof of Xu and Zhao Reference Xu and Zhao2020, Lemma 3.15) for
$\Leftrightarrow$
(2): It is proved in Xu and Zhao (Reference Xu and Zhao2020) (see the proof of Xu and Zhao Reference Xu and Zhao2020, Lemma 3.15) for
![]() $d$
-spaces and the proof is valid for general
$d$
-spaces and the proof is valid for general
![]() $T_0$
-spaces.
$T_0$
-spaces.
(1)
![]() $\Rightarrow$
(3): For
$\Rightarrow$
(3): For
![]() $U\in \mathscr{O}(X)$
, we show that
$U\in \mathscr{O}(X)$
, we show that
![]() $m_x^{-1}(\Box _{\mathscr{G}}U)=\{y\in X : \mathord{\uparrow } x\cap \mathord{\uparrow } y\subseteq U\}\in \sigma (X)$
. Clearly,
$m_x^{-1}(\Box _{\mathscr{G}}U)=\{y\in X : \mathord{\uparrow } x\cap \mathord{\uparrow } y\subseteq U\}\in \sigma (X)$
. Clearly,
![]() $m_x^{-1}(\Box _{\mathscr{G}}U)$
is an upper set of
$m_x^{-1}(\Box _{\mathscr{G}}U)$
is an upper set of
![]() $P$
. Suppose that
$P$
. Suppose that
![]() $D\in \mathscr{D}(P)$
with
$D\in \mathscr{D}(P)$
with
![]() $\bigvee D$
existing and
$\bigvee D$
existing and
![]() $\bigvee D\in m_x^{-1}(\Box _{\mathscr{G}}U)$
. Then
$\bigvee D\in m_x^{-1}(\Box _{\mathscr{G}}U)$
. Then
![]() $\mathord{\uparrow } x\cap \mathord{\uparrow } \bigvee D \subseteq U$
. Let
$\mathord{\uparrow } x\cap \mathord{\uparrow } \bigvee D \subseteq U$
. Let
![]() $A=X\setminus U$
. Then
$A=X\setminus U$
. Then
![]() $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
![]() $(\mathord{\uparrow } \bigvee D) \cap \mathord{\uparrow } x\cap A=\emptyset$
. Hence,
$(\mathord{\uparrow } \bigvee D) \cap \mathord{\uparrow } x\cap A=\emptyset$
. Hence,
![]() $\bigvee D\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
by (1). It follows that
$\bigvee D\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
by (1). It follows that
![]() $D\not \subseteq \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
, and consequently, there is
$D\not \subseteq \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
, and consequently, there is
![]() $d\in D$
such that
$d\in D$
such that
![]() $d\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. So
$d\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. So
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A=\emptyset$
, that is,
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A=\emptyset$
, that is,
![]() $d\in m_x^{-1}(\Box _{\mathscr{G}}U)$
. Thus,
$d\in m_x^{-1}(\Box _{\mathscr{G}}U)$
. Thus,
![]() $m_x^{-1}(\Box _{\mathscr{G}}U)\in \sigma (P)$
. Therefore,
$m_x^{-1}(\Box _{\mathscr{G}}U)\in \sigma (P)$
. Therefore,
![]() $m_x : \Sigma P \rightarrow P_S(\mathscr{G})$
is continuous.
$m_x : \Sigma P \rightarrow P_S(\mathscr{G})$
is continuous.
(3)
![]() $\Rightarrow$
(4): Let
$\Rightarrow$
(4): Let
![]() $U\in \sigma (X)$
. We show that
$U\in \sigma (X)$
. We show that
![]() $m^{-1}(\Box _{\mathscr{G}}U)=\{(x, y)\in X\times X : \mathord{\uparrow } x\cap \mathord{\uparrow } y\subseteq U\}\in \sigma (X\times X)$
. Clearly,
$m^{-1}(\Box _{\mathscr{G}}U)=\{(x, y)\in X\times X : \mathord{\uparrow } x\cap \mathord{\uparrow } y\subseteq U\}\in \sigma (X\times X)$
. Clearly,
![]() $m^{-1}(\Box _{\mathscr{G}}U)$
is an upper set of
$m^{-1}(\Box _{\mathscr{G}}U)$
is an upper set of
![]() $X\times X$
. Suppose that
$X\times X$
. Suppose that
![]() $D\in \mathscr{D}(X\times X)$
such that
$D\in \mathscr{D}(X\times X)$
such that
![]() $\bigvee D$
existing and
$\bigvee D$
existing and
![]() $\bigvee D\in m^{-1}(\Box _{\mathscr{G}}U)$
. Let
$\bigvee D\in m^{-1}(\Box _{\mathscr{G}}U)$
. Let
![]() $p_i : X\times X \rightarrow X$
be the
$p_i : X\times X \rightarrow X$
be the
![]() $i$
th projection (
$i$
th projection (
![]() $i=1, 2$
). Then
$i=1, 2$
). Then
![]() $D_i=p_i(D)$
is a directed subset of
$D_i=p_i(D)$
is a directed subset of
![]() $X$
(
$X$
(
![]() $i=1, 2$
) and
$i=1, 2$
) and
![]() $\bigvee D=(\bigvee D_1, \bigvee D_2)$
. Hence,
$\bigvee D=(\bigvee D_1, \bigvee D_2)$
. Hence,
![]() $m_{\bigvee D_2}(\bigvee D_1)=(\mathord{\uparrow } \bigvee D_1)\cap (\mathord{\uparrow } \bigvee D_2)=m(\bigvee D)\in \Box _{\mathscr{G}}U$
. By (3), there is
$m_{\bigvee D_2}(\bigvee D_1)=(\mathord{\uparrow } \bigvee D_1)\cap (\mathord{\uparrow } \bigvee D_2)=m(\bigvee D)\in \Box _{\mathscr{G}}U$
. By (3), there is
![]() $d_1\in D$
such that
$d_1\in D$
such that
![]() $m_{p_1(d_1)}(\bigvee D_2)=(\mathord{\uparrow } p_1(d_1))\cap (\mathord{\uparrow } \bigvee D_2)\in \Box _{\mathscr{G}}U$
. By (3) again, there is
$m_{p_1(d_1)}(\bigvee D_2)=(\mathord{\uparrow } p_1(d_1))\cap (\mathord{\uparrow } \bigvee D_2)\in \Box _{\mathscr{G}}U$
. By (3) again, there is
![]() $d_2\in D$
such that
$d_2\in D$
such that
![]() $(\mathord{\uparrow } p_1(d_1))\cap (\mathord{\uparrow } p_2(d_2))\in \Box _{\mathscr{G}}U$
. By the directedness of
$(\mathord{\uparrow } p_1(d_1))\cap (\mathord{\uparrow } p_2(d_2))\in \Box _{\mathscr{G}}U$
. By the directedness of
![]() $D$
, there is
$D$
, there is
![]() $d_3\in D$
with
$d_3\in D$
with
![]() $d_1\leq d_3$
and
$d_1\leq d_3$
and
![]() $d_2\leq d_3$
. Then
$d_2\leq d_3$
. Then
![]() $m(d_3)=(\mathord{\uparrow } p_1(d_3))\cap (\mathord{\uparrow } p_2(d_3))\subseteq (\mathord{\uparrow } p_1(d_1))\cap (\mathord{\uparrow } p_2(d_2))\in \Box _{\mathscr{G}}U$
and hence
$m(d_3)=(\mathord{\uparrow } p_1(d_3))\cap (\mathord{\uparrow } p_2(d_3))\subseteq (\mathord{\uparrow } p_1(d_1))\cap (\mathord{\uparrow } p_2(d_2))\in \Box _{\mathscr{G}}U$
and hence
![]() $d_3\in m^{-1}(\Box _{\mathscr{G}}U)$
. Thus,
$d_3\in m^{-1}(\Box _{\mathscr{G}}U)$
. Thus,
![]() $m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G})$
is continuous.
$m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G})$
is continuous.
(4)
![]() $\Rightarrow$
(1): For
$\Rightarrow$
(1): For
![]() $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
![]() $x\in X$
, we show that
$x\in X$
, we show that
![]() $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. Suppose that
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. Suppose that
![]() $D$
is a directed subset of
$D$
is a directed subset of
![]() $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
with
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
with
![]() $\bigvee D$
existing. If
$\bigvee D$
existing. If
![]() $\bigvee D\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. Then
$\bigvee D\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. Then
![]() $U=X\setminus A\in \mathscr{O}(X)$
and
$U=X\setminus A\in \mathscr{O}(X)$
and
![]() $(\mathord{\uparrow } \bigvee D)\cap \mathord{\uparrow } x\subseteq U$
. Let
$(\mathord{\uparrow } \bigvee D)\cap \mathord{\uparrow } x\subseteq U$
. Let
![]() $D_x=\{(d, x) : d\in D\}$
. Then
$D_x=\{(d, x) : d\in D\}$
. Then
![]() $D_x\in \mathscr{D}(X\times X)$
and
$D_x\in \mathscr{D}(X\times X)$
and
![]() $m(\bigvee D_x)=m((\bigvee D, x))=(\mathord{\uparrow } \bigvee D)\cap \mathord{\uparrow } x \subseteq U$
. Hence,
$m(\bigvee D_x)=m((\bigvee D, x))=(\mathord{\uparrow } \bigvee D)\cap \mathord{\uparrow } x \subseteq U$
. Hence,
![]() $\bigvee D_x\in m^{-1}(\Box _{\mathscr{G}}U)\in \sigma (X\times X)$
by (4). It follows that
$\bigvee D_x\in m^{-1}(\Box _{\mathscr{G}}U)\in \sigma (X\times X)$
by (4). It follows that
![]() $(d, x)\in m^{-1}(\Box _{\mathscr{G}}U)$
for some
$(d, x)\in m^{-1}(\Box _{\mathscr{G}}U)$
for some
![]() $d\in D$
, that is,
$d\in D$
, that is,
![]() $d\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
, a contradiction, proving that
$d\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
, a contradiction, proving that
![]() $\bigvee D\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. So
$\bigvee D\not \in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. So
![]() $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
Based on Proposition 60, we obtain the following characterizations of strong
![]() $d$
-spaces.
$d$
-spaces.
Theorem 61.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space and
$T_0$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
closed under intersection such that
$X$
closed under intersection such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in X\}$
. Then the following conditions are equivalent:
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in X\}$
. Then the following conditions are equivalent:
-
(1)
 $X$
is a strong
$X$
is a strong
 $d$
-space.
$d$
-space.
-
(2) For any
 $D\in \mathscr{D}(X)$
,
$D\in \mathscr{D}(X)$
,
 $\mathord{\uparrow } F\in \mathbf{Fin} X$
and
$\mathord{\uparrow } F\in \mathbf{Fin} X$
and
 $U\in \mathscr{O}(X)$
,
$U\in \mathscr{O}(X)$
,
 $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
implies
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
implies
 $\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
for some
$\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
for some
 $d\in D$
.
$d\in D$
. -
(3)
 $X$
is a
$X$
is a
 $d$
-space, and for any
$d$
-space, and for any
 $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
 $x\in X$
,
$x\in X$
,
 $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. -
(4)
 $X$
is a dcpo, and for any
$X$
is a dcpo, and for any
 $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
 $x\in X$
,
$x\in X$
,
 $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. -
(5)
 $X$
is a dcpo, and for any
$X$
is a dcpo, and for any
 $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
 $K\in \mathord{\mathsf{K}} (\Sigma \,\!\!X)$
,
$K\in \mathord{\mathsf{K}} (\Sigma \,\!\!X)$
,
 $\mathord{\downarrow } (K\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (K\cap A)\in \mathscr{C}(\Sigma X)$
. -
(6)
 $X$
is a dcpo, and for all
$X$
is a dcpo, and for all
 $x\in X$
, the mapping
$x\in X$
, the mapping
 $m_x : \Sigma X \rightarrow P_S(\mathscr{G}), y\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m_x : \Sigma X \rightarrow P_S(\mathscr{G}), y\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
-
(7)
 $X$
is a dcpo, and the mapping
$X$
is a dcpo, and the mapping
 $m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
Proof. (1)
![]() $\Leftrightarrow$
(2): Obviously, (2)
$\Leftrightarrow$
(2): Obviously, (2)
![]() $\Rightarrow$
(1). Conversely, suppose that
$\Rightarrow$
(1). Conversely, suppose that
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space,
$d$
-space,
![]() $D\in \mathscr{D}(X)$
,
$D\in \mathscr{D}(X)$
,
![]() $\mathord{\uparrow } F\in \mathbf{Fin} X$
and
$\mathord{\uparrow } F\in \mathbf{Fin} X$
and
![]() $U\in \mathscr{O}(X)$
such that
$U\in \mathscr{O}(X)$
such that
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
. Then for each
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
. Then for each
![]() $u\in F$
,
$u\in F$
,
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } u\subseteq U$
, and hence
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } u\subseteq U$
, and hence
![]() $\mathord{\uparrow } d_u\cap \mathord{\uparrow } u\subseteq U$
for some
$\mathord{\uparrow } d_u\cap \mathord{\uparrow } u\subseteq U$
for some
![]() $d_u\in D$
. Since
$d_u\in D$
. Since
![]() $F$
is finite and
$F$
is finite and
![]() $D$
is a direct subset of
$D$
is a direct subset of
![]() $X$
, there is a
$X$
, there is a
![]() $d_0\in D$
such that
$d_0\in D$
such that
![]() $\mathord{\uparrow } d_0\subseteq \bigcap _{u\in F}\mathord{\uparrow } d_u$
. It follows that
$\mathord{\uparrow } d_0\subseteq \bigcap _{u\in F}\mathord{\uparrow } d_u$
. It follows that
![]() $\mathord{\uparrow } d_0\cap \mathord{\uparrow } F=\bigcup _{u\in F}\mathord{\uparrow } d_0\cap \mathord{\uparrow } u\subseteq \bigcup _{u\in F}\mathord{\uparrow } d_u\cap \mathord{\uparrow } u\subseteq U$
.
$\mathord{\uparrow } d_0\cap \mathord{\uparrow } F=\bigcup _{u\in F}\mathord{\uparrow } d_0\cap \mathord{\uparrow } u\subseteq \bigcup _{u\in F}\mathord{\uparrow } d_u\cap \mathord{\uparrow } u\subseteq U$
.
(1)
![]() $\Rightarrow$
(3): Suppose that
$\Rightarrow$
(3): Suppose that
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space. Then by Proposition 20,
$d$
-space. Then by Proposition 20,
![]() $X$
is a
$X$
is a
![]() $d$
-space and hence
$d$
-space and hence
![]() $X$
is a dcpo. For
$X$
is a dcpo. For
![]() $A\in \mathscr{C}(X)$
and
$A\in \mathscr{C}(X)$
and
![]() $x\in X$
, we show that
$x\in X$
, we show that
![]() $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. Let
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. Let
![]() $D\in \mathscr{D}(X)$
with
$D\in \mathscr{D}(X)$
with
![]() $D\subseteq \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. Then
$D\subseteq \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. Then
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
for all
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
for all
![]() $d\in D$
. As
$d\in D$
. As
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space,
$d$
-space,
![]() $\bigcap _{d\in D}(\mathord{\uparrow } d\cap \mathord{\uparrow } x)\cap A\neq \emptyset$
or, equivalently,
$\bigcap _{d\in D}(\mathord{\uparrow } d\cap \mathord{\uparrow } x)\cap A\neq \emptyset$
or, equivalently,
![]() $\uparrow \bigvee D \cap \mathord{\uparrow } x\cap A\neq \emptyset$
. Hence,
$\uparrow \bigvee D \cap \mathord{\uparrow } x\cap A\neq \emptyset$
. Hence,
![]() $\bigvee D\in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. So
$\bigvee D\in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. So
![]() $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
(3)
![]() $\Rightarrow$
(4): Trivial.
$\Rightarrow$
(4): Trivial.
(4)
![]() $\Leftrightarrow$
(5)
$\Leftrightarrow$
(5)
![]() $\Leftrightarrow$
(6)
$\Leftrightarrow$
(6)
![]() $\Leftrightarrow$
(7): By Proposition 60.
$\Leftrightarrow$
(7): By Proposition 60.
(4)
![]() $\Rightarrow$
(1): Let
$\Rightarrow$
(1): Let
![]() $D\in \mathscr{D}(X)$
,
$D\in \mathscr{D}(X)$
,
![]() $x\in X$
and
$x\in X$
and
![]() $A\in \mathscr{C}(X)$
. If
$A\in \mathscr{C}(X)$
. If
![]() $\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
for all
$\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
for all
![]() $d\in D$
, then
$d\in D$
, then
![]() $D\subseteq \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. By (4),
$D\subseteq \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. By (4),
![]() $\bigvee D\in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
, namely,
$\bigvee D\in \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
, namely,
![]() $\bigcap _{d\in D}\mathord{\uparrow } d \cap \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. Hence,
$\bigcap _{d\in D}\mathord{\uparrow } d \cap \mathord{\downarrow } (\mathord{\uparrow } x\cap A)$
. Hence,
![]() $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
. Thus,
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } x\cap A\neq \emptyset$
. Thus,
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space.
$d$
-space.
Remark 62.
Let
![]() $X$
be a
$X$
be a
![]() $T_0$
-space and
$T_0$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
closed under intersection such that
$X$
closed under intersection such that
![]() $\mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in X\}\subseteq \mathscr{G}\subseteq \mathbf{up}(P)$
. It is easy to verify that the specialization order of
$\mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in X\}\subseteq \mathscr{G}\subseteq \mathbf{up}(P)$
. It is easy to verify that the specialization order of
![]() $P_S(\mathscr{G})$
is the Smyth order (i.e.,,
$P_S(\mathscr{G})$
is the Smyth order (i.e.,,
![]() $\leq _{P_S(\mathscr{G})}=\sqsubseteq$
). When
$\leq _{P_S(\mathscr{G})}=\sqsubseteq$
). When
![]() $\mathscr{G}$
is equipped with the Smyth order, the mappings
$\mathscr{G}$
is equipped with the Smyth order, the mappings
![]() $m_x : \Sigma X \rightarrow P_S(\mathscr{G})$
and
$m_x : \Sigma X \rightarrow P_S(\mathscr{G})$
and
![]() $m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G})$
are defined by
$m : \Sigma (X\times X) \rightarrow P_S(\mathscr{G})$
are defined by
![]() $m_x(y)=\mathord{\uparrow } x\bigvee _{P_S(\mathscr{G})} \mathord{\uparrow } y=\mathord{\uparrow } x\bigvee _{\mathscr{G}} \mathord{\uparrow } y$
and
$m_x(y)=\mathord{\uparrow } x\bigvee _{P_S(\mathscr{G})} \mathord{\uparrow } y=\mathord{\uparrow } x\bigvee _{\mathscr{G}} \mathord{\uparrow } y$
and
![]() $m((u, v))=\mathord{\uparrow } u\bigvee _{P_S(\mathscr{G})} \mathord{\uparrow } v=\mathord{\uparrow } u\bigvee _{\mathscr{G}} \mathord{\uparrow } v$
. The preceding theorem indicates that the notion of a strong
$m((u, v))=\mathord{\uparrow } u\bigvee _{P_S(\mathscr{G})} \mathord{\uparrow } v=\mathord{\uparrow } u\bigvee _{\mathscr{G}} \mathord{\uparrow } v$
. The preceding theorem indicates that the notion of a strong
![]() $d$
-space can be seen as a variant of join continuity.
$d$
-space can be seen as a variant of join continuity.
Corollary 63.
Let
![]() $P$
be a poset and
$P$
be a poset and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $P$
such that
$P$
such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. Then the following conditions are equivalent:
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. Then the following conditions are equivalent:
-
(1)
 $\Sigma P$
is a strong
$\Sigma P$
is a strong
 $d$
-space.
$d$
-space.
-
(2) For any
 $D\in \mathscr{D}(P)$
,
$D\in \mathscr{D}(P)$
,
 $\mathord{\uparrow } F\in \mathbf{Fin} P$
and
$\mathord{\uparrow } F\in \mathbf{Fin} P$
and
 $U\in \sigma (P)$
,
$U\in \sigma (P)$
,
 $\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
implies
$\bigcap _{d\in D}\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
implies
 $\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
for some
$\mathord{\uparrow } d\cap \mathord{\uparrow } F\subseteq U$
for some
 $d\in D$
.
$d\in D$
. -
(3)
 $P$
is a dcpo, and for any
$P$
is a dcpo, and for any
 $A\in \mathscr{C}(\Sigma P)$
and
$A\in \mathscr{C}(\Sigma P)$
and
 $x\in P$
,
$x\in P$
,
 $\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
.
$\mathord{\downarrow } (\mathord{\uparrow } x\cap A)\in \mathscr{C}(\Sigma X)$
. -
(4)
 $P$
is a dcpo, and for any
$P$
is a dcpo, and for any
 $A\in \mathscr{C}(\Sigma P)$
and
$A\in \mathscr{C}(\Sigma P)$
and
 $K\in \mathord{\mathsf{K}} (\Sigma \,\!\!P)$
,
$K\in \mathord{\mathsf{K}} (\Sigma \,\!\!P)$
,
 $\mathord{\downarrow } (K\cap A)\in \mathscr{C}(\Sigma P)$
.
$\mathord{\downarrow } (K\cap A)\in \mathscr{C}(\Sigma P)$
. -
(5)
 $X$
is a dcpo, and for all
$X$
is a dcpo, and for all
 $x\in X$
, the mapping
$x\in X$
, the mapping
 $m_x : \Sigma P \rightarrow P_S(\mathscr{G}), y\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m_x : \Sigma P \rightarrow P_S(\mathscr{G}), y\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
-
(6)
 $P$
is a dcpo, and the mapping
$P$
is a dcpo, and the mapping
 $m : \Sigma (P\times P) \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m : \Sigma (P\times P) \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
For
![]() $\mathscr{G}=\mathbf{up}(P)$
, the equivalences of conditions (1), (3), (4), and (5) in Corollary 63 were also given in Miao et al. (Reference Miao, Yuan and Li2021, Lemma 3.2 and Proposition 3.3).
$\mathscr{G}=\mathbf{up}(P)$
, the equivalences of conditions (1), (3), (4), and (5) in Corollary 63 were also given in Miao et al. (Reference Miao, Yuan and Li2021, Lemma 3.2 and Proposition 3.3).
The following result follows directly from Proposition 26 and Corollary 63(4).
Corollary 64.
For a dcpo
![]() $P$
, if
$P$
, if
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space, then it is well-filtered.
$d$
-space, then it is well-filtered.
Remark 65. Note that Corollary 64, together with Lemma 50(3), is an improvement on Theorem 43 .
Corollary 66.
Let
![]() $P$
be a dcpo. If
$P$
be a dcpo. If
![]() $\Sigma P$
is core-compact (especially, locally compact) and a strong
$\Sigma P$
is core-compact (especially, locally compact) and a strong
![]() $d$
-space, then
$d$
-space, then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
Proof. By Theorem29 and Corollary 64.
Corollary 67.
(Miao et al. Reference Miao, Yuan and Li2021
, Corollary 3.6) Let
![]() $P$
be a Scott-compact poset such that
$P$
be a Scott-compact poset such that
![]() $\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is Scott-compact for all
$\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is Scott-compact for all
![]() $x, y\in P$
(in particular,
$x, y\in P$
(in particular,
![]() $\Sigma P$
is coherent). Then the following three conditions are equivalent:
$\Sigma P$
is coherent). Then the following three conditions are equivalent:
-
(1)
 $\Sigma P$
is an R-space.
$\Sigma P$
is an R-space.
-
(2)
 $\Sigma P$
is a strong
$\Sigma P$
is a strong
 $d$
-space.
$d$
-space.
-
(3)
 $\Sigma P$
is well-filtered.
$\Sigma P$
is well-filtered.
Proof. By Lemma 50(3), (1) implies (2), and (2) implies (3) by Corollary 64. The implication (3) implies (1) follows from Lemma 25, Proposition 33, and the equivalence of property R and
![]() $\Omega ^{\star }$
-compactness (Theorem35).
$\Omega ^{\star }$
-compactness (Theorem35).
By Theorem44 and Corollary 67, we get the following corollary.
Corollary 68.
For a dcpo
![]() $P$
, the following two conditions are equivalent:
$P$
, the following two conditions are equivalent:
-
(1)
 $(P,\lambda (P))$
is compact.
$(P,\lambda (P))$
is compact.
-
(2)
 $\Sigma P$
is a compact strong
$\Sigma P$
is a compact strong
 $d$
-space and
$d$
-space and
 $\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is Scott-compact for all
$\mathord{\uparrow } x\cap \mathord{\uparrow } y$
is Scott-compact for all
 $x, y\in P$
.
$x, y\in P$
.
In Xu et al. (Reference Xu, Shen, Xi and Zhao2020a), it was proved that every first-countable well-filtered space is sober (see Xu et al. Reference Xu, Shen, Xi and Zhao2020a, Theorem 4.2). By this result and Corollary 64, we deduce the following result.
Proposition 69.
Let
![]() $P$
be a dcpo for which
$P$
be a dcpo for which
![]() $\Sigma P$
is first-countable and a strong
$\Sigma P$
is first-countable and a strong
![]() $d$
-space (especially, an R-space). Then
$d$
-space (especially, an R-space). Then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
For a
![]() $T_0$
-space
$T_0$
-space
![]() $X$
and
$X$
and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
such that
$X$
such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
, there is naturally another mapping from
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
, there is naturally another mapping from
![]() $X\times X$
to
$X\times X$
to
![]() $\mathscr{G}$
, more precisely, the mapping
$\mathscr{G}$
, more precisely, the mapping
![]() $m^{\star } : X\times X \rightarrow P_S(\mathscr{G})$
defined by
$m^{\star } : X\times X \rightarrow P_S(\mathscr{G})$
defined by
![]() $m^{\star }((x, y))=\mathord{\uparrow } x\cap \mathord{\uparrow } y$
for all
$m^{\star }((x, y))=\mathord{\uparrow } x\cap \mathord{\uparrow } y$
for all
![]() $(x, y)\in X\times X$
. In this way, for
$(x, y)\in X\times X$
. In this way, for
![]() $x\in X$
, one can define a mapping
$x\in X$
, one can define a mapping
![]() $m^{\star }_x : X \rightarrow P_S(\mathscr{G})$
by
$m^{\star }_x : X \rightarrow P_S(\mathscr{G})$
by
![]() $m^{\star }_x(y)=\mathord{\uparrow } x\cap \mathord{\uparrow } y$
for all
$m^{\star }_x(y)=\mathord{\uparrow } x\cap \mathord{\uparrow } y$
for all
![]() $y\in X$
. It is easy to verify that the continuity of
$y\in X$
. It is easy to verify that the continuity of
![]() $m^{\star }$
implies the continuity of
$m^{\star }$
implies the continuity of
![]() $m^{\star }_x$
for all
$m^{\star }_x$
for all
![]() $x\in X$
. The following example shows that the converse fails in general (comparing it with the equivalence of conditions (3) and (4) in Proposition 60). It also shows that even for a strong
$x\in X$
. The following example shows that the converse fails in general (comparing it with the equivalence of conditions (3) and (4) in Proposition 60). It also shows that even for a strong
![]() $d$
-space
$d$
-space
![]() $X$
, the mapping
$X$
, the mapping
![]() $m^{\star } : X\times X \rightarrow P_S(\mathscr{G})$
may not be continuous.
$m^{\star } : X\times X \rightarrow P_S(\mathscr{G})$
may not be continuous.
Example 70.
Let
![]() $X$
be a countably infinite set and
$X$
be a countably infinite set and
![]() $X_{cof}$
the space equipped with the co-finite topology (see Example 54) and
$X_{cof}$
the space equipped with the co-finite topology (see Example 54) and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
such that
$X$
such that
![]() $\mathscr{G}\supseteq \mathcal \{\{x\} : x\in X\}$
. Then
$\mathscr{G}\supseteq \mathcal \{\{x\} : x\in X\}$
. Then
-
(a)
 $X_{cof}$
is a locally compact
$X_{cof}$
is a locally compact
 $T_1$
-space.
$T_1$
-space.
-
(b)
 $X_{cof}$
is a strong
$X_{cof}$
is a strong
 $d$
-space but not well-filtered.
$d$
-space but not well-filtered.
-
(c) For each
 $x\in X$
,
$x\in X$
,
 $m^{\star }_x : X_{cof} \rightarrow P_S(\mathscr{G}), y\mapsto \{x\}\cap \{y\}$
, is continuous. For
$m^{\star }_x : X_{cof} \rightarrow P_S(\mathscr{G}), y\mapsto \{x\}\cap \{y\}$
, is continuous. For
 $y\in X$
, we have
Therefore, for any
$y\in X$
, we have
Therefore, for any \begin{equation*}m^{\star }_x(y)= \begin {cases} \emptyset, & y\neq x\\ \{x\},& y=x. \end {cases}\end{equation*}
\begin{equation*}m^{\star }_x(y)= \begin {cases} \emptyset, & y\neq x\\ \{x\},& y=x. \end {cases}\end{equation*}
 $U\in \mathscr{O}(X_{cof})$
,So
$U\in \mathscr{O}(X_{cof})$
,So \begin{equation*}(m^{\star }_x)^{-1}(U)= \begin {cases} X,& x\in U\\ X\setminus \{x\},& x\not \in U. \end {cases}\end{equation*}
\begin{equation*}(m^{\star }_x)^{-1}(U)= \begin {cases} X,& x\in U\\ X\setminus \{x\},& x\not \in U. \end {cases}\end{equation*}
 $m^{\star }_x : X_{cof} \rightarrow P_S(\mathscr{G})$
is continuous.
$m^{\star }_x : X_{cof} \rightarrow P_S(\mathscr{G})$
is continuous.
-
(d)
 $m^{\star } : X_{cof}\times X_{cof} \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \{x\}\cap \{y\}$
, is not continuous. Assume, on the contrary, that
$m^{\star } : X_{cof}\times X_{cof} \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \{x\}\cap \{y\}$
, is not continuous. Assume, on the contrary, that
 $m^{\star }$
is continuous. Then
$m^{\star }$
is continuous. Then
 $(m^{\star })^{-1}(\Box _{\mathscr{G}}\emptyset )=\{(x, y)\in X\times X : \{x\}\cap \{y\}=\emptyset \}=X\times X\setminus \{(x, x) : x\in X\}\in \mathscr{O}(X_{cof}\times X_{cof})$
. Hence, for two different point
$(m^{\star })^{-1}(\Box _{\mathscr{G}}\emptyset )=\{(x, y)\in X\times X : \{x\}\cap \{y\}=\emptyset \}=X\times X\setminus \{(x, x) : x\in X\}\in \mathscr{O}(X_{cof}\times X_{cof})$
. Hence, for two different point
 $s, t\in X$
(i.e.,
$s, t\in X$
(i.e.,
 $s\neq t$
), there are
$s\neq t$
), there are
 $F, G\in X^{(\lt \omega )}$
such that
$F, G\in X^{(\lt \omega )}$
such that
 $(s, t)\in (X\setminus F)\times (X\setminus G)\subseteq (m^{\star })^{-1}(\Box _{\mathscr{G}}\emptyset )$
. Select a point
$(s, t)\in (X\setminus F)\times (X\setminus G)\subseteq (m^{\star })^{-1}(\Box _{\mathscr{G}}\emptyset )$
. Select a point
 $u\in X\setminus (F\cup G)$
. Then
$u\in X\setminus (F\cup G)$
. Then
 $(u, u)\in (X\setminus F)\times (X\setminus G)\subseteq X\times X\setminus \{(x, x) : x\in X\}$
, a contradiction. Thus,
$(u, u)\in (X\setminus F)\times (X\setminus G)\subseteq X\times X\setminus \{(x, x) : x\in X\}$
, a contradiction. Thus,
 $m^{\star } : X_{cof}\times X_{cof} \rightarrow P_S(\mathscr{G})$
is not continuous.
$m^{\star } : X_{cof}\times X_{cof} \rightarrow P_S(\mathscr{G})$
is not continuous.
As an immediate corollary of Theorem61 (or Corollary 63), we get the following.
Proposition 71.
Let
![]() $P$
be a poset for which
$P$
be a poset for which
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space and
$d$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $P$
such that
$P$
such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If
![]() $\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then the mapping
$\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then the mapping
![]() $m^{\star } : \Sigma P\times \Sigma P \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
$m^{\star } : \Sigma P\times \Sigma P \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous.
From Theorem17 and Proposition 71, we deduce the following.
Corollary 72.
Let
![]() $P$
be a poset for which
$P$
be a poset for which
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space and
$d$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $P$
such that
$P$
such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If
![]() $\Sigma P$
is core-compact (especially, locally compact), then the mapping
$\Sigma P$
is core-compact (especially, locally compact), then the mapping
![]() $m^{\star } : \Sigma P\times \Sigma P \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
is continuous.
$m^{\star } : \Sigma P\times \Sigma P \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
is continuous.
Finally, we give some conditions on a
![]() $d$
-space
$d$
-space
![]() $X$
under which
$X$
under which
![]() $X$
is sober. In particular, we show that for a dcpo
$X$
is sober. In particular, we show that for a dcpo
![]() $P$
, if
$P$
, if
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space and
$d$
-space and
![]() $\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then
$\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
Proposition 73.
Let
![]() $X$
be a
$X$
be a
![]() $d$
-space and
$d$
-space and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
such that
$X$
such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If the mapping
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If the mapping
![]() $m^{\star } : X\times X \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
is continuous, then
$m^{\star } : X\times X \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
is continuous, then
![]() $X$
is sober.
$X$
is sober.
Proof. Since
![]() $X$
is a
$X$
is a
![]() $d$
-space,
$d$
-space,
![]() $X$
(with the specialization order) is a dcpo and
$X$
(with the specialization order) is a dcpo and
![]() $\mathscr{C}(X)\subseteq \mathscr{C}(\Sigma X)$
. Suppose that
$\mathscr{C}(X)\subseteq \mathscr{C}(\Sigma X)$
. Suppose that
![]() $A\in{\mathsf{Irr}}_c(X)$
. We show that
$A\in{\mathsf{Irr}}_c(X)$
. We show that
![]() $A$
is directed. Assume, on the contrary, that
$A$
is directed. Assume, on the contrary, that
![]() $A$
is not directed. Then there exist
$A$
is not directed. Then there exist
![]() $b, c\in A$
such that
$b, c\in A$
such that
![]() $\mathord{\uparrow } b\cap \mathord{\uparrow } c\cap A=\emptyset$
or, equivalently,
$\mathord{\uparrow } b\cap \mathord{\uparrow } c\cap A=\emptyset$
or, equivalently,
![]() $(b, c)\in (m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))$
. As
$(b, c)\in (m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))$
. As
![]() $m^{\star } : X\times X \rightarrow P_S(\mathscr{G})$
is continuous,
$m^{\star } : X\times X \rightarrow P_S(\mathscr{G})$
is continuous,
![]() $(m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))\in \mathscr{O}(X\times X)$
. Hence, there exist
$(m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))\in \mathscr{O}(X\times X)$
. Hence, there exist
![]() $V, W\in \mathscr{O}(X)$
such that
$V, W\in \mathscr{O}(X)$
such that
![]() $(b,c)\in V\times W\subseteq (m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))$
. As
$(b,c)\in V\times W\subseteq (m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))$
. As
![]() $A\in{\mathsf{Irr}}_c(X$
,
$A\in{\mathsf{Irr}}_c(X$
,
![]() $A\cap V\neq \emptyset$
and
$A\cap V\neq \emptyset$
and
![]() $A\cap W\neq \emptyset$
, we have that
$A\cap W\neq \emptyset$
, we have that
![]() $A\cap V\cap W\neq \emptyset$
. Select a
$A\cap V\cap W\neq \emptyset$
. Select a
![]() $z\in A\cap V\cap W$
. Then
$z\in A\cap V\cap W$
. Then
![]() $m^{\star }(z,z)=\mathord{\uparrow } z\cap \mathord{\uparrow } z=\mathord{\uparrow } z\in (m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))$
, that is
$m^{\star }(z,z)=\mathord{\uparrow } z\cap \mathord{\uparrow } z=\mathord{\uparrow } z\in (m^{\star })^{-1}(\Box _{\mathscr{G}}(X\setminus A))$
, that is
![]() $\mathord{\uparrow } z\subseteq X\setminus A$
, a contradiction. So
$\mathord{\uparrow } z\subseteq X\setminus A$
, a contradiction. So
![]() $A$
is directed and hence
$A$
is directed and hence
![]() $\bigvee A\in A$
, and consequently,
$\bigvee A\in A$
, and consequently,
![]() $A=\mathord{\downarrow } \bigvee A={\textrm{cl}}_{X}\{\bigvee A\}$
. Thus,
$A=\mathord{\downarrow } \bigvee A={\textrm{cl}}_{X}\{\bigvee A\}$
. Thus,
![]() $X$
is sober.
$X$
is sober.
Corollary 74.
Let
![]() $P$
be a dcpo and
$P$
be a dcpo and
![]() $\mathscr{G}$
a family of subsets of
$\mathscr{G}$
a family of subsets of
![]() $X$
such that
$X$
such that
![]() $\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If the mapping
$\mathscr{G}\supseteq \mathscr{S}^u_2=\{\mathord{\uparrow } x\cap \mathord{\uparrow } y : x, y\in P\}$
. If the mapping
![]() $m^{\star } : \Sigma P\times \Sigma P \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous, then
$m^{\star } : \Sigma P\times \Sigma P \rightarrow P_S(\mathscr{G}), (x, y)\mapsto \mathord{\uparrow } x\cap \mathord{\uparrow } y$
, is continuous, then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
From Theorem61 and Proposition 73, we deduce the following.
Proposition 75.
If
![]() $X$
is a strong
$X$
is a strong
![]() $d$
-space and
$d$
-space and
![]() $X\times X=\Sigma (X\times X)$
, then
$X\times X=\Sigma (X\times X)$
, then
![]() $X$
is sober.
$X$
is sober.
As an immediate corollary of Proposition 75, we get the following important result.
Theorem 76.
If
![]() $P$
is a dcpo such that
$P$
is a dcpo such that
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space and
$d$
-space and
![]() $\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then
$\Sigma (P\times P)=\Sigma P\times \Sigma P$
, then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
Corollary 77.
Let
![]() $P$
be a dcpo for which
$P$
be a dcpo for which
![]() $\Sigma (P\times P)=\Sigma P\times \Sigma P$
. If
$\Sigma (P\times P)=\Sigma P\times \Sigma P$
. If
![]() $P$
satisfies one of the following conditions:
$P$
satisfies one of the following conditions:
-
(1)
 $P$
is a complete lattice.
$P$
is a complete lattice.
-
(2)
 $(P, \lambda (P))$
is compact.
$(P, \lambda (P))$
is compact.
-
(3)
 $(P, \lambda (P))$
is upper-semicompact.
$(P, \lambda (P))$
is upper-semicompact.
-
(4)
 $\Sigma P$
is well-filtered and coherent.
$\Sigma P$
is well-filtered and coherent.
-
(5)
 $\Sigma P$
is an R-space.
$\Sigma P$
is an R-space.
Then
![]() $\Sigma P$
is sober.
$\Sigma P$
is sober.
Proof. By Lemma 6, Propositions 42 and 33(2), we have that (1)
![]() $\Rightarrow$
(2)
$\Rightarrow$
(2)
![]() $\Rightarrow$
(3)
$\Rightarrow$
(3)
![]() $\Rightarrow$
(5) and (4)
$\Rightarrow$
(5) and (4)
![]() $\Rightarrow$
(5). By Lemma 50(3), (5) implies that
$\Rightarrow$
(5). By Lemma 50(3), (5) implies that
![]() $\Sigma P$
is a strong
$\Sigma P$
is a strong
![]() $d$
-space. Therefore, we get Corollary 77 by Theorem76.
$d$
-space. Therefore, we get Corollary 77 by Theorem76.
Remark 78.
If
![]() $P$
is a dcpo such that
$P$
is a dcpo such that
![]() $\Sigma P$
is a first-countable strong
$\Sigma P$
is a first-countable strong
![]() $d$
-space, then by Theorem 4.2 of Xu et al. (Reference Xu, Shen, Xi and Zhao2020a) and Corollary 64 we also get the sobriety of
$d$
-space, then by Theorem 4.2 of Xu et al. (Reference Xu, Shen, Xi and Zhao2020a) and Corollary 64 we also get the sobriety of
![]() $\Sigma P$
.
$\Sigma P$
.
Acknowledgements
The authors would like to thank the referee for the numerous and very helpful suggestions that have improved this paper substantially.
Competing interest
The authors declare that they have no conflicts of interest to this work. We declare that we do not have any commercial or associative interest that represents a conflict of interest in connection with the work entitled “T0-spaces and the lower topology” (Manuscript ID: MSCS-2023-087 ).