Hostname: page-component-6bf8c574d5-gr6zb Total loading time: 0 Render date: 2025-02-22T21:48:18.825Z Has data issue: false hasContentIssue false
  • English
  • Français

Rewriting systems for the surface classification theorem

Published online by Cambridge University Press:  27 May 2010

GABRIELE PULCINI
GABRIELE PULCINI*
Affiliation:
Département d'Informatique, École Normale Supérieure, Paris, 45, rue d'Ulm – F-75230 Paris Cedex 05 – France Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The work reported in this paper refers to Massey's proof of the surface classification theorem based on the standard word-rewriting treatment of surfaces. We arrange this approach into a formal rewriting system 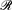and provide a new version of Massey's argument. Moreover, we study the computational properties of two subsystems of 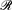: 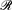or for dealing with words denoting orientable surfaces and 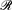nor for dealing with words denoting non-orientable surfaces. We show how such properties induce an alternative proof for the surface classification in which the basic homeomorphism between the connected sum of three projective planes and the connected sum of a torus with a projective plane is not required.

Type
Paper
Information
Mathematical Structures in Computer Science , Volume 20 , Issue 4 , August 2010 , pp. 577 - 588
Copyright
Copyright © Cambridge University Press 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andreoli, J.-M., Pulcini, G. and Ruet, P. (2005) Permutative Logic. In: Ong, L. (ed.) Proceedings, Computer Science Logic 19th International Workshop, CSL 2005. Springer-Verlag Lecture Notes in Computer Science 3634 184–199.CrossRefGoogle Scholar
Gaubert, C. (2004) Two-dimensional proof-structures and the exchange rule. Mathematical Structures in Computer Science 14 (1)73–96.CrossRefGoogle Scholar
Girard, J.-Y. (1989) Proofs and Types, Cambridge Tracts in Theoretical Computer Science 7.Google Scholar
Girard, J.-Y. (1995) Linear Logic: its syntax and semantics. Advances in Linear Logic. London Mathematical Society Lecture Note Series 222, Cambridge University Press 1–42.Google Scholar
Girard, J.-Y. (2001) Locus Solum. Mathematical Structures in Computer Science 11 301–506.CrossRefGoogle Scholar
Massey, W. S. (1991) A basic course in algebraic topology, Springerr-Verlag.CrossRefGoogle Scholar
Melliès, P.-A. (2004) A topological correctness criterion for multiplicative non-commutative logic. In: Linear logic in computer science. London Mathematical Society Lecture Notes Series 316, Cambridge University Press.Google Scholar
Métayer, F. (2001) Implicit exchange in multiplicative proofnets. Mathematical Structures in Computer Science 11 (2)261–272.CrossRefGoogle Scholar
Pulcini, G. (2009) Computing Surfaces via pq-Permutations. International Journal of Imaging Systems and Technology 19 (2)132–139.CrossRefGoogle Scholar