Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-29T07:52:16.695Z Has data issue: false hasContentIssue false
  • English
  • Français

Quantum circuits generating four-qubit maximally entangled states

Published online by Cambridge University Press:  28 September 2022

Marc Bataille Open the ORCID record for Marc Bataille [Opens in a new window]
Marc Bataille*
Affiliation:
LITIS Laboratory, Université Rouen-Normandie, 685 Avenue de l’Université, 76800 Saint-Étienne-du-Rouvray, France
*
Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We describe quantum circuits generating four-qubit maximally entangled states, the amount of entanglement being quantified by using the absolute value of the Cayley hyperdeterminant as an entanglement monotone. More precisely we show that this type of four-qubit entangled states can be obtained by the action of a family of 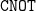$\mathtt{CNOT}$ circuits on some special states of the LU orbit of the state 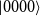$|0000\rangle$ .

Keywords

quantum informationquantum circuitsentanglementhyperdeterminant
Type
Paper
Information
Mathematical Structures in Computer Science , Volume 32 , Issue 3 , March 2022 , pp. 257 - 270
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Alsina, D. (2017). Multipartite Entanglement and Quantum Algorithms. Phd thesis, Universitat de Barcelona. arXiv:1706.08318.Google Scholar
Bataille, M. (2022). Quantum circuits of CNOT gates: optimization and entanglement. Quantum Information Processing 21. https://doi.org/10.1007/s11128-022-03577-8.Google Scholar
Chen, L. and Djokovic, D. Z. (2013). Proof of the Gour-Wallach conjecture. Physical Review A 88 (4) 2–4.CrossRefGoogle Scholar
Gelfand, I. M., Kapranov, M. M. and Zelevisnky, A. V. (1992). Discriminants, Resultants and Multidimensional Determinant, Birkhäuser, Boston, MA.Google Scholar
Gour, G. and Wallach, N. R. (2010). All maximally entangled four-qubit states. Journal of Mathematical Physics 51 (11) 112201.CrossRefGoogle Scholar
Gour, G. and Wallach, N. R. (2012). On symmetric SL-invariant polynomials in four qubits. arXiv:1211.5586.Google Scholar
Huggins, P., Sturmfels, B., Yu, J. and Yuster, D. S. (2008). The hyperdeterminant and triangulations of the 4-cube. Mathematics of Computation 77 (263) 1653–1679.Google Scholar
Jaffali, H. (2020). Étude de l’Intrication dans les Algorithmes Quantiques: Approche Géométrique et Outils Dérivés. Phd thesis, Université Bourgogne Franche-Comté.Google Scholar
Jaffali, H. and Oeding, L. (2020). Learning algebraic models of quantum entanglement. Quantum Information Processing 19 (9) 12–14.CrossRefGoogle Scholar
Luque, J., Thibon, J. and Toumazet, F. (2007). Unitary invariants of qubit systems. Mathematical Structures in Computer Science 17 1133–1151.CrossRefGoogle Scholar
Luque, J.-G. and Thibon, J.-Y. (2003). The polynomial invariants of four qubits. Physical Review A 67 042303.Google Scholar
Miyake, A. (2003). Classification of multipartite entangled states by multidimensional determinant. Physical Review A 67 012108.CrossRefGoogle Scholar
Miyake, A. and Wadati, M. (2002). Multipartite entanglement and hyperdeterminants. Quantum Information and Computation 2 540–555.Google Scholar
Nielsen, M. A. and Chuang, I. L. (2011). Quantum Computation and Quantum Information: 10th Anniversary Edition, 10th ed., Cambridge University Press, New York, NY, USA.Google Scholar
Osterloh, A. and Siewert, J. (2006). Entanglement monotones and maximally entangled states in multipartite qubit systems. International Journal of Quantum Information 04 (03) 531–540.Google Scholar