Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-28T15:14:21.387Z Has data issue: false hasContentIssue false
  • English
  • Français

A linear category of polynomial diagrams

Published online by Cambridge University Press:  17 May 2013

PIERRE HYVERNAT
PIERRE HYVERNAT*
Affiliation:
Laboratoire de Mathématiques, CNRS UMR 5126 – Université de Savoie, 73376 Le Bourget-du-Lac Cedex, France Email: [email protected] Website: http://lama.univ-savoie.fr/~hyvernat/
Get access Rights & Permissions [Opens in a new window]

Abstract

We present a categorical model for intuitionistic linear logic in which objects are polynomial diagrams and morphisms are simulation diagrams. The multiplicative structure (tensor product and its adjoint) can be defined in any locally cartesian closed category, but the additive (product and coproduct) and exponential (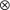-comonoid comonad) structures require additional properties and are only developed in the category Set, where the objects and morphisms have natural interpretations in terms of games, simulation and strategies.

Type
Paper
Information
Mathematical Structures in Computer Science , Volume 24 , Issue 1 , February 2014 , e240104
Copyright
Copyright © Cambridge University Press 2013 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abott, M., Altenkirch, T. and Ghani, N. (2005) Containers – constructing strictly positive types. Theoretical Computer Science 342 3–27.Google Scholar
Blute, R. F., Cockett, J. R. B. and Seely, R. A. G. (2006) Differential categories. Mathematical Structures in Computer Science 16 1049–1083.Google Scholar
Ehrhard, T. and Regnier, L. (2003) The differential lambda calculus. Theoretical Computer Science 309 (1)1–41.Google Scholar
Ehrhard, T. and Regnier, L. (2006) Differential interaction nets. Theoretical Computer Science 364 166–195.Google Scholar
Gambino, N. and Kock, J. (2013) Polynomial functors and polynomial monads. Mathematical Proceedings of the Cambridge Philosophical Society 154 (1)153–192.Google Scholar
Hancock, P. and Hyvernat, P. (2006) Programming interfaces and basic topology. Annals of Pure and Applied Logic 137 (1–3)189–239.Google Scholar
Hofmann, M. (1995) On the interpretation of type theory in locally cartesian closed categories. In: Pacholski, L. and Tiuryn, J. (eds.) Computer Science Logic: Selected Papers, 8th Workshop, CSL ‘94. Springer-Verlag Lecture Notes in Computer Science 933 427–441.CrossRefGoogle Scholar
Hyvernat, P. (2005) A Logical Investigation of Interaction Systems, Thèse de doctorat, Institut mathématique de Luminy, Université Aix-Marseille II.Google Scholar
Hyvernat, P. (2012) A linear category of polynomial functors (extensional part). In preparation.CrossRefGoogle Scholar
Joyal, A. (1977) Remarques sur la théorie des jeux à deux personnes. Gazette des sciences mathématiques du Quebec 1 (4)175.Google Scholar
Kock, J. (2009) Notes on polynomial functors. Preliminary draft.CrossRefGoogle Scholar
Martin-Löf, P. (1984) Intuitionistic type theory, Bibliopolis. (Notes by Giovanni Sambin.)Google Scholar
Morris, P. and Altenkirch, T. (2009) Indexed containers. In: Proceedings of the 24th Annual IEEE Symposium on Logic in Computer Science, LICS 2009, IEEE Computer Society 277–285.Google Scholar
Seely, R. A. G. (1984) Locally cartesian closed categories and type theory. Mathematical Proceedings of the Cambridge Philosophical Society 95 (1)33–48.Google Scholar