Research Article
Finite equilibrated groups
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 579-588
-
- Article
- Export citation
Uniqueness theorems for left-symmetric enveloping algebras
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 193-206
-
- Article
- Export citation
An algebraic condition to determine whether a knot is prime
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 385-389
-
- Article
- Export citation
On a class of aperiodic sum-free sets
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 01-05
-
- Article
- Export citation
Ramanujan's formula for the logarithmic derivative of the gamma function
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 391-401
-
- Article
- Export citation
Countable partitions of Euclidean space
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 07-12
-
- Article
- Export citation
Decomposing the complexity quotient category
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 589-595
-
- Article
- Export citation
Path homomorphisms
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 207-220
-
- Article
- Export citation
Complexity and varieties for infinitely generated modules, II
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 597-615
-
- Article
- Export citation
On the size of products of distances from prescribed points
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 403-409
-
- Article
- Export citation
Transfer maps for fibrations
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 221-235
-
- Article
- Export citation
The elliptic Mahler measure
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 13-25
-
- Article
- Export citation
Nilpotent semigroups of matrices
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 617-630
-
- Article
- Export citation
Representation of an element in SL(2,
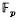 ,) as a short word in two generators
,) as a short word in two generators
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 27-29
-
- Article
- Export citation
On the complement of a nef and big divisor on an algebraic variety
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 411-422
-
- Article
- Export citation
On braid monodromies of non-simple braided surfaces
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 237-245
-
- Article
- Export citation
The mod p cohomology group of extra-special p-group of order p5 and of exponent p2
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 423-440
-
- Article
- Export citation
On the lengths of Koszul homology modules and generalized fractions
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 31-42
-
- Article
- Export citation
Configurations of 2-spheres in the K3 surface and other 4-manifolds
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 247-253
-
- Article
- Export citation
A distance formula and Bourgain algebras
-
- Published online by Cambridge University Press:
- 24 October 2008, pp. 631-641
-
- Article
- Export citation

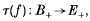
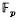 ,) as a short word in two generators
,) as a short word in two generators
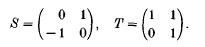
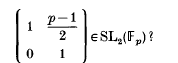
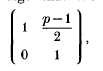
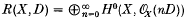 . As is known, for a semi-ample divisor
. As is known, for a semi-ample divisor 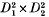 such that
such that 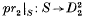 is a branched covering map of degree
is a branched covering map of degree 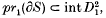 , where
, where 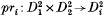 is the projection. It was defined L. Rudolph [
is the projection. It was defined L. Rudolph [ and
and 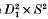 is the tubular neighbourhood of a standard 2-sphere in
is the tubular neighbourhood of a standard 2-sphere in 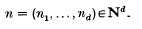

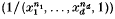 in
in 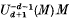 a polynomial in
a polynomial in