Hostname: page-component-586b7cd67f-dsjbd Total loading time: 0 Render date: 2024-11-29T23:15:25.513Z Has data issue: false hasContentIssue false
  • English
  • Français

Unstable Adams operations on classifying spaces

Published online by Cambridge University Press:  24 October 2008

Kenshi Ishiguro
Kenshi Ishiguro
Affiliation:
Department of Mathematics, University of Chicago, Chicago, IL 60637, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Extract

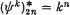The Adams operations {ψk} considered as self-maps of BU have the property that . Id2n on H2n(BU; ℚ). Sullivan in his M.I.T. notes [12] constructed ψk-type self-maps of BU(n) in the case in which k is prime to n!. Later work by Friedlander[5] and Wilkerson[14] gave constructions for all other compact connected Lie groups G, subject to the condition that k be prime to the order of the Weyl group W(G).

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 102 , Issue 1 , July 1987 , pp. 71 - 75
Copyright
Copyright © Cambridge Philosophical Society 1987

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Adams, J. F.. Lectures on Lie Groups (Benjamin, 1969).Google Scholar
[2] Adams, J. F. and Mahmud, Z.. Maps between classifying spaces. Invent. Math. 35 (1976), 1–41.Google Scholar
[3] Brown, K. S.. Cohomology of Groups (Springer-Verlag, 1982).CrossRefGoogle Scholar
[4] Dwyer, W. G.. Maps between classifying spaces. Preprint.Google Scholar
[5] Friedlander, E. M.. Exceptional isogenies and the classifying spaces of simple Lie groups. Ann. of Math. 101 (1975), 510–520.Google Scholar
[6] Hubbuck, J. R.. Homotopy-homomorphisms of Lie groups. In New Developments in Topology, L.M.S. Lecture Notes series, No. 11 (Cambridge University Press, 1974), 33–41.CrossRefGoogle Scholar
[7] Hubbuck, J. R.. Mapping degrees for classifying spaces, I. Quart. J. Math. Oxford Ser. (2), 25 (1974), 113–133.Google Scholar
[8] Ishiguro, K.. Classifying spaces and p-local irreducibility. To appear in J. of Pure and Applied Alg.Google Scholar
[9] Miller, H. R. and Wilkerson, C. W.. Maps of elementary p-groups into compact Lie groups. Talk at 1985Yale Conference on Algebraic Topology.Google Scholar
[10] Mislin, G.. The homotopy classification of self-maps of infinite quaternionic projective space. Preprint.Google Scholar
[11] Serre, J. P.. Linear Representation of Finite Groups (Springer-Verlag, 1977).CrossRefGoogle Scholar
[12] Sullivan, D.. Localization, Periodicity, and Galois Symmetry. Geometric Topology I Notes, M.I.T. 1970, revised 1971.Google Scholar
[13] Tits, J.. Sur les constantes de structure et le théorème d'existence de algèbres de Lie semisimples. Inst. Hautes Etudes Sci. Publ. Math. 31 (1966), 21–58.CrossRefGoogle Scholar
[14] Wilkerson, C. W.. Self-maps of classifying spaces. In Localization in Group Theory and Homotopy Theory, Lecture Notes in Math., vol. 418 (Springer-Verlag, 1974), 150–157.CrossRefGoogle Scholar
[15] Wilkerson, C. W.. Classifying spaces, Steenrod operations and algebraic closure. Topology 16 (1977), 227–237.CrossRefGoogle Scholar