1. It is trivial that

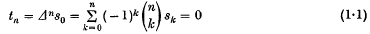
for all integers n ≥ 0 implies sn = 0 (n = 0, 1, 2, …). This conclusion is no longer true if it is only known that (1·1) is true for an infinity of n. But we shall show that the truth of (1·1) for, roughly speaking, one-half of all positive integers, together with an order condition on the magnitude of sn, ensures sn = 0 for all n. This follows from

Theorem 1. Let a1 < a2 < … be positive integers, n(R) the number of a's not exceeding R, sn a sequence of complex numbers. If

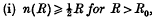
(ii) sn = ο(nK)as n tends to infinity

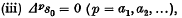
then sn = P(n), where P(x) is a polynomial of degree less than K.