Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-26T13:24:35.179Z Has data issue: false hasContentIssue false
  • English
  • Français

A Tauberian theorem for absolute summability

Published online by Cambridge University Press:  24 October 2008

G. Das
G. Das
Affiliation:
University College, London
Get access Rights & Permissions [Opens in a new window]

Extract

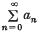Let be the given infinite series with {sn} as the sequence of partial sums and let be the binomial coefficient of zn in the power series expansion of the function (l-z)-σ-1 |z| < 1. Now let, for β > – 1,

converge for 0 ≤ x < 1. If fβ(x) → s as x → 1–, then we say that ∑an is summable (Aβ) to s. If, further, f(x) is a function of bounded variation in (0, 1), then ∑an is summable |Aβ| or absolutely summable (Aβ). We write this symbolically as {sn} ∈ |Aβ|. This method was first introduced by Borwein in (l) where he proves that for α > β > -1, (Aα) ⊂ (Aβ). Note that for β = 0, (Aβ) is the same as Abel method (A). Borwein (2) also introduced the (C, α, β) method as follows: Let α + β ╪ −1, −2, … Then the (C, α, β) mean is defined by

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 67 , Issue 2 , March 1970 , pp. 321 - 326
Copyright
Copyright © Cambridge Philosophical Society 1970

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Borwein, D.On a scale of Abel type summability methods. Proc. Cambridge Philos. Soc. 53 (1957), 318–322.CrossRefGoogle Scholar
(2)Borwein, D.Theorems on some methods of summability. Quart. J. Math. Oxford Ser. 9 (1958), 310–314.Google Scholar
(3)Das, G.On some methods of summability. Quart. J. Math. Oxford Ser. 17 (1966), 244–256.CrossRefGoogle Scholar
(4)Flett, T. M.On an extension of absolute summability and some theorems of Littlewood and Paley. Proc. London Math. Soc. (3), 7 (1957), 113–141.CrossRefGoogle Scholar
(5)Flett, T. M.Some generalisations of Tauber's second theorem. Quart. J. Math. Soc. Oxford Ser. 10 (1959), 70–80.Google Scholar
(6)Hardy, G. H.Divergent series (Oxford, 1949)Google Scholar
(7)Hyslop, J. M.A Tauberian theorem for absolute summability. J. London Math. Soc. 12 (1937), 176–180.CrossRefGoogle Scholar
(8)Jakimovski, A.The sequence-to-function analogues to Hausdorff transformations. Bull. Research Council. Israel, 3 (1960), 135–154.Google Scholar
(9)Knopp, K. and Lorentz, G. G.Beiträge zur absoluten Limitierung. Arch. Math. (Basel), 2 (1949), 10–16.CrossRefGoogle Scholar
(10)Misra, B. P.Absolute summability of infinite series on a scale of Abel type summability methods. Proc. Cambridge Philos. Soc. 64 (1968), 377–387.CrossRefGoogle Scholar
(11)Morley, H.A theorem on Hausdorff transformations and its applications to Cesaro and Holder's means. J. London Math. Soc. 25 (1950), 168–173.CrossRefGoogle Scholar