Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-27T08:29:41.087Z Has data issue: false hasContentIssue false
  • English
  • Français

Sublinear discrete-time order-preserving dynamical systems

Published online by Cambridge University Press:  24 October 2008

J. F. Jiang
J. F. Jiang
Affiliation:
Department of Mathematics, University of Science and Technology of China, Hefei, Anhui, China
Get access Rights & Permissions [Opens in a new window]

Abstract

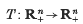Suppose that the continuous mapping is order-preserving and sublinear. If every positive semi-orbit has compact closure, then every positive semi-orbit converges to a fixed point. This result does not require that the order be strongly preserved.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 119 , Issue 3 , April 1996 , pp. 561 - 574
Copyright
Copyright © Cambridge Philosophical Society 1996

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Hirsch, M. W.. Systems of differential equations that are competitive or cooperative. II: Convergence almost everywhere. SIAM J. Math. Anal. 16 (1985), 432–439.CrossRefGoogle Scholar
[2]Hirsch, M. W.. Systems of differential equations that are competitive or cooperative. V: Convergence in 3-dimensional systems. J. Diff. Eqns. 80 (1989), 94–106.CrossRefGoogle Scholar
[3]Hirsch, M. W.. Stability and convergence in strongly monotone dynamical systems. J. reine angew. Math. 383 (1988), 1–53.Google Scholar
[4]Hirsch, M. W.. The dynamical systems approach to differential equations. Bull. Amer. Math. Soc. 11 (1984), 1–64.CrossRefGoogle Scholar
[5]Matano, H.. Strong comparison principle in nonlinear parabolic equations: in Nonlinear parabolic equations: qualitative properties of solutions (Boccardo, L. and Tesei, A., eds.), Pitman Res. Notes in Math. 149 (Longman Scientific and Technical, 1987), 148–155.Google Scholar
[6]Smith, H. L. and Thieme, H. R.. Quasiconvergence and stability for strongly order-preserving semiflows. SIAM J. Math. Anal. 21 (1990), 673–692.CrossRefGoogle Scholar
[7]Smith, H. L. and Thieme, H. R.. Convergence for strongly order-preserving semiflows. SIAM J.Math. Anal. 22 (1991), 1081–1101.CrossRefGoogle Scholar
[8]Smith, H. L.. Cooperative systems of differential equations with concave nonlinearities. J. Nonlinear Anal. 10 (1986), 1037–1052.CrossRefGoogle Scholar
[9]Polaĉik, P.. Convergence in smooth strongly monotone flows defined by semilinear parabolic equations. J. Diff. Eqns. 79 (1989), 89–110.CrossRefGoogle Scholar
[10]Alikakos, N. D. and Hess, P.. On stabilization of discrete monotone dynamical systems. Israel J.Math. 59 (1987), 185–194.CrossRefGoogle Scholar
[11]Alikakos, N. D., Hess, P. and Matano, H.. Discrete order preserving semigroups and stability for periodic parabolic differential equations. J. Diff. Eqns. 82 (1989), 322–341.CrossRefGoogle Scholar
[12]Takáĉ, P.. Convergence to equilibrium on invariant d-hypersurfaces for strongly increasing discrete-time semigroups. J. Math. Anal. Appl. 148 (1990), 223–244.CrossRefGoogle Scholar
[13]Takáĉc, P.. Asymptotic behavior of discrete-time semigroups of sublinear, strongly increasing mappings with applications to biology. J. Nonlinear Anal. 14 (1990). 35–42.CrossRefGoogle Scholar
[14]Dancer, E. N. and Hess, P.. Stability of fixed points for order-preserving discrete-time dynamical systems. J. reine angew. Math. 419 (1991), 125–139.Google Scholar
[15]Jiang, J. F.. Convergence to trap almost everywhere for flows generated by cooperative and irreducible vector fields. Chin. Ann. of Math. Series B 14 (1993), 165–174.Google Scholar
[16]Jiang, J. F.. A Liapunov function for three-dimensional feedback systems. Proc. Amer. Math. Soc. 114 (1992), 1009–1013.CrossRefGoogle Scholar
[17]Jiang, J. F.. A Liapunov function for 4-dimensional positive feedback systems. Quarterly Appl. Math. LII (1994), 601–614.CrossRefGoogle Scholar
[18]Jiang, J. F.. The algebraic criteria for the asymptotic behavior of cooperative systems with concave nonlinearities. J. Systems Sci. Math. Sci. 6 (1993), 193–208.Google Scholar
[19]Jiang, J. F.. A note on a global stability theorem of M. W. Hirsch. Proc. Amer.Math. Soc. 112 (1991), 803–806.CrossRefGoogle Scholar
[20]Jiang, J. F.. On the global stability of cooperative systems. Bull. LondonMath. Soc. 26 (1994), 455–458.Google Scholar
[21]Jiang, J. F.. Three- and four-dimensional cooperative systems with every equilibrium stable. J. Math. Anal. Appl. 188 (1994), 92–100.CrossRefGoogle Scholar
[22]Jiang, J. F.. On the analytic order-preserving discrete-time dynamical systems in Rn with every fixed point stable. To appear in J. LondonMath. Soc.Google Scholar
[23]Coppel, W. A.. Stability and asymptotic behavior of differential equations (Heath, 1965).Google Scholar
[24]Smith, H. L.. Periodic solutions of periodic competitive and cooperative systems. SIAM J. Math. Anal. 17 (1986), 1289–1318.CrossRefGoogle Scholar