Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-28T14:42:16.519Z Has data issue: false hasContentIssue false
  • English
  • Français

Some further results on the birth-and-death process and its integral

Published online by Cambridge University Press:  24 October 2008

Prem S. Puri
Prem S. Puri
Affiliation:
Statistical Laboratory, University of California, Berkeley
Get access Rights & Permissions [Opens in a new window]

Abstract

In a simple homogeneous birth-and-death process with λ and μ as the constant birth and death rates respectively, let X(t) denote the population size at time t, Z(t) the number of deaths and N(t) the number of events (births and deaths combined) occurring during (0, t). Also let 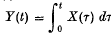. The results obtained include the following:

(a) An explicit formula for the characteristic quasi-probability generating function of the joint distribution of X(t), Y(t) and Z(t).

(b) Let X(0) = 1. It is shown that, if t → ∞ while λ ≤ μ, N(t) ↑ N a.s., where N takes only positive odd integral values. If λ > μ, then P[N(t) ↑ ∞] = 1 − μ/λ. Given that N(t)∞, the limiting distribution of N(t) is similar to that of N. It was reported earlier (Puri (11)), that the limiting distribution of Y(t) is a weighted average of certain chi-square distributions. It is now found that these weights are nothing but the probabilities P[N = 2k + 1] (k = 0, 1,…).

(c) Let λ = μ, and MXω), MYω and MZω be defined as in (36), then as

where the c.f. of (X*; Y*; Z*) is given by (38).

(d) Exact expressions for the p.d.f. of Y(t) are derived for the cases (i) λ = 0, μ > 0, (ii) λ > 0, μ = 0. For the case (iii) λ gt; 0, μ > 0, since the complete expression is complicated, only the procedure of derivation is indicated.

(e) Finally, it is shown that the regressions of Y(t) and of Z(t) on X(t) are linear for X(t) ≥ 1.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 64 , Issue 1 , January 1968 , pp. 141 - 154
Copyright
Copyright © Cambridge Philosophical Society 1968

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Bartlett, M. S.An introduction to stochastic processes with special reference to methods and applications (Cambridge University Press, 1955).Google Scholar
(2)Bartlett, M. S.Equations for stochastic path integrals. Proc. Cambridge Philos. Soc. 57 (1961), 568–573.CrossRefGoogle Scholar
(3)Bartlett, M. S. and Kendall, D. G.On the use of the characteristic functional in the analysis of some stochastic processes occurring in physics and biology. Proc. Cambridge Philos. Soc. 46 (1950), 65–76.Google Scholar
(4)Bellman, R. and Harris, T. E.On the theory of age-dependent stochastic branching processes. Proc. Nat. Acad. Sci. U.S.A. 34 (1948), 601–4.CrossRefGoogle ScholarPubMed
(5)Harris, T. E.The theory of branching processes (Springer-Verlag; Berlin, 1963).CrossRefGoogle Scholar
(6)Kendall, D. G.Stochastic processes and population growth, J. Roy. Statist. Soc. ser. B 11, no. 2 (1949), 230–282.Google Scholar
(7)Kendall, D. G.An artificial realisation of a simple ‘Birth and death’ process. J. Roy. Statist. Soc. supplement 12 (1950), 116–119.Google Scholar
(8)Kendall, D. G.Random fluctuations in the age-distribution of a population whose development is controlled by the simple ‘Birth and Death’ process. J. Roy. Statist. Soc. supplement 12 (1950), 278–285.Google Scholar
(9)Kendall, M. G. and Stuart, A.The advanced theory of statistics, vol I (Hafner Publishing Co.; New York, 1958).Google Scholar
(10)Orey, Steven. Strong ratio limit property. Bull. Amer. Math. Soc. (1961), 571–74.CrossRefGoogle Scholar
(11)Puri, Prem S.On the homogeneous birth-and-death process and its integral. Biometrika 53 (1966), 61–71.CrossRefGoogle ScholarPubMed
(12)Sevast'yanov, B. A.The theory of branching processes. Uspehi Mat. Nauk 6 (1951), 47–49 [Russian].Google Scholar