Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-08T14:06:53.116Z Has data issue: false hasContentIssue false
  • English
  • Français

Simultaneous rational approximations and related diophantine equations

Published online by Cambridge University Press:  24 October 2008

John H. Rickert
John H. Rickert
Affiliation:
The Rose-Hulman Institute of Technology, Terre Haute, Indiana 47803, U.S.A.
Get access Rights & Permissions [Opens in a new window]

Abstract

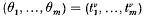In this paper we shall consider simultaneous approximations to algebraic numbers of the form for rational t1, …, tm, v. If t1, …, tm, are sufficiently close to 1 with respect to a common denominator we shall derive an effective inequality of the form max {|θ1 – p1 / q|, …, |θm – pm / q|} > cq–1–λ for all integers p1, …, pm, q with q > 0. The technique is used to derive the inequality

We use this to show that the only solution to the simultaneous equations

are the trivial ones.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 113 , Issue 3 , May 1993 , pp. 461 - 472
Copyright
Copyright © Cambridge Philosophical Society 1993

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baker, A.. Rational approximations to certain algebraic numbers. Proc. London Math. Soc. (3) 14 (1964), 385–398.CrossRefGoogle Scholar
[2]Baker, A.. Rational approximations to and other algebraic numbers. Quart. J. Math. Oxford Ser. (2) 15 (1964), 375–383.CrossRefGoogle Scholar
[3]Baker, A.. Simultaneous rational approximations to certain algebraic numbers. Proc. Cambridge Philos. Soc. 63 (1967), 693–702.CrossRefGoogle Scholar
[4]Baker, A.. Transcendental Number Theory (Cambridge University Press, 1975).CrossRefGoogle Scholar
[5]Fel'dman, N. I.. An estimate of an incomplete linear form in several algebraic variables. Math. Notes 7 (1970), 343–349.CrossRefGoogle Scholar
[6]Fel'dman, N. I.. Effective bounds for the number of solutions of certain diophantine equations. Math. Notes 8 (1970), 674–679.CrossRefGoogle Scholar
[7]Osgood, C. F.. The simultaneous diophantine approximation of certain kth roots. Proc. Cambridge Philos. Soc. 67 (1970), 75–86.CrossRefGoogle Scholar
[8]Roth, K. F.. Rational approximations to algebraic numbers. Mathematika 2 (1955), 1–20CrossRefGoogle Scholar
corrigendum, Mathematika 168.Google Scholar
[9]Schmidt, W. M.Simultaneous approximation to algebraic numbers by rationals. Acta Math. 125, 189–201.CrossRefGoogle Scholar