No CrossRef data available.
Positive-moment problems in abstract measure spaces
Published online by Cambridge University Press: 24 October 2008
Extract
In this paper we continue the investigation of positive-moment problems, begun in (4). For an arbitrary index set A we consider a family (fα)α ∈ A of measurable real-valued functions on a measure-space (X,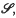 
µ). We suppose throughout that
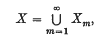
where (Xm) is an increasing sequence of measurable subsets of X and where, for each α in A and each m, fα is µ-integrable over Xm. Let (сα)α ∈ A be a given family of real numbers. We consider the following restricted positive-moment problem: does there exist a measurable function g on X such that 0 ≤° g ≤° 1 and such that
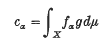
for every α in A? (Here the symbol ‘≤°’ indicates that the relation ≤ holds almost everywhere with respect to µ on X. Symbols ‘ = °, <°, …’ are used similarly.) If such a g exists we call (сα)α ∈ A a moment family for the problem:
- Type
- Research Article
- Information
- Mathematical Proceedings of the Cambridge Philosophical Society , Volume 78 , Issue 3 , November 1975 , pp. 461 - 469
- Copyright
- Copyright © Cambridge Philosophical Society 1975
References
REFERENCES
(1)Dunford, N. and Schwahtz, J. T.Linear Operators Part 1 (New York, Interscience, 1958).Google Scholar
(2)Karlin, S. and Shapley, L. S.Geometry of Moment Spaces. Mem. Amer. Math. Soc. 12 (1953).Google Scholar
(4)Rogosinski, H. P.Finite positive-moment problems. J. London. Math. Soc. 43 (1968), 658–666.CrossRefGoogle Scholar
(5)Rogosinski, W. W.Moments of non-negative mass. Proc. Royal Soc. A 245 (1958), 1–27.Google Scholar