Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-28T14:18:10.744Z Has data issue: false hasContentIssue false
  • English
  • Français

A periodicity theorem in homological algebra

Published online by Cambridge University Press:  24 October 2008

J. F. Adams
J. F. Adams
Affiliation:
Department of Mathematics, University of Manchester
Get access Rights & Permissions [Opens in a new window]

Extract

Introduction. In (1–3,6) it is shown that homological algebra can be applied to stable homotopy-theory. In this application, we deal with A -modules, where A is the mod p Steenrod algebra. To obtain a concrete geometrical result by this method usually involves work of two distinct sorts. To illustrate this, we consider the spectral sequence of (1,2):

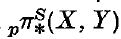Here each group Extss, t which occurs in the E2 term can be effectively computed; the process is purely algebraic. However, no such effective method is given for computing the differentials dr in the spectral sequence, or for determining the group extensions by which is built up from the E∞ term; these are topological problems.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 62 , Issue 3 , July 1966 , pp. 365 - 377
Copyright
Copyright © Cambridge Philosophical Society 1966

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Adams, J. F.On the structure and applications of the Steenrod algebra. Comment. Math. Helv. 32 (1958), 180–214.CrossRefGoogle Scholar
(2)Adams, J. F.Théorie de l'homotopie stable. Bull. Soc. Math. France, 87 (1959), 277–280.Google Scholar
(3)Adams, J. F.On the non-existence of elements of Hopf invariant one. Ann. of Math. 72 (1960), 20–104.CrossRefGoogle Scholar
(4)Adams, J. F.A finiteness theorem in homological algebra. Proc. Cambridge Philos. Soc. 57 (1961), 31–36.CrossRefGoogle Scholar
(5)Adams, J. F.Stable homotopy theory (Springer-Verlag, 1964).CrossRefGoogle Scholar
(6) Cartan Seminar Notes, 1958/1959.Google Scholar
(7)Cartan, H. and Eilenberg, S.Homological algebra (Princeton, 1956).Google Scholar
(8)Liulevicius, A.The factorisation of cyclic reduced powers by secondary cohomology operations. Mem. Amer. Math. Soc. 42 (1962).Google Scholar
(9)Liulevicius, A.Zeroes of the cohomology of the Steenrod algebra. Proc. Amer. Math. Soc. 14 (1963), 972–976.CrossRefGoogle Scholar
(10)May, J. P. Thesis (Princeton, 1964).Google Scholar
(11)Milnor, J.The Steenrod algebra and its dual. Ann. of Math. 67 (1958), 150–171.CrossRefGoogle Scholar
(12)Shimada, N. and Yamanoshita, T.On triviality of the mod p Hopf invariant. Japanese Journal of Math. 31 (1961), 1–25.Google Scholar