Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Schweigert, Christoph
and
Fuchs, Jürgen
2012.
Highlights in Lie Algebraic Methods.
Vol. 295,
Issue. ,
p.
189.
Chen, Qi
Yu, Chih-Chien
and
Zhang, Yu
2012.
Three-manifold invariants associated with restricted quantum groups.
Mathematische Zeitschrift,
Vol. 272,
Issue. 3-4,
p.
987.
Costantino, Francesco
Geer, Nathan
and
Patureau-Mirand, Bertrand
2015.
Relations between Witten–Reshetikhin–Turaev and nonsemisimple sl(2) 3–manifold invariants.
Algebraic & Geometric Topology,
Vol. 15,
Issue. 3,
p.
1363.
Chen, Qi
and
Kerler, Thomas
2017.
Integrality and gauge dependence of Hennings TQFTs.
Journal of Pure and Applied Algebra,
Vol. 221,
Issue. 11,
p.
2689.
Neumann, Daniel López
2021.
Kuperberg invariants for balanced sutured 3-manifolds.
Canadian Journal of Mathematics,
Vol. 73,
Issue. 6,
p.
1698.
López Neumann, Daniel
2022.
Twisting Kuperberg invariants via Fox calculus and Reidemeister torsion.
Algebraic & Geometric Topology,
Vol. 22,
Issue. 5,
p.
2419.
Cheong, Winston
Doser, Alexander
Gray, McKinley
and
Sawin, Stephen F.
2023.
Relationship of the Hennings and Witten–Reshetikhan–Turaev invariants for higher rank quantum groups.
Journal of Knot Theory and Its Ramifications,
Vol. 32,
Issue. 07,


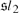 .
.