Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-26T08:45:04.382Z Has data issue: false hasContentIssue false
  • English
  • Français

On the possibility of a thermal effect accompanying sudden changes in the magneton-numbers in CuCl2 and NiSO47 H2O

Published online by Cambridge University Press:  24 October 2008

D. S. Kothari
D. S. Kothari
Affiliation:
Clare College
Get access Rights & Permissions [Opens in a new window]

Extract

1. The temperature variation of the paramagnetic susceptibility of most of the solids follows the generalised Curie law

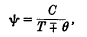
as found first by Kamerlingh Onnes and Weiss. This gives a linear relation between 1/ψ and T. The value of C is a measure of the atomic magnetic moment, and if this moment is expressed in terms of the Weiss magneton-number p, then

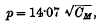
where CM is the value of C when ψ refers to a gram-molecule. The experimental results for a paramagnetic substance are usually expressed in terms of p and θ. Weiss (1), Foex (2), Cabrera and others have found that in some substances there are discontinuities in the slope of the 1/ψ, T curve. Thus Weiss (3) finds that magnetite above its Curie point shows several sudden changes in the 1/ψ, T curve, which he has interpreted as corresponding to the magneton-numbers p = 4, 5, 6, 8,10. Similarly, for cupric chloride (anhydride) Weiss (4) gives p= 9.2 and 10, for the temperature ranges — 140° to 20° and 20° to 500° respectively. Nickel sulphate (5) has also been found to possess a transition point at about — 113°, where the p value on cooling changes from 14.6 to 18.2.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 28 , Issue 3 , July 1932 , pp. 338 - 340
Copyright
Copyright © Cambridge Philosophical Society 1932

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Weiss, , Jour. de Phys., 5, p. 147 (1924).Google Scholar
(2)Foex, , Ann. d. Physique, 16, p. 247 (1921).Google Scholar
(3)Weiss, , Jour. de Phys., 1, p. 95 (1911).Google Scholar
(4)Weiss, Jour. de Phys., 5, p. 147 (1924).Google Scholar
(5)Jackson, , Phil. Trans., 224, p. 1 (1923).Google Scholar
(6)Kopp, W., “Der thermische Verlauf des Paramagnetismus”, Promotions arbeit, Zurich (1919).Google Scholar
(7)Birch, , Jour. de Phys., 9, p. 139 (1928).Google Scholar
(8)A Dictionary of Applied Physics, ii, “Potentiometer”.Google Scholar